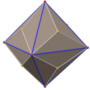
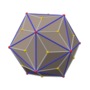
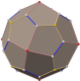
| Tetrakis hexahedron | |
|---|---|
 (Click here for rotating model) | |
| Type | Catalan solid |
| Coxeter diagram | |
| Conway notation | kC |
| Face type | V4.6.6 isosceles triangle |
| Faces | 24 |
| Edges | 36 |
| Vertices | 14 |
| Vertices by type | 6{4}+8{6} |
| Symmetry group | Oh, B3, , (*432) |
| Rotation group | O, , (432) |
| Dihedral angle | 143°07′48″ arccos(−4/5) |
| Properties | convex, face-transitive |
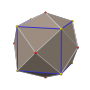
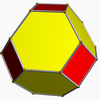 Truncated octahedron (dual polyhedron) |
 Net |

 Dual compound of truncated octahedron and tetrakis hexahedron. The woodcut on the left is from Perspectiva Corporum Regularium (1568) by Wenzel Jamnitzer.
Dual compound of truncated octahedron and tetrakis hexahedron. The woodcut on the left is from Perspectiva Corporum Regularium (1568) by Wenzel Jamnitzer.

 Die and crystal model
Die and crystal model

 Drawing and crystal model of variant with tetrahedral symmetry called hexakis tetrahedron
Drawing and crystal model of variant with tetrahedral symmetry called hexakis tetrahedron
In geometry, a tetrakis hexahedron (also known as a tetrahexahedron, hextetrahedron, tetrakis cube, and kiscube) is a Catalan solid. Its dual is the truncated octahedron, an Archimedean solid.
It can be called a disdyakis hexahedron or hexakis tetrahedron as the dual of an omnitruncated tetrahedron, and as the barycentric subdivision of a tetrahedron.
Cartesian coordinates
Cartesian coordinates for the 14 vertices of a tetrakis hexahedron centered at the origin, are the points
The length of the shorter edges of this tetrakis hexahedron equals 3/2 and that of the longer edges equals 2. The faces are acute isosceles triangles. The larger angle of these equals and the two smaller ones equal .
Orthogonal projections
The tetrakis hexahedron, dual of the truncated octahedron has 3 symmetry positions, two located on vertices and one mid-edge.
| Projective symmetry |
|||
|---|---|---|---|
| Tetrakis hexahedron |
|

|
|
| Truncated octahedron |

|

|

|
Uses
Naturally occurring (crystal) formations of tetrahexahedra are observed in copper and fluorite systems.
Polyhedral dice shaped like the tetrakis hexahedron are occasionally used by gamers.
A 24-cell viewed under a vertex-first perspective projection has a surface topology of a tetrakis hexahedron and the geometric proportions of the rhombic dodecahedron, with the rhombic faces divided into two triangles.
The tetrakis hexahedron appears as one of the simplest examples in building theory. Consider the Riemannian symmetric space associated to the group SL4(R). Its Tits boundary has the structure of a spherical building whose apartments are 2-dimensional spheres. The partition of this sphere into spherical simplices (chambers) can be obtained by taking the radial projection of a tetrakis hexahedron.
Symmetry
With Td, (*332) tetrahedral symmetry, the triangular faces represent the 24 fundamental domains of tetrahedral symmetry. This polyhedron can be constructed from 6 great circles on a sphere. It can also be seen by a cube with its square faces triangulated by their vertices and face centers and a tetrahedron with its faces divided by vertices, mid-edges, and a central point.

|

|

|
| Truncated octahedron |
Disdyakis hexahedron |
Deltoidal dodecahedron |

|

|
|
| Rhombic hexahedron |
Tetrahedron | |
| Spherical polyhedron | |||
|---|---|---|---|

|

|

|

|
| (see rotating model) | Orthographic projections from 2-, 3- and 4-fold axes | ||
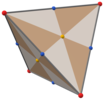
The edges of the spherical tetrakis hexahedron belong to six great circles, which correspond to mirror planes in tetrahedral symmetry. They can be grouped into three pairs of orthogonal circles (which typically intersect on one coordinate axis each). In the images below these square hosohedra are colored red, green and blue.
| Stereographic projections | |||
|---|---|---|---|

|
2-fold | 3-fold | 4-fold |

|
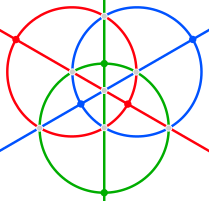
|
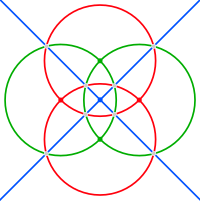
| |
Dimensions
If we denote the edge length of the base cube by a, the height of each pyramid summit above the cube is The inclination of each triangular face of the pyramid versus the cube face is (sequence A073000 in the OEIS). One edge of the isosceles triangles has length a, the other two have length which follows by applying the Pythagorean theorem to height and base length. This yields an altitude of in the triangle (OEIS: A204188). Its area is and the internal angles are and the complementary
The volume of the pyramid is so the total volume of the six pyramids and the cube in the hexahedron is
Kleetope

It can be seen as a cube with square pyramids covering each square face; that is, it is the Kleetope of the cube. A non-convex form of this shape, with equilateral triangle faces, has the same surface geometry as the regular octahedron, and a paper octahedron model can be re-folded into this shape. This form of the tetrakis hexahedron was illustrated by Leonardo da Vinci in Luca Pacioli's Divina proportione (1509).
This non-convex form of the tetrakis hexahedron can be folded along the square faces of the inner cube as a net for a four-dimensional cubic pyramid.
Related polyhedra and tilings
| Uniform octahedral polyhedra | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: , (*432) | (432) |
= (*332) |
(3*2) | |||||||
| {4,3} | t{4,3} | r{4,3} r{3} |
t{3,4} t{3} |
{3,4} {3} |
rr{4,3} s2{3,4} |
tr{4,3} | sr{4,3} | h{4,3} {3,3} |
h2{4,3} t{3,3} |
s{3,4} s{3} |
= |
= |
= |
||||||||
| Duals to uniform polyhedra | ||||||||||
| V4 | V3.8 | V(3.4) | V4.6 | V3 | V3.4 | V4.6.8 | V3.4 | V3 | V3.6 | V3 |
| *n32 symmetry mutation of truncated tilings: n.6.6 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sym. *n42 |
Spherical | Euclid. | Compact | Parac. | Noncompact hyperbolic | |||||||
| *232 |
*332 |
*432 |
*532 |
*632 |
*732 |
*832 ... |
*∞32 |
|||||
| Truncated figures |

|

|

|

|

|

|

|

|

|

|

| |
| Config. | 2.6.6 | 3.6.6 | 4.6.6 | 5.6.6 | 6.6.6 | 7.6.6 | 8.6.6 | ∞.6.6 | 12i.6.6 | 9i.6.6 | 6i.6.6 | |
| n-kis figures |

|

|

|

|

|

|

|

|
||||
| Config. | V2.6.6 | V3.6.6 | V4.6.6 | V5.6.6 | V6.6.6 | V7.6.6 | V8.6.6 | V∞.6.6 | V12i.6.6 | V9i.6.6 | V6i.6.6 | |
It is a polyhedra in a sequence defined by the face configuration V4.6.2n. This group is special for having all even number of edges per vertex and form bisecting planes through the polyhedra and infinite lines in the plane, and continuing into the hyperbolic plane for any n ≥ 7.
With an even number of faces at every vertex, these polyhedra and tilings can be shown by alternating two colors so all adjacent faces have different colors.
Each face on these domains also corresponds to the fundamental domain of a symmetry group with order 2,3,n mirrors at each triangle face vertex.
| *n32 symmetry mutation of omnitruncated tilings: 4.6.2n | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sym. *n32 |
Spherical | Euclid. | Compact hyperb. | Paraco. | Noncompact hyperbolic | |||||||
| *232 |
*332 |
*432 |
*532 |
*632 |
*732 |
*832 |
*∞32 |
|
|
|
| |
| Figures | 
|

|

|

|

|

|

|

|

|

|

| |
| Config. | 4.6.4 | 4.6.6 | 4.6.8 | 4.6.10 | 4.6.12 | 4.6.14 | 4.6.16 | 4.6.∞ | 4.6.24i | 4.6.18i | 4.6.12i | 4.6.6i |
| Duals | 
|

|

|

|

|

|

|

|

|

|

|

|
| Config. | V4.6.4 | V4.6.6 | V4.6.8 | V4.6.10 | V4.6.12 | V4.6.14 | V4.6.16 | V4.6.∞ | V4.6.24i | V4.6.18i | V4.6.12i | V4.6.6i |
See also
- Disdyakis triacontahedron
- Disdyakis dodecahedron
- Kisrhombille tiling
- Compound of three octahedra
- Deltoidal icositetrahedron, another 24-face Catalan solid.
References
- Hexakistetraeder in German, see e.g. Meyers page and Brockhaus page. The same drawing appears in Brockhaus and Efron as преломленный пирамидальный тетраэдр (refracted pyramidal tetrahedron).
- Conway, Symmetries of Things, p.284
- Langer, Joel C.; Singer, David A. (2010), "Reflections on the lemniscate of Bernoulli: the forty-eight faces of a mathematical gem", Milan Journal of Mathematics, 78 (2): 643–682, doi:10.1007/s00032-010-0124-5, MR 2781856
- Rus, Jacob (2017), "Flowsnake Earth", in Swart, David; Séquin, Carlo H.; Fenyvesi, Kristóf (eds.), Proceedings of Bridges 2017: Mathematics, Art, Music, Architecture, Education, Culture, Phoenix, Arizona: Tessellations Publishing, pp. 237–244, ISBN 978-1-938664-22-9
- Pacioli, Luca (1509), "Plates 11 and 12", Divina proportione
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. (Section 3-9)
- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, doi:10.1017/CBO9780511569371, ISBN 978-0-521-54325-5, MR 0730208 (The thirteen semiregular convex polyhedra and their duals, Page 14, Tetrakishexahedron)
- The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, page 284, Tetrakis hexahedron)
External links
- Weisstein, Eric W., "Tetrakis hexahedron" ("Catalan solid") at MathWorld.
- Virtual Reality Polyhedra www.georgehart.com: The Encyclopedia of Polyhedra
- VRML model
- Conway Notation for Polyhedra Try: "dtO" or "kC"
- Tetrakis Hexahedron – Interactive Polyhedron model
- The Uniform Polyhedra
| Convex polyhedra | |||||
|---|---|---|---|---|---|
| Platonic solids (regular) | |||||
| Archimedean solids (semiregular or uniform) | |||||
| Catalan solids (duals of Archimedean) |
| ||||
| Dihedral regular | |||||
| Dihedral uniform |
| ||||
| Dihedral others | |||||
| Degenerate polyhedra are in italics. | |||||


 and the two smaller ones equal
and the two smaller ones equal  .
.
 The inclination of each triangular face of the pyramid versus the cube face is
The inclination of each triangular face of the pyramid versus the cube face is  (sequence
(sequence  which follows by applying the
which follows by applying the  in the triangle (
in the triangle ( and the internal angles are
and the internal angles are  and the complementary
and the complementary 
 so the total volume of the six pyramids and the cube in the hexahedron is
so the total volume of the six pyramids and the cube in the hexahedron is