| Revision as of 00:03, 29 April 2007 editAziz1005 (talk | contribs)Extended confirmed users4,083 edits Undid revision 126709430 by Arash the Archer per talk page(talk)← Previous edit | Latest revision as of 22:42, 15 November 2024 edit undoMeters (talk | contribs)Extended confirmed users, New page reviewers, Pending changes reviewers, Rollbackers172,128 editsm Reverted edit by 98.20.144.215 (talk) to last version by ClueBot NGTag: Rollback | ||
| Line 1: | Line 1: | ||
| {{Short description|none}} <!-- "none" is preferred when the title is sufficiently descriptive; see ] --> | |||
| In the ], '''Islamic mathematics''' or '''Arabic mathematics''' refers to the ] developed by the ]ic civilization between ] and ]. While most scientist in this period were Muslim and ] was the dominant language, contributions were made by people of many religions (]s, ], ]s, ]) and ethnic groups (]s, ], ]).<ref></ref> The center of Islamic mathematics was located in present-day ] and ], but at its greatest extent stretched from ], ] and ] in the west, to the border of ] in the east.<ref>John J. O'Connor and Edmund F. Robertson. . ]. ]. 1999.</ref> | |||
| {{pp-move-indef|small=yes}} | |||
| ]'' by ]]] | |||
| ] during the ], especially during the 9th and 10th centuries, was built upon syntheses of ] (], ], ]) and ] (], ]). Important developments of the period include extension of the ] to include ], the systematised study of ] and advances in ] and ].<ref>{{harvp|Katz|1993}}: "A complete history of mathematics of medieval Islam cannot yet be written, since so many of these Arabic manuscripts lie unstudied... Still, the general outline... is known. In particular, Islamic mathematicians fully developed the decimal place-value number system to include decimal fractions, systematised the study of algebra and began to consider the relationship between algebra and geometry, studied and made advances on the major Greek geometrical treatises of Euclid, Archimedes, and Apollonius, and made significant improvements in plane and spherical geometry."<br/> | |||
| Islamic science and mathematics flourished under the Islamic ]ate (also known as the ] or ]) established across the ], ], ], ], the ], and in parts of ] and ] (known as ] at the time) in the 8th century. Although most Islamic texts on mathematics were written in Arabic, they were not all written by ]s, since—much like ] in ]—Arabic was used as the written language of scholars throughout the ] at the time. | |||
| ^ {{harvp|Smith|1958|loc=Vol. 1, Chapter VII.4}}: "In a general way it may be said that the Golden Age of Arabian mathematics was confined largely to the 9th and 10th centuries; that the world owes a great debt to Arab scholars for preserving and transmitting to posterity the classics of Greek mathematics; and that their work was chiefly that of transmission, although they developed considerable originality in algebra and showed some genius in their work in trigonometry."</ref> | |||
| The ] underwent significant developments in mathematics. ] played a key role in this transformation, introducing algebra as a distinct field in the 9th century. ]'s approach, departing from earlier arithmetical traditions, laid the groundwork for the arithmetization of ], influencing mathematical thought for an extended period. Successors like ] expanded on his work, contributing to advancements in various mathematical domains. The practicality and broad applicability of these mathematical methods facilitated the dissemination of Arabic mathematics to the West, contributing substantially to the evolution of Western mathematics.<ref>{{cite book |last1=Lumpkin |first1=Beatrice |url=https://archive.org/details/goldenageofmoor00vans |title=Golden age of the Moor, Volume 11 |last2=Zitler |first2=Siham |publisher=Transaction Publishers |year=1992 |isbn=1-56000-581-5 |editor-last=Van Sertima |editor-first=Ivan |editor-link=Ivan van Sertima |page= |chapter=Cairo: Science Academy of the Middle Ages}} "The Islamic mathematicians exercised a prolific influence on the development of science in Europe, enriched as much by their own discoveries as those they had inherited by the Greeks, the Indians, the Syrians, the Babylonians, etc."</ref> | |||
| Recent research paints a new picture of the debt that we owe to Islamic mathematics. Certainly many of the ideas which were previously thought to have been brilliant new conceptions due to ]an mathematicians of the 16th, 17th, and 18th centuries are now known to have been developed by Arabic/Islamic mathematicians around four centuries earlier. In many respects, the mathematics studied today is far closer in style to that of Islamic mathematics than to that of ]. | |||
| Arabic mathematical knowledge spread through various channels during the ], driven by the practical applications of ]'s methods. This dissemination was influenced not only by economic and political factors but also by cultural exchanges, exemplified by events such as the ] and the translation movement. The ], spanning from the 8th to the 14th century, marked a period of considerable advancements in various scientific disciplines, attracting scholars from medieval Europe seeking access to this knowledge. Trade routes and cultural interactions played a crucial role in introducing Arabic mathematical ideas to the West. The translation of Arabic mathematical texts, along with Greek and Roman works, during the 14th to 17th century, played a pivotal role in shaping the intellectual landscape of the ]. | |||
| ==Influences == | |||
| {{unreferenced|section|date=December 2006}} | |||
| ] and ] had an important role in the development of early Islamic mathematics, especially works such as ]'s classic ], ]'s ] and ]'s ], and it is thought that they contributed to the era of Islamic scientific innovation that lasted until the ]. Many ancient ] texts have survived only as ] translations by Islamic scholars. Perhaps the most important mathematical contribution from ] was the decimal ] ], also known as the ]. The ] historian ] (c. 1050) in his book ''Tariq al-Hind'' states that the great ] ] ] had an embassy from India and with them brought a book which was translated to Arabic as ''Sindhind''. It is assumed that ''Sindhind'' is none other than Brahmagupta's '']''. | |||
| == |
== Origin and spread of Arab-Islamic mathematics == | ||
| Arabic mathematics, particularly algebra, developed significantly during the ]. ]'s ({{Langx|ar|محمد بن موسى الخوارزمي}}; {{Circa|780|850}}) work between AD 813 and 833 in Baghdad was a turning point. He introduced the term "algebra" in the title of his book, "]," marking it as a distinct discipline. He regarded his work as "a short work on Calculation by (the rules of) Completion and Reduction, confining it to what is easiest and most useful in arithmetic".<ref name="ben Musa-2013">{{Cite book |last=ben Musa |first=Mohammed |url=http://dx.doi.org/10.1017/cbo9781139505871 |title=The Algebra of Mohammed ben Musa |date=2013-03-28 |publisher=Cambridge University Press |isbn=978-1-108-05507-9}}</ref> Later, people commented his work was not just a theoretical treatise but also practical, aimed at solving problems in areas like commerce and land measurement. | |||
| {{Mergefrom|List of Muslim mathematicians|date=April 2007}} | |||
| ===Muhammad ibn Musa al-Khwarizmi=== | |||
| {{main|Muhammad ibn Musa al-Khwarizmi}} | |||
| ]'']] | |||
| An important figure of Islamic mathematics was {{Unicode|Muḥammad ibn Mūsā al-Ḵwārizmī}} (c. 780-850), also known as al-Khwarizmi, the ] mathematician and ] of the caliph of ]. He wrote several important books and is today known for introducing the ] ] ] system, which we use today. The system was developed in ] in the ], but it was known to Europeans only in the ], from a Latin translation of al-Khwarizmi. Medieval European mathematical works used the phrase "dixit ]i" ("so says al-Khwarizmi") when they used the decimal system; from this is derived the word "]". Also the word "]" is derived from one of his works, '']'', which dealt with ]s, ]s, ], etc. – specifically it explained how to reveal unknown quantities in equations by executing balancing procedures which preserve the equality. Though some claim that his personal religion was ], it is much more likely that he was Muslim given that he was named after ], the prophet of ] and that the name ibn Musa means "son of Moses", who is an important figure in the Jewish and Muslim religions but not in Zoroastrianism. Regardless, his work has always been and remains in the mainstream of Islamic intellectual history. Al-Khwarizmi is often considered the father of algebra and algorithms for his important works in these fields. But algebra actually existed before Al-Khwarizmi, it was written about centuries before he was born by the ancient Greek mathematician Diophantus. | |||
| ]'s approach was groundbreaking in that it did not arise from any previous "arithmetical" tradition, including that of ]. He developed a new vocabulary for algebra, distinguishing between purely algebraic terms and those shared with arithmetic. Al-Khwārizmī noticed that the representation of numbers is crucial in daily life. Thus, he wanted to find or summarize a way to simplify the mathematical operation, so-called later, the algebra.<ref name="ben Musa-2013" /> His algebra was initially focused on linear and quadratic equations and the elementary arithmetic of binomials and trinomials. This approach, which involved solving equations using radicals and related algebraic calculations, influenced mathematical thinking long after his death. | |||
| Perhaps one of the most significant advances made by Islamic mathematics began with the work of al-Khwarizmi, namely the beginnings of algebra. It is important to understand just how significant this new idea was. It was a revolutionary move away from the Greek concept of mathematics which was essentially ]. | |||
| Al-Khwārizmī's proof of the rule for solving ] of the form (ax^2 + bx = c), commonly referred to as "squares plus roots equal numbers," was a monumental achievement in the history of algebra. This breakthrough laid the groundwork for the systematic approach to solving quadratic equations, which became a fundamental aspect of algebra as it developed in the Western world.<ref name="Swetz-2012">{{Cite report |url=http://dx.doi.org/10.4169/loci003901 |title=Mathematical Treasures: Mesopotamian Accounting Tokens |last=Swetz |first=Frank J. |date=2012-08-15 |publisher=The MAA Mathematical Sciences Digital Library |location=Washington, DC}}</ref> Al-Khwārizmī's method, which involved completing the square, not only provided a practical solution for equations of this type but also introduced an abstract and generalized approach to mathematical problems. His work, encapsulated in his seminal text "Al-Kitab al-Mukhtasar fi Hisab al-Jabr wal-Muqabala" (The Compendious Book on Calculation by Completion and Balancing), was translated into ] in the 12th century. This translation played a pivotal role in the transmission of algebraic knowledge to Europe, significantly influencing mathematicians during the Renaissance and shaping the evolution of modern mathematics.<ref name="Swetz-2012" /> Al-Khwārizmī's contributions, especially his proof for quadratic equations, are a testament to the rich mathematical heritage of the Islamic world and its enduring impact on Western mathematics. | |||
| Algebra was a unifying theory which allowed ]s, ]s, geometrical magnitudes, etc., to all be treated as "algebraic objects". It gave mathematics a whole new development path so much broader in concept to that which had existed before, and provided a vehicle for future development of the subject. Another important aspect of the introduction of algebraic ideas was that it allowed mathematics to be applied to itself in a way which had not happened before. | |||
| The spread of Arabic mathematics to the West was facilitated by several factors. The practicality and general applicability of al-Khwārizmī's methods were significant. They were designed to convert numerical or geometrical problems into equations in normal form, leading to canonical solution formulae. His work and that of his successors like ] laid the foundation for advances in various mathematical fields, including ], ], and ].<ref>{{Cite web |title=Extending al-Karaji's Work on Sums of Odd Powers of Integers - Introduction {{!}} Mathematical Association of America |url=https://maa.org/press/periodicals/convergence/extending-al-karajis-work-on-sums-of-odd-powers-of-integers-introduction |access-date=2023-12-15 |website=maa.org |language=en}}</ref> | |||
| Al-Khwarizmi's successors undertook a systematic application of ] to algebra, algebra to arithmetic, both to ], algebra to the ]ean theory of numbers, algebra to geometry, and geometry to algebra. This was how the creation of ], ], ], the ] of equations, the new elementary ], and the geometric construction of equations arose. | |||
| Al-Khwārizmī's algebra was an autonomous discipline with its historical perspective, eventually leading to the "arithmetization of algebra". His successors expanded on his work, adapting it to new theoretical and technical challenges and reorienting it towards a more arithmetical direction for abstract algebraic calculation. | |||
| Though Al-Khwarizmi's approach to mathematics was mostly algebraic, he did contribute to the study of practical geometry. | |||
| Arabic mathematics, epitomized by al-Khwārizmī's work, was crucial in shaping the mathematical landscape. Its spread to the West was driven by its practical applications, the expansion of mathematical concepts by his successors, and the translation and adaptation of these ideas into the Western context. This spread was a complex process involving economics, politics, and cultural exchange, greatly influencing Western mathematics. | |||
| ===Mahani=== | |||
| Persian mathematician Mahani (known as ]) (b. 820) conceived the idea of reducing geometrical problems such as duplicating the cube to problems in algebra. | |||
| The period known as the ] (8th to 14th century) was characterized by significant advancements in various fields, including ]. Scholars in the Islamic world made substantial contributions to ], ], ], and other ]. As a result, the intellectual achievements of Islamic scholars attracted the attention of scholars in medieval Europe who sought to access this wealth of knowledge. Trade routes, such as the ], facilitated the movement of goods, ideas, and knowledge between the East and West. Cities like ], ], and ] became centers of learning and attracted scholars from different cultural backgrounds.Therefore, mathematical knowledge from the Islamic world found its way to Europe through various channels. Meanwhile, the ] connected Western Europeans with the Islamic world. While the primary purpose of the Crusades was military, there was also cultural exchange and exposure to Islamic knowledge, including mathematics. European scholars who traveled to the Holy Land and other parts of the Islamic world gained access to Arabic manuscripts and mathematical treatises. During the 14th to 17th century, the translation of Arabic mathematical texts, along with ] and ] ones, played a crucial role in shaping the intellectual landscape of the Renaissance. Figures like ], who studied in North Africa and the Middle East, helped introduce and popularize Arabic numerals and mathematical concepts in Europe. | |||
| ===Al-Kindi=== | |||
| ] mathematician (also dealt with other sciences and philosophy) ] wrote many books on the subject of mathematics (geometry, harmony of numbers, measuring proportions and time, ]).<ref>http://www.muslimphilosophy.com/ma/eip/ma-k-mp.pdf</ref> He has made Hindu-Arabic numerals very popular.<ref>http://www-gap.dcs.st-and.ac.uk/%7Ehistory/HistTopics/Indian_numerals.html</ref> | |||
| == Concepts == | |||
| al-kindi | |||
| ]'s "Cubic equations and intersections of conic sections" the first page of the two-chaptered manuscript kept in Tehran University]] | |||
| === |
=== Algebra === | ||
| {{further|History of algebra}} | |||
| ] mathematician and ] ] (b. 836) made many contributions to mathematics, particularly geometry. In his work on ], he discovered an important theorem which allowed pairs of ]s to be found, that is two numbers such that each is the sum of the proper divisors of the other. Amicable numbers later played a large role in Islamic mathematics. | |||
| The study of ], the name of which is derived from the ] word meaning completion or "reunion of broken parts",<ref>{{cite web |title=algebra |work=] |url=http://www.etymonline.com/index.php?term=algebra&allowed_in_frame=0}}</ref> flourished during the ]. ], a Persian scholar in the ] in ] was the founder of algebra, is along with the ] mathematician ], known as the father of algebra. In his book '']'', Al-Khwarizmi deals with ways to solve for the ] ]s of first and second-degree (linear and quadratic) ]. He introduces the method of ], and unlike Diophantus, also gives general solutions for the equations he deals with.{{sfn|Boyer|1991|p=}}<ref>{{cite book|last=Swetz |first=Frank J. |title=Learning Activities from the History of Mathematics |url=https://books.google.com/books?id=zVYMoKhU_roC&pg=PA26 |year=1993|publisher=Walch Publishing |isbn=978-0-8251-2264-4 |page=26}}</ref><ref name=Gullberg>{{cite book |last1=Gullberg |first1=Jan |author-link=Jan Gullberg |title=Mathematics: From the Birth of Numbers |url=https://archive.org/details/mathematicsfromb1997gull |url-access=registration |date=1997 |publisher=W. W. Norton |isbn=0-393-04002-X |page=}}</ref> | |||
| Astronomy, time-keeping and ] provided other motivations for geometrical and trigonometrical research. Thabit ibn Qurra studied curves required in the construction of ]s. Thabit ibn Qurra also undertook both theoretical and observational work in astronomy. | |||
| Al-Khwarizmi's algebra was rhetorical, which means that the equations were written out in full sentences. This was unlike the algebraic work of Diophantus, which was syncopated, meaning that some symbolism is used. The transition to symbolic algebra, where only symbols are used, can be seen in the work of ] and ].<ref>{{MacTutor|id=Al-Banna|title=al-Marrakushi ibn Al-Banna}}</ref><ref name=Gullberg/> | |||
| ===Abu Kamil=== | |||
| ]ian mathematician ] (850) forms an important link in the development of algebra between al-Khwarizmi and ]. He had begun to understand what we would write in symbols as <math>\ x^n.x^m = x^{m+n}</math>. He also studied algebra using irrational numbers. | |||
| On the work done by Al-Khwarizmi, J. J. O'Connor and ] said:<ref>{{MacTutor |class=HistTopics |id=Arabic_mathematics |title=Arabic mathematics: forgotten brilliance?}}</ref> | |||
| ===Al-Battani=== | |||
| ] mathematician and astronomer ] (868-929) made accurate astronomical observations which allowed him to improve on ]'s data for the ] and the ]. He also produced a number of trigonometrical relationships: | |||
| {{quote|"Perhaps one of the most significant advances made by Arabic mathematics began at this time with the work of al-Khwarizmi, namely the beginnings of algebra. It is important to understand just how significant this new idea was. It was a revolutionary move away from the Greek concept of mathematics which was essentially geometry. Algebra was a unifying theory which allowed ], ]s, geometrical magnitudes, etc., to all be treated as "algebraic objects". It gave mathematics a whole new development path so much broader in concept to that which had existed before, and provided a vehicle for the future development of the subject. Another important aspect of the introduction of algebraic ideas was that it allowed mathematics to be applied to itself in a way which had not happened before." | ]}} | |||
| ::<math>\tan a = \frac{\sin a}{\cos a}</math> | |||
| Several other mathematicians during this time period expanded on the algebra of Al-Khwarizmi. ] wrote a book of algebra accompanied with geometrical illustrations and proofs. He also enumerated all the possible solutions to some of his problems. ], ], along with ], found several solutions of the ]. Omar Khayyam found the general geometric solution of a cubic equation.{{Citation needed|date=April 2023|reason=Wasn't Scipione del Ferro the first one?}} | |||
| ::<math>\sec a = \sqrt{1 + \tan^2 a }</math> | |||
| === Cubic equations === | |||
| He also solved the equation sin ''x'' = ''a'' cos ''x'' discovering the formula: | |||
| ] ''x''<sup>2</sup> = ''ay'', a ] with diameter ''b''/''a''<sup>2</sup>, and a vertical line through the intersection point. The solution is given by the length of the horizontal line segment from the origin to the intersection of the vertical line and the ''x''-axis.]] | |||
| {{further|Cubic equation}} | |||
| ] (c. 1038/48 in ] – 1123/24){{sfn|Struik|1987|p=96}} wrote the ''Treatise on Demonstration of Problems of Algebra'' containing the systematic solution of ], going beyond the ''Algebra'' of al-Khwārizmī.{{sfn|Boyer|1991|pp=241–242}} Khayyám obtained the solutions of these equations by finding the intersection points of two ]s. This method had been used by the Greeks,{{sfn|Struik|1987|p=97}} but they did not generalize the method to cover all equations with positive ].{{sfn|Boyer|1991|pp=241–242}} | |||
| ::<math>\sin x = \frac{a}{\sqrt{1 + a^2}}</math> | |||
| <!--needs more context | |||
| Khayyám differentiated between "geometric" and "arithmetic" solutions.{{sfn|Struik|1987|p=97}} Khayyám mistakenly believed{{sfn|Boyer|1991|pp=241–242}} arithmetic solutions only existed if the ] were ] and ].{{sfn|Struik|1987|p=97}} Khayyám did not concern himself with numerical calculations of the solutions.{{sfn|Struik|1987|p=97}} | |||
| {{#tag:ref|"Omar Khayyam (ca. 1050–1123), the "tent-maker," wrote an ''Algebra'' that went beyond that of al-Khwarizmi to include equations of third degree. Like his predecessors, Omar Khayyam provided for quadratic equations both arithmetic and geometric solutions; for general cubic equations, he believed (mistakenly, as the sixteenth century later showed), arithmetic solutions were impossible; hence he gave only geometric solutions. The scheme of using intersecting conics to solve cubics had been used earlier by Menaechmus, Archimedes, and Alhazan, but Omar Khayyam took the praiseworthy step of generalizing the method to cover all third-degree equations (having positive roots). For equations of higher degree than three, Omar Khayyam evidently did not envision similar geometric methods, for space does not contain more than three dimensions, "{{sfn|Boyer|1991|pp=241–242}}|group="note"}} | |||
| --> | |||
| ] (? in ] – 1213/4) developed a novel approach to the investigation of cubic equations—an approach which entailed finding the point at which a cubic polynomial obtains its maximum value. For example, to solve the equation <math>\ x^3 + a = b x</math>, with ''a'' and ''b'' positive, he would note that the maximum point of the curve <math>\ y = b x - x^3</math> occurs at <math>x = \textstyle\sqrt{\frac{b}{3}}</math>, and that the equation would have no solutions, one solution or two solutions, depending on whether the height of the curve at that point was less than, equal to, or greater than ''a''. His surviving works give no indication of how he discovered his formulae for the maxima of these curves. Various conjectures have been proposed to account for his discovery of them.<ref>{{cite journal |last1=Berggren |first1=J. Lennart |title=Innovation and Tradition in Sharaf al-Dīn al-Ṭūsī's ''al-Muʿādalāt'' |jstor=604533 |journal=Journal of the American Oriental Society |volume=110 |issue=2 |year=1990 |pages=304–309 |doi=10.2307/604533 |last2=Al-Tūsī |first2=Sharaf Al-Dīn |last3=Rashed |first3=Roshdi}}</ref> | |||
| and used ]'s idea of ]s ("shadows") to develop equations for calculating tangents and ]s, compiling tables of them. | |||
| {{Islamic studies sidebar}} | |||
| === |
=== Induction === | ||
| {{See also|Mathematical induction#History}} | |||
| Arab scientist ] (c. 880-943) was the son of Thabit ibn Qurra and the father of ]. He wrote the mathematical treatise ''On the elements of geometry'', a commentary on ]' ''On triangles'', and several other astronomical and political treatises. He studied medicine, the science of ], the '']'', astronomy, the theories of meteorological phenomena, logic and metaphysics. | |||
| The earliest implicit traces of mathematical induction can be found in ]'s ] (c. 300 BCE). The first explicit formulation of the principle of induction was given by ] in his {{Lang|fr|Traité du triangle arithmétique}} (1665). | |||
| ===Ibrahim ibn Sinan=== | |||
| Although Islamic mathematicians are most famed for their work on algebra, number theory and ]s, they also made considerable contributions to geometry, trigonometry and mathematical astronomy. ] (b. 908),] mathematician and the son of ] and grandson of ], introduced a method of ] more general than that of ], and was a leading figure in a revival and continuation of Greek higher geometry in the Islamic world. He studied ] and investigated the optical properties of ]s made from ]s. | |||
| In between, implicit ] by induction for ] was introduced by ] (c. 1000) and continued by ], who used it for special cases of the ] and properties of ]. | |||
| Ibrahim ibn Sinan, like his grandfather, also studied curves required in the construction of sundials, for the purposes of astronomy, time-keeping and geography, which provided motivations for geometrical and trigonometrical research. | |||
| === Irrational numbers === | |||
| ===Abu'l-Hasan al-Uqlidisi=== | |||
| The Greeks had discovered ]s, but were not happy with them and only able to cope by drawing a distinction between ''magnitude'' and ''number''. In the Greek view, magnitudes varied continuously and could be used for entities such as line segments, whereas numbers were discrete. Hence, irrationals could only be handled geometrically; and indeed Greek mathematics was mainly geometrical. Islamic mathematicians including ] and ] slowly removed the distinction between magnitude and number, allowing irrational quantities to appear as coefficients in equations and to be solutions of algebraic equations.<ref name=Sesiano/><ref>{{MacTutor|id=Al-Baghdadi|title=Abu Mansur ibn Tahir Al-Baghdadi}}</ref> They worked freely with irrationals as mathematical objects, but they did not examine closely their nature.<ref>{{cite web |last1=Allen |first1=G. Donald |date=n.d. |title=The History of Infinity |publisher=Texas A&M University |url=http://www.math.tamu.edu/~dallen/history/infinity.pdf |access-date=7 September 2016}}</ref> | |||
| The Indian methods of arithmetic with the Indo-Arabic numerals were originally used with a dust board similar to a ]. ] mathematician ] (b. 920) showed how to modify the Indian methods of arithmetic for pen and paper use. | |||
| In the twelfth century, ] translations of ]'s ] on the ] introduced the ] ] to the ].<ref name="Struik 93">{{harvnb|Struik|1987| p= 93}}</ref> His ''Compendious Book on Calculation by Completion and Balancing'' presented the first systematic solution of ] and ]s. In ] Europe, he was considered the original inventor of algebra, although it is now known that his work is based on older Indian or Greek sources.{{sfn|Rosen|1831|p=v–vi}}<ref>{{cite encyclopedia |last=Toomer |first=Gerald |author-link=Gerald Toomer |title=Al-Khwārizmī, Abu Ja'far Muḥammad ibn Mūsā |encyclopedia=] |volume=7 |editor=Gillispie, Charles Coulston |publisher=Charles Scribner's Sons |location=New York |date=1990 |isbn=0-684-16962-2 |url=http://www.encyclopedia.com/doc/1G2-2830902300.html |via=Encyclopedia.com}}</ref> He revised ]'s '']'' and wrote on astronomy and astrology. However, ] suggests that al-Khwarizmi's original work was not based on Ptolemy but on a derivative world map,{{sfn|Nallino|1939}} presumably in ] or ]. | |||
| === |
=== Spherical trigonometry === | ||
| {{further|Law of sines|History of trigonometry}} | |||
| Persian mathematician ] (940-998) invented the ] function. The Indo-Arabic system of calculating allowed the extraction of ] by Abu'l-Wáfa. | |||
| The spherical ] was discovered in the 10th century: it has been attributed variously to ], ] and ], with ] as a contributor.<ref name=Sesiano>{{cite book | |||
| Abu'l-Wáfa applied ] to astronomy and also used formulas involving ] and tangent. | |||
| | last = Sesiano | first = Jacques | |||
| | editor-last = Selin | editor-first = Helaine | editor-link = Helaine Selin | |||
| | contribution = Islamic Mathematics | |||
| | doi = 10.1007/978-94-011-4301-1_9 | |||
| | isbn = 978-94-011-4301-1 | |||
| | pages = 137–165 | |||
| | publisher = Springer Netherlands | |||
| | title = Mathematics Across Cultures: The History of Non-Western Mathematics | |||
| | year = 2000}}</ref> ]'s ''The book of unknown arcs of a sphere'' in the 11th century introduced the general law of sines.<ref name="MacTutor Al-Jayyani">{{MacTutor |id=Al-Jayyani |title=Abu Abd Allah Muhammad ibn Muadh Al-Jayyani}}</ref> The plane law of sines was described in the 13th century by ]. In his ''On the Sector Figure'', he stated the law of sines for plane and spherical triangles and provided proofs for this law.{{sfn|Berggren|2007|p=518}} | |||
| === |
=== Negative numbers === | ||
| {{further|Negative numbers}} | |||
| ] was further developed by Persian mathematician Karaji (known as ] in Arabic) (953-1029) in his treatise ''al-Fakhri'', where he extends the methodology to incorporate integral powers and integral roots of unknown quantities. | |||
| In the 9th century, Islamic mathematicians were familiar with negative numbers from the works of Indian mathematicians, but the recognition and use of negative numbers during this period remained timid.<ref name=Rashed>{{Cite book| publisher = Springer| isbn = 9780792325659| last = Rashed| first = R.| title = The Development of Arabic Mathematics: Between Arithmetic and Algebra| date =1994-06-30|pages=36–37}}</ref> ] did not use negative numbers or negative coefficients.<ref name=Rashed /> But within fifty years, ] illustrated the rules of signs for expanding the multiplication <math>(a \pm b)(c \pm d)</math>.<ref name=Ismail>{{citation | author = Mat Rofa Bin Ismail | title = Algebra in Islamic Mathematics | encyclopedia = Encyclopaedia of the History of Science, Technology, and Medicine in Non-Western Cultures | volume = 1 | year = 2008 | publisher = Springer | isbn = 9781402045592 | editor = Helaine Selin | editor-link = Helaine Selin | page=115 | edition=2nd}}</ref> ] wrote in his book ''al-Fakhrī'' that "negative quantities must be counted as terms".<ref name=Rashed /> In the 10th century, ] considered debts as negative numbers in ''A Book on What Is Necessary from the Science of Arithmetic for Scribes and Businessmen''.<ref name=Ismail /> | |||
| Karaji is seen by many as the first person to completely free algebra from geometrical operations and to replace them with the arithmetical type of operations which are at the core of algebra today. He was first to define the monomials <math>\ x, x^2, x^3, ...</math> and <math>\ 1/x, 1/x^2, 1/x^3, ...</math> and to give rules for products of any two of these. He started a school of algebra which flourished for several hundreds of years. | |||
| By the 12th century, al-Karaji's successors were to state the general rules of signs and use them to solve ]s.<ref name=Rashed /> As ] writes: | |||
| The discovery of the ] for integer ] by al-Karaji was a major factor in the development of ] based on the decimal system. | |||
| <blockquote>the product of a negative number—''al-nāqiṣ''—by a positive number—''al-zāʾid''—is negative, and by a negative number is positive. If we subtract a negative number from a higher negative number, the remainder is their negative difference. The difference remains positive if we subtract a negative number from a lower negative number. If we subtract a negative number from a positive number, the remainder is their positive sum. If we subtract a positive number from an empty power (''martaba khāliyya''), the remainder is the same negative, and if we subtract a negative number from an empty power, the remainder is the same positive number.<ref name=Rashed /></blockquote> | |||
| === |
=== Double false position === | ||
| {{further|False position method}} | |||
| ],]i mathematician (b. 965), also known as Alhazen, in his work on number theory, seems to have been the first to attempt to classify all even ]s (numbers equal to the sum of their proper divisors) as those of the form <math>\ 2^{k-1}(2^k - 1)</math> where <math>\ 2^k - 1</math> is ]. | |||
| Between the 9th and 10th centuries, the ] mathematician ] wrote a now-lost treatise on the use of double false position, known as the ''Book of the Two Errors'' (''Kitāb al-khaṭāʾayn''). The oldest surviving writing on double false position from the ] is that of ] (10th century), an ] mathematician from ], ]. He justified the technique by a formal, ]. Within the tradition of Golden Age Muslim mathematics, double false position was known as ''hisāb al-khaṭāʾayn'' ("reckoning by two errors"). It was used for centuries to solve practical problems such as commercial and juridical questions (estate partitions according to rules of ]), as well as purely recreational problems. The algorithm was often memorized with the aid of ], such as a verse attributed to ] and balance-scale diagrams explained by ] and ], who were each mathematicians of ] origin.<ref>{{Cite conference |conference=Eighth North African Meeting on the History of Arab Mathematics |last=Schwartz |first=R. K. |title=Issues in the Origin and Development of Hisab al-Khata'ayn (Calculation by Double False Position) |location=Radès, Tunisia |year=2004 |url=http://www.ub.edu/islamsci/Schwartz.pdf |access-date=2012-06-08 |archive-url=https://web.archive.org/web/20140516012137/http://www.ub.edu/islamsci/Schwartz.pdf |archive-date=2014-05-16}} {{cite web|url=http://facstaff.uindy.edu/~oaks/Biblio/COMHISMA8paper.doc |title=Issues in the Origin and Development of Hisab al-Khata'ayn (Calculation by Double False Position) |archive-url=https://web.archive.org/web/20110915195359/http://facstaff.uindy.edu/%7Eoaks/Biblio/COMHISMA8paper.doc |archive-date=2011-09-15 |url-status=dead |format=.doc}}</ref> | |||
| Al-Haytham is also the first person that we know to state ], namely that if <math>\ p</math> is prime then <math>\ 1+(p-1)!</math> is divisible by <math>\ p</math>. It is unclear whether he knew how to prove this result. It is called Wilson's theorem because of a comment made by ] in 1770 that ] had noticed the result. There is no evidence that John Wilson knew how to prove it and most certainly Waring did not. ] gave the first proof in 1771 and it should be noticed that it is more than 750 years after al-Haytham before number theory surpasses this achievement of Islamic mathematics. | |||
| == Influences == | |||
| Al-Haytham is also known as the "father of optics" for his development of modern ], which included the investigation the optical properties of mirrors made from conic sections. He was also the first to develop the modern ]. | |||
| The influence of medieval Arab-Islamic mathematics to the rest of the world is wide and profound, in both the realm of science and mathematics. The knowledge of the Arabs went into the western world through ] and ] during the translation movement. "The Moors (western Mohammedans from that part of North Africa once known as Mauritania) crossed over into Spain early in the seventh century, bringing with them the cultural resources of the Arab world".<ref name="Masters-2011a">{{Cite journal |last=Masters |first=Barry R. |date=2011-06-08 |title=Biomedical ethics, 7th edition David DeGrazia, Thomas A. Mappes, Jeffrey Brand-Ballard: 2010, Softcover, 732pp, ISBN-9780073407456 £171.15 McGraw-Hill Incorporated |url=http://dx.doi.org/10.1007/s00417-011-1640-x |journal=Graefe's Archive for Clinical and Experimental Ophthalmology |volume=250 |issue=1 |pages=159–160 |doi=10.1007/s00417-011-1640-x |issn=0721-832X}}</ref> In the 13th century, King ] established the ], in the ], where scholars translated numerous scientific and philosophical works from ] into ]. The translations included Islamic contributions to ], which helps European mathematicians and astronomers in their studies. European scholars such as ] (1114–1187) played a key role in translating and disseminating these works, thus making them accessible to a wider audience. Cremona is said to have translated into Latin "no fewer than 90 complete Arabic texts."<ref name="Masters-2011a" /> European mathematicians, building on the foundations laid by Islamic scholars, further developed practical trigonometry for applications in navigation, cartography, and celestial navigation, thus pushing forward the age of discovery and scientific revolution. The practical applications of trigonometry for navigation and astronomy became increasingly important during the Age of Exploration. | |||
| ] is one of the islamic mathematicians who made great contributions to the development of trigonometry. He "innovated new trigonometric functions, created a table of cotangents, and made some formulas in spherical trigonometry."<ref>{{Citation |title=Edited by |date=1972 |url=http://dx.doi.org/10.1016/s0049-237x(08)71546-5 |work=Contributions to Non-Standard Analysis |pages=iii |access-date=2023-12-15 |publisher=Elsevier}}</ref> These discoveries, together with his astronomical works which are praised for their accuracy, greatly advanced astronomical calculations and instruments. | |||
| ===Abu Nasr Mansur=== | |||
| ] (970-1036) was a ] ]. Abu Nasr Mansur applied spherical geometry to astronomy and also used formulas involving sine and tangent. He is well known for discovering the ]. | |||
| ] (1048–1131) was a Persian mathematician, astronomer, and poet, known for his work on algebra and geometry, particularly his investigations into the solutions of cubic equations. He was "the first in history to elaborate a geometrical theory of equations with degrees ≤ 3",<ref name="Rashed-2014">{{Cite book |last=Rashed |first=Roshdi |url=http://dx.doi.org/10.4324/9781315753867 |title=Classical Mathematics from Al-Khwarizmi to Descartes |date=2014-08-21 |publisher=Routledge |isbn=978-1-317-62239-0}}</ref> and has great influence on the work of Descartes, a French mathematician who is often regarded as the founder of analytical geometry. Indeed, "to read ]' Géométrie is to look upstream towards al-Khayyām and al-Ṭūsī; and downstream towards Newton, Leibniz, Cramer, Bézout and the Bernoulli brothers".<ref name="Rashed-2014" /> Numerous problems that appear in "La Géométrie" (Geometry) have foundations that date back to al-Khayyām. | |||
| ===Abu Sahl Kuhi (al-Kuhi)=== | |||
| Persian mathematician ] (10th century), also known as Abu Sahl al-Kuhi or just Kuhi, was a leading figure in a revival and continuation of Greek higher geometry in the Islamic world. He studied optics and investigated the optical properties of mirrors made from conic sections. He also did some important work on the ]. | |||
| ] ({{Langx|ar|أبو كامل شجاع بن أسلم بن محمد بن شجاع|links=no}}, also known as Al-ḥāsib al-miṣrī—lit. "The Egyptian Calculator") (c. 850 – c. 930), was studied algebra following the author of ''Algebra'', al-Khwārizmī. His ''Book of Algebra'' (Kitāb fī al-jabr wa al-muqābala) is "essentially a commentary on and elaboration of al-Khwārizmī's work; in part for that reason and in part for its own merit, the book enjoyed widespread popularity in the Muslim world".<ref name="Masters-2011b">{{Cite journal |last=Masters |first=Barry R. |date=2011-06-08 |title=Biomedical ethics, 7th edition David DeGrazia, Thomas A. Mappes, Jeffrey Brand-Ballard: 2010, Softcover, 732pp, ISBN-9780073407456 £171.15 McGraw-Hill Incorporated |url=http://dx.doi.org/10.1007/s00417-011-1640-x |journal=Graefe's Archive for Clinical and Experimental Ophthalmology |volume=250 |issue=1 |pages=159–160 |doi=10.1007/s00417-011-1640-x |issn=0721-832X}}</ref> It contains 69 problems, which is more than al-Khwārizmī who had 40 in his book.<ref name="Masters-2011b" /> Abū Kāmil's Algebra plays a significant role in shaping the trajectory of Western mathematics, particularly in its impact on the works of the Italian mathematician Leonardo of Pisa, widely recognized as Fibonacci. In his ''Liber Abaci'' (1202), Fibonacci extensively incorporated ideas from Arabic mathematicians, using approximately 29 problems from ''Book of Algebra'' with scarce modification.<ref name="Masters-2011b" /> | |||
| ===Biruni=== | |||
| Persian mathematician Biruni (known in Arabic as ]) (b. ]) used the sine formula in both astronomy and in the calculation of ]s and ]s of many cities. Both astronomy and geography motivated al-Biruni's extensive studies of projecting a ] onto the ]. | |||
| == Western historians' perception of the contribution of Arab mathematicians == | |||
| His contributions to ] include: | |||
| Despite the fundamental works Arabic mathematicians have done on the development of Algebra and algebraic geometry, Western historians in the 18th and early 19th century still regarded it as a fact that ] and math were unique phenomena of the West. Even though some math contributions from Arab mathematicians are occasionally acknowledged, they are considered to be "outside history or only integrated in so far as it contributed to science, which is essentially European",<ref name="Rashed-1994">{{Cite journal |last=Rashed |first=Roshdi |date=1994 |title=The Development of Arabic Mathematics: Between Arithmetic and Algebra |url=http://dx.doi.org/10.1007/978-94-017-3274-1 |journal=Boston Studies in the Philosophy of Science |doi=10.1007/978-94-017-3274-1 |issn=0068-0346}}</ref> and just some technical innovations to the ] heritage rather than open up a completely new branch of mathematics. In the French philosopher ]'s work, Arabic math is merely "a reflection of ], combined with ] and Indian influences". And according to ], "Arabic science only reproduced the teachings received from Greek science". Besides being considered as merely some insignificant additions or reflections to the great tradition of Greek classical science, math works from Arabic mathematicians are also blamed for lacking rigor and too focused on practical applications and calculations, and this is why Western historians argued they could never reach the level of Greek mathematicians.<ref name="Rashed-1994" /> As ] wrote, Arabic math "in no way superseded the level attained by Diophantus". On the other hand, they perceived that Western mathematicians went into a very different way both in its method employed and ultimate purpose, "the hallmark of Western science in its Greek origins as well as in its modern renaissance, is its conformity to rigorous standards".<ref name="Rashed-1994" /> Thus, the perceived non-rigorous proof in Arabic mathematicians' book authorizes ] to exclude the Arabic period when he retraced the evolution of algebra.<ref name="Rashed-1994" /> And instead, the history of classical algebra is written as the work of the ] and the origin of algebraic geometry is traced back to Descartes, while Arabic mathematicians' contributions are ignored deliberately. In Rashed's words: "To justify the exclusion of science written in Arabic from the history of science, one invokes its absence of rigor, its calculatory appearance and its practical aims. Furthermore, strictly dependent on Greek science and, lastly, incapable of introducing experimental norms, scientists of that time were relegated to the role of conscientious guardians of the Hellenistic museum."<ref name="Rashed-1994" /> | |||
| * theoretical and practical ] | |||
| * summation of series | |||
| * ] | |||
| * the ] | |||
| * ]s | |||
| * ] | |||
| * algebraic definitions | |||
| * method of solving algebraic equations | |||
| * ] | |||
| * ]' theorems | |||
| In 18th century ] and ], the prevailing ] view was "East and West oppose each other not as geographical but as historical positivities",<ref name="Rashed-1994" /> which labeled "]" as the essence of the West, while the "Call of the ]" movement emerged in the 19th century was interpreted as "against Rationalism"<ref name="Rashed-1994" /> and a return to a more "spiritual and harmonious" lifestyle. Thus, the prevailing ] in that period was one of the main reasons why Arabic mathematicians were often ignored for their contributions, as people outside the West were considered to be lacking the necessary rationality and scientific spirit to made significant contributions to math and science. | |||
| Some of his notable achievements included: | |||
| * At the age of seventeen, he calculated the ] of Kath, ], using the maximum altitude of the sun. | |||
| * By the age of twenty-two, he had written several short works, including a study of ], "]", which included a ] for projecting a ] on a ]. | |||
| * By the age of twenty-seven, he had written a book called "]" which referred to other work he had completed (now lost) that included one book about the ], one about the ], four about ], and two about ]. | |||
| * He attempted to refine the calculations of Eratosthenes using his methods, but his calculations were off by approximately 400 miles. | |||
| == |
== Conclusion == | ||
| The medieval Arab-Islamic world played a crucial role in shaping the trajectory of mathematics, with ]'s algebraic innovations serving as a cornerstone. The dissemination of Arabic mathematics to the West during the ], facilitated by cultural exchanges and translations, left a lasting impact on Western mathematical thought. Mathematicians like ], ], and ], with their contributions to ], ], and ], extended their influence beyond their time. Despite the foundational contributions of Arab mathematicians, Western historians in the 18th and early 19th centuries, influenced by ] views, sometimes marginalized these achievements. The East lacking rationality and scientific spirit perpetuated a biased perspective, hindering the recognition of the significant role played by Arabic mathematics in the development of algebra and other mathematical disciplines. Reevaluating the history of mathematics necessitates acknowledging the interconnectedness of diverse mathematical traditions and dispelling the notion of a uniquely European mathematical heritage. The contributions of Arab mathematicians, marked by practical applications and theoretical innovations, form an integral part of the rich tapestry of mathematical history, and deserves recognition. | |||
| Arab mathematician ] (b. 980) looked at a slight variant of Thabit ibn Qurra's theorem of amicable numbers. There were three different types of arithmetic used around this period and, by the end of the 10th century, authors such as al-Baghdadi were writing texts comparing the three ]s: Finger-reckoning arithmetic (a system derived from counting on the fingers with the numerals written entirely in words), the ] numeral system (developed by the ]), and the Indo-Arabic numerals. This third system of calculating allowed most of the advances in ]. Al-Baghdadi also contributed to improvements in the Indo-Arabic decimal system.he had quite an irrgeular choice in his verb usage. | |||
| == Other major figures == | |||
| ===Omar Khayyam=== | |||
| * ] (fl. 830) (quadratics) | |||
| The Persian poet ] (b. 1048) was also a mathematician, and wrote ''Discussions of the Difficulties in Euclid'', a book about flaws in ]. He gave a geometric solution to ]s, one of the most original discoveries in Islamic mathematics. He was also very influential in ]. He also wrote influential work on ]'s ]. | |||
| * ] (d. after 864) | |||
| * ] (826–901) | |||
| * ] (before 858 – 929) | |||
| * ] (c. 850 – c. 930) | |||
| * ] (fl. 952) (arithmetic) | |||
| * ] (d. 967) | |||
| * ] (c. 940–1000) (centres of gravity) | |||
| * ] (c. 965–1040) | |||
| * ] (973–1048) (trigonometry) | |||
| * ] (1048–1131) | |||
| * ] (c. 1116–1196) | |||
| * ] (1136–1206) | |||
| * ] (c. 1380–1429) (decimals and estimation of the circle constant) | |||
| == Gallery == | |||
| Omar Khayyam gave a complete classification of cubic equations with geometric solutions found by means of intersecting ]s. Khayyam also wrote that he hoped to give a full description of the algebraic solution of cubic equations in a later work: | |||
| <gallery> | |||
| File:Gravure originale du compas parfait par Abū Sahl al-Qūhī.jpg|Engraving of ]'s perfect compass to draw conic sections | |||
| File:Theorem of al-Haitham.JPG|The ] | |||
| </gallery> | |||
| == See also == | |||
| "''If the opportunity arises and I can succeed, I shall give all these fourteen forms with all their branches and cases, and how to distinguish whatever is possible or impossible so that a paper, containing elements which are greatly useful in this art will be prepared.''" | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | |||
| == References == | |||
| The Indo-Arabic system of calculating also allowed the extraction of roots by Omar Khayyam. Omar Khayyam also combined the use of trigonometry and ] to provide methods of solving ] by geometrical means. | |||
| {{Reflist|30em}} | |||
| == Sources ==<!--should be harvard, must be used in refs above--> | |||
| ===Al-Samawal=== | |||
| {{refbegin|2}} | |||
| ] mathematician of ],] (b. 1130) was an important member of al-Karaji's school of algebra. Al-Samawal was the first to give the new topic of algebra a precise description when he wrote that it was concerned "''with operating on unknowns using all the arithmetical tools, in the same way as the arithmetician operates on the known.''" | |||
| * {{cite book |last=Berggren |first=J. Lennart |editor=Victor J. Katz |year=2007 |title=The Mathematics of Egypt, Mesopotamia, China, India, and Islam: A Sourcebook|chapter=Mathematics in Medieval Islam|edition=2nd |publisher=] |location=Princeton, New Jersey |isbn=978-0-691-11485-9}} | |||
| * {{Citation|last=Boyer|year=1991|first=Carl B.|author-link=Carl Benjamin Boyer|title=A History of Mathematics|chapter=Greek Trigonometry and Mensuration, and The Arabic Hegemony|edition=2nd|publisher=John Wiley & Sons|location=New York City|isbn=0-471-54397-7|url=https://archive.org/details/historyofmathema00boye}} | |||
| * {{cite book |last=Katz|year=1993|first=Victor J. |title=A History of Mathematics: An Introduction|publisher=HarperCollins college publishers|isbn=0-673-38039-4}} | |||
| * {{Citation |last=Nallino |first=C.A. |author-link=C.A. Nallino |contribution=Al-Ḥuwārismī e il suo rifacimento della Geografia di Tolomeo |pages=458–532 |title=Raccolta di scritti editi e inediti |volume=V |location=Rome |publisher=Istituto per l'Oriente |year=1939 |language=it}} | |||
| * {{cite book |last=Rosen|first=Fredrick |title=The Algebra of Mohammed Ben Musa|date=1831|publisher=Kessinger Publishing |isbn=1-4179-4914-7 |url=https://archive.org/details/algebraofmohamme00khuwrich}} | |||
| * {{cite book |last=Smith|year=1958|first=David E.|author-link=David Eugene Smith|title=History of Mathematics|publisher=Dover Publications|isbn=0-486-20429-4}} | |||
| * {{Citation|last=Struik|year=1987|first=Dirk J.|author-link=Dirk Jan Struik|title=A Concise History of Mathematics|edition=4th rev.|publisher=Dover Publications|isbn=0-486-60255-9|url-access=registration|url=https://archive.org/details/concisehistoryof0000stru_m6j1}} | |||
| {{refend}} | |||
| ==Further reading== | |||
| ===Sharaf al-Din al-Tusi=== | |||
| {{Refbegin|2}} | |||
| Persian mathematician ] (b. 1135), although almost exactly the same age as al-Samawal, did not follow the general development that came through al-Karaji's school of algebra but rather followed Khayyam's application of algebra to geometry. He wrote a treatise on cubic equations, which represents an essential contribution to another algebra which aimed to study curves by means of equations, thus inaugurating the beginning of algebraic geometry. | |||
| ;Books on Islamic mathematics | |||
| ===Nasir al-Din al-Tusi=== | |||
| * {{cite book |last=Berggren |first=J. Lennart |title=Episodes in the Mathematics of Medieval Islam|year=1986|publisher=Springer-Verlag|location=New York|isbn=0-387-96318-9}} | |||
| ] was largely developed by Muslims, and systematized (along with plane trigonometry) by Persian ] mathematician ] (1201–1274). He also wrote influential work on ]'s ]. | |||
| ** Review: {{cite journal |last1=Toomer|first1=Gerald J.|author-link=Gerald J. Toomer|title=Episodes in the Mathematics of Medieval Islam |journal=] |volume=95 |issue=6 |year=1988 |doi=10.2307/2322777 |page=567 |publisher=Mathematical Association of America|last2=Berggren|first2=J. L.|jstor=2322777}} | |||
| ** Review: {{cite journal |last1=Hogendijk |first1=Jan P.| title=''Episodes in the Mathematics of Medieval Islam'' by J. Lennart Berggren |journal=Journal of the American Oriental Society |volume=109 |issue=4 |year=1989 |pages=697–698 |doi=10.2307/604119 |publisher=American Oriental Society|last2=Berggren|first2=J. L. |jstor=604119}} | |||
| * {{cite book |last=Daffa' |first=Ali Abdullah al- |author-link=Ali Abdullah Al-Daffa |title=The Muslim contribution to mathematics |year=1977 |publisher=Croom Helm |location=London |isbn=0-85664-464-1}} | |||
| * {{cite book |last=Ronan|year=1983|first=Colin A.|author-link=Colin Ronan|title=The Cambridge Illustrated History of the World's Science|publisher=Cambridge University Press|isbn=0-521-25844-8}} | |||
| * {{cite book |last=Rashed|first=Roshdi |author-link=Roshdi Rashed |others=Translated by A. F. W. Armstrong |title=The Development of Arabic Mathematics: Between Arithmetic and Algebra |publisher=Springer|year=2001 |isbn=0-7923-2565-6}} | |||
| * {{cite book |last=Youschkevitch |first=Adolf P. |author-link=Adolph Pavlovich Yushkevich |author2=Rozenfeld, Boris A. |title=Die Mathematik der Länder des Ostens im Mittelalter |year=1960|location=Berlin}} Sowjetische Beiträge zur Geschichte der Naturwissenschaft pp. 62–160. | |||
| * {{cite book |last=Youschkevitch |first=Adolf P. |title=Les mathématiques arabes: VIII<sup>e</sup>–XV<sup>e</sup> siècles |others=translated by M. Cazenave and K. Jaouiche |publisher=Vrin |location=Paris |year=1976 |isbn=978-2-7116-0734-1}} | |||
| ; Book chapters on Islamic mathematics | |||
| Nasir al-Din al-Tusi, like many other Muslim mathematicians, based his theoretical astronomy on Ptolemy's work, but al-Tusi made the most significant development in the ] until the development of the ]. One of these developments is the ], which was later used in the Copernican model. | |||
| * Lindberg, D.C., and M. H. Shank, eds. ''The Cambridge History of Science. Volume 2: Medieval Science'' (Cambridge UP, 2013), chapters 2 and 3 mathematics in Islam. | |||
| * {{cite book | first=Roger | last=Cooke | author-link=Roger Cooke (mathematician) | title=The History of Mathematics: A Brief Course | chapter=Islamic Mathematics | publisher=Wiley-Interscience | year=1997 | isbn=0-471-18082-3 | url=https://archive.org/details/historyofmathema0000cook }} | |||
| ===Ibn Al-Banna=== | |||
| ] mathematician ] of ](b. 1256) used symbols in algebra, though symbols were used by other Islamic mathematicians at least a century before this. | |||
| ; Books on Islamic science | |||
| ===Al-Farisi=== | |||
| * {{cite book|first1=Ali Abdullah al-|last1=Daffa|first2=J.J.|last2=Stroyls|title=Studies in the exact sciences in medieval Islam|publisher=Wiley|location=New York|year=1984|isbn=0-471-90320-5}} | |||
| Persian mathematician ] (b. 1260) gave a new proof of Thabit ibn Qurra's theorem of amicable numbers, introducing important new ideas concerning ] and combinatorial methods. He also gave the pair of amicable numbers 17,296 and 18,416 which have been attributed to ], but we know that these were known earlier than al-Farisi, perhaps even by Thabit ibn Qurra himself. Apart from number theory, his other major contribution to mathematics was on ]. | |||
| * {{cite book|first=E. S.|last=Kennedy|author-link=Edward Stewart Kennedy|title=Studies in the Islamic Exact Sciences|year=1984|publisher=Syracuse Univ Press|isbn=0-8156-6067-7}} | |||
| ; Books on the history of mathematics | |||
| ===Ghiyath al-Kashi=== | |||
| * {{cite book|last=Joseph|first=George Gheverghese|title=The Crest of the Peacock: Non-European Roots of Mathematics|edition=2nd|publisher=Princeton University Press|year=2000|isbn=0-691-00659-8|url=https://archive.org/details/crestofpeacockno00jose}} (Reviewed: {{cite journal|first1=Victor J.|last1=Katz|title='']'' by George Gheverghese Joseph|journal=The College Mathematics Journal|volume=23|issue=1|year=1992|pages=82–84|doi=10.2307/2686206|publisher=Mathematical Association of America|last2=Joseph|first2=George Gheverghese|jstor=2686206}}) | |||
| Persian mathematician ] (1380-1429) contributed to the development of ]s not only for approximating ]s, but also for ]s such as ], which he computed to 16 decimal place of accuracy. His contribution to decimal fractions is so major that for many years he was considered as their inventor. Kashi also developed an algorithm for calculating ], which was a special case of the methods given many centuries later by ] and ]. | |||
| * {{cite book|last=Youschkevitch|first=Adolf P.|title=Gesichte der Mathematik im Mittelalter|publisher=BG Teubner Verlagsgesellschaft|location=Leipzig|year=1964}} | |||
| ;Journal articles on Islamic mathematics | |||
| Al-Kashi also produced tables of trigonometric functions as part of his studies of astronomy. His sine tables were correct to 4 ] digits, which corresponds to approximately 8 decimal places of accuracy. The construction of astronomical instruments such as the ], invented by ], was also a speciality of Muslim mathematicians. | |||
| * Høyrup, Jens. . ''Filosofi og Videnskabsteori på Roskilde Universitetscenter''. 3. Række: ''Preprints og Reprints'' 1987 Nr. 1. | |||
| ;Bibliographies and biographies | |||
| ===Ulugh Beg=== | |||
| * ]. ''Geschichte der Arabischen Litteratur''. 1.–2. Band, 1.–3. Supplementband. Berlin: Emil Fischer, 1898, 1902; Leiden: Brill, 1937, 1938, 1942. | |||
| ] mathematician ] (1393 or 1394 – 1449), also ruler of the ], produced tables of trigonometric functions as part of his studies of astronomy. His sine and tangent tables were correct to 8 decimal places of accuracy. | |||
| * {{cite book|last=Sánchez Pérez|first=José A.|author-link=José Augusto Sánchez Pérez|title=Biografías de Matemáticos Árabes que florecieron en España|location=Madrid|publisher=Estanislao Maestre|year=1921}} | |||
| * {{cite book|last=Sezgin|first=Fuat|author-link=Fuat Sezgin|title=Geschichte Des Arabischen Schrifttums|publisher=Brill Academic Publishers|language=de|year=1997|isbn=90-04-02007-1}} | |||
| * {{cite book|last=Suter|first=Heinrich|author-link=Heinrich Suter|title=Die Mathematiker und Astronomen der Araber und ihre Werke|series=Abhandlungen zur Geschichte der Mathematischen Wissenschaften Mit Einschluss Ihrer Anwendungen, X Heft|location=Leipzig|year=1900}} | |||
| ; Television documentaries | |||
| ===Al-Qalasadi=== | |||
| * ] (presenter) (2008). "The Genius of the East". '']''. ]. | |||
| ]-] mathematician ] (b. 1412) used symbols in algebra, though symbols were used by other Islamic mathematicians much earlier. | |||
| * ] (presenter) (2010). '']''. ]. | |||
| {{Refend}} | |||
| ==External links== | |||
| In the time of the ] (from ] onwards) the development of Islamic mathematics became stagnant. This parallels the stagnation of mathematics when the Romans conquerored the Hellenistic world. | |||
| {{Commonscat|Mathematics of the Islamic Golden Age}} | |||
| *{{cite web|last=Hogendijk|first=Jan P.|author-link=Jan Hogendijk|date=January 1999|url=http://www.jphogendijk.nl/publ/Islamath.html|title=Bibliography of Mathematics in Medieval Islamic Civilization}} | |||
| *{{MacTutor|class=HistTopics|id=Arabic_mathematics|title=Arabic mathematics: forgotten brilliance?|year=1999}} | |||
| * | |||
| * | |||
| {{History of mathematics}} | |||
| {{Islamic mathematics}} | |||
| {{Islamic studies}} | |||
| {{DEFAULTSORT:Mathematics In Medieval Islam}} | |||
| ===Muhammad Baqir Yazdi=== | |||
| ] | |||
| In the 17th century, Persian mathematician ] gave the pair of ] 9,363,584 and 9,437,056 many years before Euler's contribution to amicable numbers. | |||
| ] | |||
| ==Translations== | |||
| Many ] texts on Islamic mathematics were translated into ] and had an important role in the evolution of later European mathematics. A list of translations, from ] and ] to Arabic, and from Arabic to Latin, is given below. | |||
| ===Greek to Arabic=== | |||
| The following mathematical ] texts on Hellenistic mathematics were translated into Arabic, and subsequently into Latin: | |||
| *]'s ''Data'', ''Optics'', ''Phaenomena'' and ''On Divisions''. | |||
| *Euclid's '']'' by ] (c. 8th century) | |||
| *Revision of Euclid's ''Elements'' by Thabit ibn Qurra. | |||
| *]' ''Conics'' by Thabit ibn Qurra. | |||
| *]'s '']'' by Thabit ibn Qurra. | |||
| *]' ''Sphere and Cylinder'' and ''Measurement of the Circle'' by Thabit ibn Qurra. | |||
| *Archimedes' ''On triangles'' by Sinan ibn Thabit. | |||
| *]' '']'' by Abu'l-Wáfa. | |||
| *]'s ''Sphaerica''. | |||
| *]'s ''Spherics''. | |||
| *]' treatise on mirrors. | |||
| *]'s work on mechanics. | |||
| ===Sanskrit to Arabic=== | |||
| The following mathematical ] texts on Indian mathematics were translated into Arabic, and subsequently into Latin: | |||
| *''The ]'' by ], ] and ] (c. 8th century). | |||
| *'']'' by al-Fazari. | |||
| *]'s '']'' by al-Fazari. | |||
| *Brahmagupta's ''Khandakhayaka''. | |||
| *]'s ''Aryabhatiya''. | |||
| *Aryabhata's ''Arya Siddhanta''. | |||
| *]'s ''Pancha Siddhanta''. | |||
| *]'s ''Lagu Bhaskariya''. | |||
| *]'s ''Lilavati'' (to ] rather than Arabic). | |||
| ===Arabic to Latin=== | |||
| The following mathematical Arabic texts on Islamic mathematics were translated into ]: | |||
| *''Introduction to Astronomy'' by ] (fl. 1116-1142). | |||
| *]'s arithmetical work ''Liber ysagogarum Alchorismi'' and ''Astronomical Tables'' by Adelard of Bath. | |||
| *Al-Khwarizmi's trigonometrical tables which deal with the sine and tangent by Adelard of Bath (1126). | |||
| *Al-Khwarizmi's ''Zij al-Sindhind'' in ] (1126). | |||
| *''Liber alghoarismi de practica arismetrice'', an ellaboration of al-Khwarizmi's ''Arithmetic'', by ] and ] (fl. 1135-1153). | |||
| *'']'' by John of Seville and Domingo Gundisalvo. | |||
| *''De differentia spiritus et animae'' of ] (Costa Ben Luca in Latin) by John of Seville and Domingo Gundisalvo. | |||
| *]'s ''De motu stellarum'', which contains important material on trigonometry, by ] (fl. 1134-1145). | |||
| *]'s ''Liber embadorum'' by Plato of Tivoli. | |||
| *''Liber de compositione alchimiae'' (''The Book of the Composition of Alchemy'') by ] (f. 1141-1150). | |||
| *Al-Khwarizmi's '']'' (''Algebra''), ''Kitab al-Adad al-Hindi'' ('']''), and revised astronomical tables by Robert of Chester. | |||
| *Al-Khwarizmi's ''Kitab-ul Jama wat Tafriq'' by Bon Compagni (1157). | |||
| *Al-Khwarizmi's ''Algebra'' by ] (fl. 1150-1185). | |||
| *]'s ''Elementa astronomica'' by Gerard of Cremona. | |||
| *The ]'s (], ] and ]) works on geometry by Gerard of Cremona. | |||
| *]'s commentary on Euclid's ''Elements'' by Gerard of Cremona. | |||
| *Muhammad ibn Muhammad Baqi's commentary on Euclid's ''Elements'' by Gerard of Cremona. | |||
| *Abul Abbas Nairizi's commentaries on Euclid and Ptolemy by Gerard of Cremona. | |||
| *The works of ] by Gerard of Cremona. | |||
| *]'s ''Algebra''. | |||
| *]'s ''Tariq Al Hind''. | |||
| *]'s ''The Sindhind''. | |||
| *The works of ] (Avicenna in Latin) | |||
| *The works of ] (Alhazen in Latin) | |||
| *The works of ] (Averroes in Latin) | |||
| *The works of ]. | |||
| *The works of Mu'ayyad al-Din al-'Urdi (c. 1250). | |||
| *The works of ] (1304–1375). | |||
| *The works of ] (to ] and then Latin). | |||
| *The works of ] (Arzachel in Latin). | |||
| ==See also== | |||
| *] | |||
| ==References== | |||
| <references/> | |||
| * Berggren, J. L. ''Episodes in the Mathematics of Medieval Islam''. Springer-Verlag: 1986. | |||
| * Berggren, J. L. ''Mathematics in medieval Islam''. ]. | |||
| * Burton, David M. ''The History of Mathematics: An Introduction''. McGraw Hill: 1997. | |||
| * Joseph, George Gheverghese. ''The Crest of the Peacock: Non-European Roots of Mathematics'', 2nd Edition. ]: 2000. | |||
| * Katz, Victor J. ''A History of Mathematics: An Introduction'', 2nd Edition. ]: 1998. | |||
| * Rashed, Roshdi. ''The Development of Arabic Mathematics: Between Arithmetic and Algebra''. Transl. by A. F. W. Armstrong. Kluwer Academic Publishers: 1994. | |||
| * John J. O'Connor and Edmund F. Robertson. . ]. ]. 1999. | |||
| * John J. O'Connor and Edmund F. Robertson. . MacTutor History of Mathematics archive. St Andrews University. 2004. | |||
| * George Saliba, , ], 1999. | |||
| * | |||
| *http://www.usc.edu/dept/MSA/introduction/woi_knowledge.html#28 | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
Latest revision as of 22:42, 15 November 2024

Mathematics during the Golden Age of Islam, especially during the 9th and 10th centuries, was built upon syntheses of Greek mathematics (Euclid, Archimedes, Apollonius) and Indian mathematics (Aryabhata, Brahmagupta). Important developments of the period include extension of the place-value system to include decimal fractions, the systematised study of algebra and advances in geometry and trigonometry.
The medieval Islamic world underwent significant developments in mathematics. Muhammad ibn Musa al-Khwārizmī played a key role in this transformation, introducing algebra as a distinct field in the 9th century. Al-Khwārizmī's approach, departing from earlier arithmetical traditions, laid the groundwork for the arithmetization of algebra, influencing mathematical thought for an extended period. Successors like Al-Karaji expanded on his work, contributing to advancements in various mathematical domains. The practicality and broad applicability of these mathematical methods facilitated the dissemination of Arabic mathematics to the West, contributing substantially to the evolution of Western mathematics.
Arabic mathematical knowledge spread through various channels during the medieval era, driven by the practical applications of Al-Khwārizmī's methods. This dissemination was influenced not only by economic and political factors but also by cultural exchanges, exemplified by events such as the Crusades and the translation movement. The Islamic Golden Age, spanning from the 8th to the 14th century, marked a period of considerable advancements in various scientific disciplines, attracting scholars from medieval Europe seeking access to this knowledge. Trade routes and cultural interactions played a crucial role in introducing Arabic mathematical ideas to the West. The translation of Arabic mathematical texts, along with Greek and Roman works, during the 14th to 17th century, played a pivotal role in shaping the intellectual landscape of the Renaissance.
Origin and spread of Arab-Islamic mathematics
Arabic mathematics, particularly algebra, developed significantly during the medieval period. Muhammad ibn Musa al-Khwārizmī's (Arabic: محمد بن موسى الخوارزمي; c. 780 – c. 850) work between AD 813 and 833 in Baghdad was a turning point. He introduced the term "algebra" in the title of his book, "Kitab al-jabr wa al-muqabala," marking it as a distinct discipline. He regarded his work as "a short work on Calculation by (the rules of) Completion and Reduction, confining it to what is easiest and most useful in arithmetic". Later, people commented his work was not just a theoretical treatise but also practical, aimed at solving problems in areas like commerce and land measurement.
Al-Khwārizmī's approach was groundbreaking in that it did not arise from any previous "arithmetical" tradition, including that of Diophantus. He developed a new vocabulary for algebra, distinguishing between purely algebraic terms and those shared with arithmetic. Al-Khwārizmī noticed that the representation of numbers is crucial in daily life. Thus, he wanted to find or summarize a way to simplify the mathematical operation, so-called later, the algebra. His algebra was initially focused on linear and quadratic equations and the elementary arithmetic of binomials and trinomials. This approach, which involved solving equations using radicals and related algebraic calculations, influenced mathematical thinking long after his death.
Al-Khwārizmī's proof of the rule for solving quadratic equations of the form (ax^2 + bx = c), commonly referred to as "squares plus roots equal numbers," was a monumental achievement in the history of algebra. This breakthrough laid the groundwork for the systematic approach to solving quadratic equations, which became a fundamental aspect of algebra as it developed in the Western world. Al-Khwārizmī's method, which involved completing the square, not only provided a practical solution for equations of this type but also introduced an abstract and generalized approach to mathematical problems. His work, encapsulated in his seminal text "Al-Kitab al-Mukhtasar fi Hisab al-Jabr wal-Muqabala" (The Compendious Book on Calculation by Completion and Balancing), was translated into Latin in the 12th century. This translation played a pivotal role in the transmission of algebraic knowledge to Europe, significantly influencing mathematicians during the Renaissance and shaping the evolution of modern mathematics. Al-Khwārizmī's contributions, especially his proof for quadratic equations, are a testament to the rich mathematical heritage of the Islamic world and its enduring impact on Western mathematics.
The spread of Arabic mathematics to the West was facilitated by several factors. The practicality and general applicability of al-Khwārizmī's methods were significant. They were designed to convert numerical or geometrical problems into equations in normal form, leading to canonical solution formulae. His work and that of his successors like al-Karaji laid the foundation for advances in various mathematical fields, including number theory, numerical analysis, and rational Diophantine analysis.
Al-Khwārizmī's algebra was an autonomous discipline with its historical perspective, eventually leading to the "arithmetization of algebra". His successors expanded on his work, adapting it to new theoretical and technical challenges and reorienting it towards a more arithmetical direction for abstract algebraic calculation.
Arabic mathematics, epitomized by al-Khwārizmī's work, was crucial in shaping the mathematical landscape. Its spread to the West was driven by its practical applications, the expansion of mathematical concepts by his successors, and the translation and adaptation of these ideas into the Western context. This spread was a complex process involving economics, politics, and cultural exchange, greatly influencing Western mathematics.
The period known as the Islamic Golden Age (8th to 14th century) was characterized by significant advancements in various fields, including mathematics. Scholars in the Islamic world made substantial contributions to mathematics, astronomy, medicine, and other sciences. As a result, the intellectual achievements of Islamic scholars attracted the attention of scholars in medieval Europe who sought to access this wealth of knowledge. Trade routes, such as the Silk Road, facilitated the movement of goods, ideas, and knowledge between the East and West. Cities like Baghdad, Cairo, and Cordoba became centers of learning and attracted scholars from different cultural backgrounds.Therefore, mathematical knowledge from the Islamic world found its way to Europe through various channels. Meanwhile, the Crusades connected Western Europeans with the Islamic world. While the primary purpose of the Crusades was military, there was also cultural exchange and exposure to Islamic knowledge, including mathematics. European scholars who traveled to the Holy Land and other parts of the Islamic world gained access to Arabic manuscripts and mathematical treatises. During the 14th to 17th century, the translation of Arabic mathematical texts, along with Greek and Roman ones, played a crucial role in shaping the intellectual landscape of the Renaissance. Figures like Fibonacci, who studied in North Africa and the Middle East, helped introduce and popularize Arabic numerals and mathematical concepts in Europe.
Concepts

Algebra
Further information: History of algebraThe study of algebra, the name of which is derived from the Arabic word meaning completion or "reunion of broken parts", flourished during the Islamic golden age. Muhammad ibn Musa al-Khwarizmi, a Persian scholar in the House of Wisdom in Baghdad was the founder of algebra, is along with the Greek mathematician Diophantus, known as the father of algebra. In his book The Compendious Book on Calculation by Completion and Balancing, Al-Khwarizmi deals with ways to solve for the positive roots of first and second-degree (linear and quadratic) polynomial equations. He introduces the method of reduction, and unlike Diophantus, also gives general solutions for the equations he deals with.
Al-Khwarizmi's algebra was rhetorical, which means that the equations were written out in full sentences. This was unlike the algebraic work of Diophantus, which was syncopated, meaning that some symbolism is used. The transition to symbolic algebra, where only symbols are used, can be seen in the work of Ibn al-Banna' al-Marrakushi and Abū al-Ḥasan ibn ʿAlī al-Qalaṣādī.
On the work done by Al-Khwarizmi, J. J. O'Connor and Edmund F. Robertson said:
"Perhaps one of the most significant advances made by Arabic mathematics began at this time with the work of al-Khwarizmi, namely the beginnings of algebra. It is important to understand just how significant this new idea was. It was a revolutionary move away from the Greek concept of mathematics which was essentially geometry. Algebra was a unifying theory which allowed rational numbers, irrational numbers, geometrical magnitudes, etc., to all be treated as "algebraic objects". It gave mathematics a whole new development path so much broader in concept to that which had existed before, and provided a vehicle for the future development of the subject. Another important aspect of the introduction of algebraic ideas was that it allowed mathematics to be applied to itself in a way which had not happened before."
— MacTutor History of Mathematics archive
Several other mathematicians during this time period expanded on the algebra of Al-Khwarizmi. Abu Kamil Shuja' wrote a book of algebra accompanied with geometrical illustrations and proofs. He also enumerated all the possible solutions to some of his problems. Abu al-Jud, Omar Khayyam, along with Sharaf al-Dīn al-Tūsī, found several solutions of the cubic equation. Omar Khayyam found the general geometric solution of a cubic equation.
Cubic equations
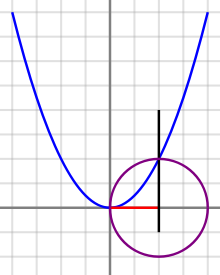
Omar Khayyam (c. 1038/48 in Iran – 1123/24) wrote the Treatise on Demonstration of Problems of Algebra containing the systematic solution of cubic or third-order equations, going beyond the Algebra of al-Khwārizmī. Khayyám obtained the solutions of these equations by finding the intersection points of two conic sections. This method had been used by the Greeks, but they did not generalize the method to cover all equations with positive roots.
Sharaf al-Dīn al-Ṭūsī (? in Tus, Iran – 1213/4) developed a novel approach to the investigation of cubic equations—an approach which entailed finding the point at which a cubic polynomial obtains its maximum value. For example, to solve the equation , with a and b positive, he would note that the maximum point of the curve occurs at , and that the equation would have no solutions, one solution or two solutions, depending on whether the height of the curve at that point was less than, equal to, or greater than a. His surviving works give no indication of how he discovered his formulae for the maxima of these curves. Various conjectures have been proposed to account for his discovery of them.
| Part of a series on |
| Islamic studies |
|---|
| Jurisprudence |
| Science in medieval times |
| Arts |
| Other topics |
| Islamization of knowledge |
Induction
See also: Mathematical induction § HistoryThe earliest implicit traces of mathematical induction can be found in Euclid's proof that the number of primes is infinite (c. 300 BCE). The first explicit formulation of the principle of induction was given by Pascal in his Traité du triangle arithmétique (1665).
In between, implicit proof by induction for arithmetic sequences was introduced by al-Karaji (c. 1000) and continued by al-Samaw'al, who used it for special cases of the binomial theorem and properties of Pascal's triangle.
Irrational numbers
The Greeks had discovered irrational numbers, but were not happy with them and only able to cope by drawing a distinction between magnitude and number. In the Greek view, magnitudes varied continuously and could be used for entities such as line segments, whereas numbers were discrete. Hence, irrationals could only be handled geometrically; and indeed Greek mathematics was mainly geometrical. Islamic mathematicians including Abū Kāmil Shujāʿ ibn Aslam and Ibn Tahir al-Baghdadi slowly removed the distinction between magnitude and number, allowing irrational quantities to appear as coefficients in equations and to be solutions of algebraic equations. They worked freely with irrationals as mathematical objects, but they did not examine closely their nature.
In the twelfth century, Latin translations of Al-Khwarizmi's Arithmetic on the Indian numerals introduced the decimal positional number system to the Western world. His Compendious Book on Calculation by Completion and Balancing presented the first systematic solution of linear and quadratic equations. In Renaissance Europe, he was considered the original inventor of algebra, although it is now known that his work is based on older Indian or Greek sources. He revised Ptolemy's Geography and wrote on astronomy and astrology. However, C.A. Nallino suggests that al-Khwarizmi's original work was not based on Ptolemy but on a derivative world map, presumably in Syriac or Arabic.
Spherical trigonometry
Further information: Law of sines and History of trigonometryThe spherical law of sines was discovered in the 10th century: it has been attributed variously to Abu-Mahmud Khojandi, Nasir al-Din al-Tusi and Abu Nasr Mansur, with Abu al-Wafa' Buzjani as a contributor. Ibn Muʿādh al-Jayyānī's The book of unknown arcs of a sphere in the 11th century introduced the general law of sines. The plane law of sines was described in the 13th century by Nasīr al-Dīn al-Tūsī. In his On the Sector Figure, he stated the law of sines for plane and spherical triangles and provided proofs for this law.
Negative numbers
Further information: Negative numbersIn the 9th century, Islamic mathematicians were familiar with negative numbers from the works of Indian mathematicians, but the recognition and use of negative numbers during this period remained timid. Al-Khwarizmi did not use negative numbers or negative coefficients. But within fifty years, Abu Kamil illustrated the rules of signs for expanding the multiplication . Al-Karaji wrote in his book al-Fakhrī that "negative quantities must be counted as terms". In the 10th century, Abū al-Wafā' al-Būzjānī considered debts as negative numbers in A Book on What Is Necessary from the Science of Arithmetic for Scribes and Businessmen.
By the 12th century, al-Karaji's successors were to state the general rules of signs and use them to solve polynomial divisions. As al-Samaw'al writes:
the product of a negative number—al-nāqiṣ—by a positive number—al-zāʾid—is negative, and by a negative number is positive. If we subtract a negative number from a higher negative number, the remainder is their negative difference. The difference remains positive if we subtract a negative number from a lower negative number. If we subtract a negative number from a positive number, the remainder is their positive sum. If we subtract a positive number from an empty power (martaba khāliyya), the remainder is the same negative, and if we subtract a negative number from an empty power, the remainder is the same positive number.
Double false position
Further information: False position methodBetween the 9th and 10th centuries, the Egyptian mathematician Abu Kamil wrote a now-lost treatise on the use of double false position, known as the Book of the Two Errors (Kitāb al-khaṭāʾayn). The oldest surviving writing on double false position from the Middle East is that of Qusta ibn Luqa (10th century), an Arab mathematician from Baalbek, Lebanon. He justified the technique by a formal, Euclidean-style geometric proof. Within the tradition of Golden Age Muslim mathematics, double false position was known as hisāb al-khaṭāʾayn ("reckoning by two errors"). It was used for centuries to solve practical problems such as commercial and juridical questions (estate partitions according to rules of Quranic inheritance), as well as purely recreational problems. The algorithm was often memorized with the aid of mnemonics, such as a verse attributed to Ibn al-Yasamin and balance-scale diagrams explained by al-Hassar and Ibn al-Banna, who were each mathematicians of Moroccan origin.
Influences
The influence of medieval Arab-Islamic mathematics to the rest of the world is wide and profound, in both the realm of science and mathematics. The knowledge of the Arabs went into the western world through Spain and Sicily during the translation movement. "The Moors (western Mohammedans from that part of North Africa once known as Mauritania) crossed over into Spain early in the seventh century, bringing with them the cultural resources of the Arab world". In the 13th century, King Alfonso X of Castile established the Toledo School of Translators, in the Kingdom of Castile, where scholars translated numerous scientific and philosophical works from Arabic into Latin. The translations included Islamic contributions to trigonometry, which helps European mathematicians and astronomers in their studies. European scholars such as Gerard of Cremona (1114–1187) played a key role in translating and disseminating these works, thus making them accessible to a wider audience. Cremona is said to have translated into Latin "no fewer than 90 complete Arabic texts." European mathematicians, building on the foundations laid by Islamic scholars, further developed practical trigonometry for applications in navigation, cartography, and celestial navigation, thus pushing forward the age of discovery and scientific revolution. The practical applications of trigonometry for navigation and astronomy became increasingly important during the Age of Exploration.
Al-Battānī is one of the islamic mathematicians who made great contributions to the development of trigonometry. He "innovated new trigonometric functions, created a table of cotangents, and made some formulas in spherical trigonometry." These discoveries, together with his astronomical works which are praised for their accuracy, greatly advanced astronomical calculations and instruments.
Al-Khayyām (1048–1131) was a Persian mathematician, astronomer, and poet, known for his work on algebra and geometry, particularly his investigations into the solutions of cubic equations. He was "the first in history to elaborate a geometrical theory of equations with degrees ≤ 3", and has great influence on the work of Descartes, a French mathematician who is often regarded as the founder of analytical geometry. Indeed, "to read Descartes' Géométrie is to look upstream towards al-Khayyām and al-Ṭūsī; and downstream towards Newton, Leibniz, Cramer, Bézout and the Bernoulli brothers". Numerous problems that appear in "La Géométrie" (Geometry) have foundations that date back to al-Khayyām.
Abū Kāmil (Arabic: أبو كامل شجاع بن أسلم بن محمد بن شجاع, also known as Al-ḥāsib al-miṣrī—lit. "The Egyptian Calculator") (c. 850 – c. 930), was studied algebra following the author of Algebra, al-Khwārizmī. His Book of Algebra (Kitāb fī al-jabr wa al-muqābala) is "essentially a commentary on and elaboration of al-Khwārizmī's work; in part for that reason and in part for its own merit, the book enjoyed widespread popularity in the Muslim world". It contains 69 problems, which is more than al-Khwārizmī who had 40 in his book. Abū Kāmil's Algebra plays a significant role in shaping the trajectory of Western mathematics, particularly in its impact on the works of the Italian mathematician Leonardo of Pisa, widely recognized as Fibonacci. In his Liber Abaci (1202), Fibonacci extensively incorporated ideas from Arabic mathematicians, using approximately 29 problems from Book of Algebra with scarce modification.
Western historians' perception of the contribution of Arab mathematicians
Despite the fundamental works Arabic mathematicians have done on the development of Algebra and algebraic geometry, Western historians in the 18th and early 19th century still regarded it as a fact that Classical science and math were unique phenomena of the West. Even though some math contributions from Arab mathematicians are occasionally acknowledged, they are considered to be "outside history or only integrated in so far as it contributed to science, which is essentially European", and just some technical innovations to the Greek heritage rather than open up a completely new branch of mathematics. In the French philosopher Ernest Renan's work, Arabic math is merely "a reflection of Greece, combined with Persian and Indian influences". And according to Duhem, "Arabic science only reproduced the teachings received from Greek science". Besides being considered as merely some insignificant additions or reflections to the great tradition of Greek classical science, math works from Arabic mathematicians are also blamed for lacking rigor and too focused on practical applications and calculations, and this is why Western historians argued they could never reach the level of Greek mathematicians. As Tannery wrote, Arabic math "in no way superseded the level attained by Diophantus". On the other hand, they perceived that Western mathematicians went into a very different way both in its method employed and ultimate purpose, "the hallmark of Western science in its Greek origins as well as in its modern renaissance, is its conformity to rigorous standards". Thus, the perceived non-rigorous proof in Arabic mathematicians' book authorizes Bourbaki to exclude the Arabic period when he retraced the evolution of algebra. And instead, the history of classical algebra is written as the work of the Renaissance and the origin of algebraic geometry is traced back to Descartes, while Arabic mathematicians' contributions are ignored deliberately. In Rashed's words: "To justify the exclusion of science written in Arabic from the history of science, one invokes its absence of rigor, its calculatory appearance and its practical aims. Furthermore, strictly dependent on Greek science and, lastly, incapable of introducing experimental norms, scientists of that time were relegated to the role of conscientious guardians of the Hellenistic museum."
In 18th century Germany and France, the prevailing Orientalist view was "East and West oppose each other not as geographical but as historical positivities", which labeled "Rationalism" as the essence of the West, while the "Call of the Orient" movement emerged in the 19th century was interpreted as "against Rationalism" and a return to a more "spiritual and harmonious" lifestyle. Thus, the prevailing Orientalism in that period was one of the main reasons why Arabic mathematicians were often ignored for their contributions, as people outside the West were considered to be lacking the necessary rationality and scientific spirit to made significant contributions to math and science.
Conclusion
The medieval Arab-Islamic world played a crucial role in shaping the trajectory of mathematics, with al-Khwārizmī's algebraic innovations serving as a cornerstone. The dissemination of Arabic mathematics to the West during the Islamic Golden Age, facilitated by cultural exchanges and translations, left a lasting impact on Western mathematical thought. Mathematicians like Al-Battānī, Al-Khayyām, and Abū Kāmil, with their contributions to trigonometry, algebra, and geometry, extended their influence beyond their time. Despite the foundational contributions of Arab mathematicians, Western historians in the 18th and early 19th centuries, influenced by Orientalist views, sometimes marginalized these achievements. The East lacking rationality and scientific spirit perpetuated a biased perspective, hindering the recognition of the significant role played by Arabic mathematics in the development of algebra and other mathematical disciplines. Reevaluating the history of mathematics necessitates acknowledging the interconnectedness of diverse mathematical traditions and dispelling the notion of a uniquely European mathematical heritage. The contributions of Arab mathematicians, marked by practical applications and theoretical innovations, form an integral part of the rich tapestry of mathematical history, and deserves recognition.
Other major figures
- 'Abd al-Hamīd ibn Turk (fl. 830) (quadratics)
- Sind ibn Ali (d. after 864)
- Thabit ibn Qurra (826–901)
- Al-Battānī (before 858 – 929)
- Abū Kāmil (c. 850 – c. 930)
- Abu'l-Hasan al-Uqlidisi (fl. 952) (arithmetic)
- 'Abd al-'Aziz al-Qabisi (d. 967)
- Abū Sahl al-Qūhī (c. 940–1000) (centres of gravity)
- Ibn al-Haytham (c. 965–1040)
- Abū al-Rayḥān al-Bīrūnī (973–1048) (trigonometry)
- Al-Khayyām (1048–1131)
- Ibn Maḍāʾ (c. 1116–1196)
- Ismail al-Jazari (1136–1206)
- Jamshīd al-Kāshī (c. 1380–1429) (decimals and estimation of the circle constant)
Gallery
-
 Engraving of Abū Sahl al-Qūhī's perfect compass to draw conic sections
Engraving of Abū Sahl al-Qūhī's perfect compass to draw conic sections
-
The theorem of Ibn Haytham
See also
- Arabic numerals
- Indian influence on Islamic mathematics in medieval Islam
- History of calculus
- History of geometry
- Science in the medieval Islamic world
- Timeline of science and engineering in the Muslim world
References
- Katz (1993): "A complete history of mathematics of medieval Islam cannot yet be written, since so many of these Arabic manuscripts lie unstudied... Still, the general outline... is known. In particular, Islamic mathematicians fully developed the decimal place-value number system to include decimal fractions, systematised the study of algebra and began to consider the relationship between algebra and geometry, studied and made advances on the major Greek geometrical treatises of Euclid, Archimedes, and Apollonius, and made significant improvements in plane and spherical geometry."
^ Smith (1958), Vol. 1, Chapter VII.4: "In a general way it may be said that the Golden Age of Arabian mathematics was confined largely to the 9th and 10th centuries; that the world owes a great debt to Arab scholars for preserving and transmitting to posterity the classics of Greek mathematics; and that their work was chiefly that of transmission, although they developed considerable originality in algebra and showed some genius in their work in trigonometry." - Lumpkin, Beatrice; Zitler, Siham (1992). "Cairo: Science Academy of the Middle Ages". In Van Sertima, Ivan (ed.). Golden age of the Moor, Volume 11. Transaction Publishers. p. 394. ISBN 1-56000-581-5. "The Islamic mathematicians exercised a prolific influence on the development of science in Europe, enriched as much by their own discoveries as those they had inherited by the Greeks, the Indians, the Syrians, the Babylonians, etc."
- ^ ben Musa, Mohammed (2013-03-28). The Algebra of Mohammed ben Musa. Cambridge University Press. ISBN 978-1-108-05507-9.
- ^ Swetz, Frank J. (2012-08-15). Mathematical Treasures: Mesopotamian Accounting Tokens (Report). Washington, DC: The MAA Mathematical Sciences Digital Library.
- "Extending al-Karaji's Work on Sums of Odd Powers of Integers - Introduction | Mathematical Association of America". maa.org. Retrieved 2023-12-15.
- "algebra". Online Etymology Dictionary.
- Boyer 1991, p. 228.
- Swetz, Frank J. (1993). Learning Activities from the History of Mathematics. Walch Publishing. p. 26. ISBN 978-0-8251-2264-4.
- ^ Gullberg, Jan (1997). Mathematics: From the Birth of Numbers. W. W. Norton. p. 298. ISBN 0-393-04002-X.
- O'Connor, John J.; Robertson, Edmund F., "al-Marrakushi ibn Al-Banna", MacTutor History of Mathematics Archive, University of St Andrews
- O'Connor, John J.; Robertson, Edmund F., "Arabic mathematics: forgotten brilliance?", MacTutor History of Mathematics Archive, University of St Andrews
- Struik 1987, p. 96.
- ^ Boyer 1991, pp. 241–242.
- Struik 1987, p. 97.
- Berggren, J. Lennart; Al-Tūsī, Sharaf Al-Dīn; Rashed, Roshdi (1990). "Innovation and Tradition in Sharaf al-Dīn al-Ṭūsī's al-Muʿādalāt". Journal of the American Oriental Society. 110 (2): 304–309. doi:10.2307/604533. JSTOR 604533.
- ^ Sesiano, Jacques (2000). "Islamic Mathematics". In Selin, Helaine (ed.). Mathematics Across Cultures: The History of Non-Western Mathematics. Springer Netherlands. pp. 137–165. doi:10.1007/978-94-011-4301-1_9. ISBN 978-94-011-4301-1.
- O'Connor, John J.; Robertson, Edmund F., "Abu Mansur ibn Tahir Al-Baghdadi", MacTutor History of Mathematics Archive, University of St Andrews
- Allen, G. Donald (n.d.). "The History of Infinity" (PDF). Texas A&M University. Retrieved 7 September 2016.
- Struik 1987, p. 93
- Rosen 1831, p. v–vi.
- Toomer, Gerald (1990). "Al-Khwārizmī, Abu Ja'far Muḥammad ibn Mūsā". In Gillispie, Charles Coulston (ed.). Dictionary of Scientific Biography. Vol. 7. New York: Charles Scribner's Sons. ISBN 0-684-16962-2 – via Encyclopedia.com.
- Nallino 1939.
- O'Connor, John J.; Robertson, Edmund F., "Abu Abd Allah Muhammad ibn Muadh Al-Jayyani", MacTutor History of Mathematics Archive, University of St Andrews
- Berggren 2007, p. 518.
- ^ Rashed, R. (1994-06-30). The Development of Arabic Mathematics: Between Arithmetic and Algebra. Springer. pp. 36–37. ISBN 9780792325659.
- ^ Mat Rofa Bin Ismail (2008), "Algebra in Islamic Mathematics", in Helaine Selin (ed.), Encyclopaedia of the History of Science, Technology, and Medicine in Non-Western Cultures, vol. 1 (2nd ed.), Springer, p. 115, ISBN 9781402045592
- Schwartz, R. K. (2004). Issues in the Origin and Development of Hisab al-Khata'ayn (Calculation by Double False Position) (PDF). Eighth North African Meeting on the History of Arab Mathematics. Radès, Tunisia. Archived from the original (PDF) on 2014-05-16. Retrieved 2012-06-08. "Issues in the Origin and Development of Hisab al-Khata'ayn (Calculation by Double False Position)". Archived from the original (.doc) on 2011-09-15.
- ^ Masters, Barry R. (2011-06-08). "Biomedical ethics, 7th edition David DeGrazia, Thomas A. Mappes, Jeffrey Brand-Ballard: 2010, Softcover, 732pp, ISBN-9780073407456 £171.15 McGraw-Hill Incorporated". Graefe's Archive for Clinical and Experimental Ophthalmology. 250 (1): 159–160. doi:10.1007/s00417-011-1640-x. ISSN 0721-832X.
- "Edited by", Contributions to Non-Standard Analysis, Elsevier, pp. iii, 1972, retrieved 2023-12-15
- ^ Rashed, Roshdi (2014-08-21). Classical Mathematics from Al-Khwarizmi to Descartes. Routledge. ISBN 978-1-317-62239-0.
- ^ Masters, Barry R. (2011-06-08). "Biomedical ethics, 7th edition David DeGrazia, Thomas A. Mappes, Jeffrey Brand-Ballard: 2010, Softcover, 732pp, ISBN-9780073407456 £171.15 McGraw-Hill Incorporated". Graefe's Archive for Clinical and Experimental Ophthalmology. 250 (1): 159–160. doi:10.1007/s00417-011-1640-x. ISSN 0721-832X.
- ^ Rashed, Roshdi (1994). "The Development of Arabic Mathematics: Between Arithmetic and Algebra". Boston Studies in the Philosophy of Science. doi:10.1007/978-94-017-3274-1. ISSN 0068-0346.
Sources
- Berggren, J. Lennart (2007). "Mathematics in Medieval Islam". In Victor J. Katz (ed.). The Mathematics of Egypt, Mesopotamia, China, India, and Islam: A Sourcebook (2nd ed.). Princeton, New Jersey: Princeton University Press. ISBN 978-0-691-11485-9.
- Boyer, Carl B. (1991), "Greek Trigonometry and Mensuration, and The Arabic Hegemony", A History of Mathematics (2nd ed.), New York City: John Wiley & Sons, ISBN 0-471-54397-7
- Katz, Victor J. (1993). A History of Mathematics: An Introduction. HarperCollins college publishers. ISBN 0-673-38039-4.
- Nallino, C.A. (1939), "Al-Ḥuwārismī e il suo rifacimento della Geografia di Tolomeo", Raccolta di scritti editi e inediti (in Italian), vol. V, Rome: Istituto per l'Oriente, pp. 458–532
- Rosen, Fredrick (1831). The Algebra of Mohammed Ben Musa. Kessinger Publishing. ISBN 1-4179-4914-7.
- Smith, David E. (1958). History of Mathematics. Dover Publications. ISBN 0-486-20429-4.
- Struik, Dirk J. (1987), A Concise History of Mathematics (4th rev. ed.), Dover Publications, ISBN 0-486-60255-9
Further reading
- Books on Islamic mathematics
- Berggren, J. Lennart (1986). Episodes in the Mathematics of Medieval Islam. New York: Springer-Verlag. ISBN 0-387-96318-9.
- Review: Toomer, Gerald J.; Berggren, J. L. (1988). "Episodes in the Mathematics of Medieval Islam". American Mathematical Monthly. 95 (6). Mathematical Association of America: 567. doi:10.2307/2322777. JSTOR 2322777.
- Review: Hogendijk, Jan P.; Berggren, J. L. (1989). "Episodes in the Mathematics of Medieval Islam by J. Lennart Berggren". Journal of the American Oriental Society. 109 (4). American Oriental Society: 697–698. doi:10.2307/604119. JSTOR 604119.
- Daffa', Ali Abdullah al- (1977). The Muslim contribution to mathematics. London: Croom Helm. ISBN 0-85664-464-1.
- Ronan, Colin A. (1983). The Cambridge Illustrated History of the World's Science. Cambridge University Press. ISBN 0-521-25844-8.
- Rashed, Roshdi (2001). The Development of Arabic Mathematics: Between Arithmetic and Algebra. Translated by A. F. W. Armstrong. Springer. ISBN 0-7923-2565-6.
- Youschkevitch, Adolf P.; Rozenfeld, Boris A. (1960). Die Mathematik der Länder des Ostens im Mittelalter. Berlin.
{{cite book}}: CS1 maint: location missing publisher (link) Sowjetische Beiträge zur Geschichte der Naturwissenschaft pp. 62–160. - Youschkevitch, Adolf P. (1976). Les mathématiques arabes: VIII–XV siècles. translated by M. Cazenave and K. Jaouiche. Paris: Vrin. ISBN 978-2-7116-0734-1.
- Book chapters on Islamic mathematics
- Lindberg, D.C., and M. H. Shank, eds. The Cambridge History of Science. Volume 2: Medieval Science (Cambridge UP, 2013), chapters 2 and 3 mathematics in Islam.
- Cooke, Roger (1997). "Islamic Mathematics". The History of Mathematics: A Brief Course. Wiley-Interscience. ISBN 0-471-18082-3.
- Books on Islamic science
- Daffa, Ali Abdullah al-; Stroyls, J.J. (1984). Studies in the exact sciences in medieval Islam. New York: Wiley. ISBN 0-471-90320-5.
- Kennedy, E. S. (1984). Studies in the Islamic Exact Sciences. Syracuse Univ Press. ISBN 0-8156-6067-7.
- Books on the history of mathematics
- Joseph, George Gheverghese (2000). The Crest of the Peacock: Non-European Roots of Mathematics (2nd ed.). Princeton University Press. ISBN 0-691-00659-8. (Reviewed: Katz, Victor J.; Joseph, George Gheverghese (1992). "The Crest of the Peacock: Non-European Roots of Mathematics by George Gheverghese Joseph". The College Mathematics Journal. 23 (1). Mathematical Association of America: 82–84. doi:10.2307/2686206. JSTOR 2686206.)
- Youschkevitch, Adolf P. (1964). Gesichte der Mathematik im Mittelalter. Leipzig: BG Teubner Verlagsgesellschaft.
- Journal articles on Islamic mathematics
- Høyrup, Jens. “The Formation of «Islamic Mathematics»: Sources and Conditions”. Filosofi og Videnskabsteori på Roskilde Universitetscenter. 3. Række: Preprints og Reprints 1987 Nr. 1.
- Bibliographies and biographies
- Brockelmann, Carl. Geschichte der Arabischen Litteratur. 1.–2. Band, 1.–3. Supplementband. Berlin: Emil Fischer, 1898, 1902; Leiden: Brill, 1937, 1938, 1942.
- Sánchez Pérez, José A. (1921). Biografías de Matemáticos Árabes que florecieron en España. Madrid: Estanislao Maestre.
- Sezgin, Fuat (1997). Geschichte Des Arabischen Schrifttums (in German). Brill Academic Publishers. ISBN 90-04-02007-1.
- Suter, Heinrich (1900). Die Mathematiker und Astronomen der Araber und ihre Werke. Abhandlungen zur Geschichte der Mathematischen Wissenschaften Mit Einschluss Ihrer Anwendungen, X Heft. Leipzig.
{{cite book}}: CS1 maint: location missing publisher (link)
- Television documentaries
- Marcus du Sautoy (presenter) (2008). "The Genius of the East". The Story of Maths. BBC.
- Jim Al-Khalili (presenter) (2010). Science and Islam. BBC.
External links
- Hogendijk, Jan P. (January 1999). "Bibliography of Mathematics in Medieval Islamic Civilization".
- O'Connor, John J.; Robertson, Edmund F. (1999), "Arabic mathematics: forgotten brilliance?", MacTutor History of Mathematics Archive, University of St Andrews
- Richard Covington, Rediscovering Arabic Science, 2007, Saudi Aramco World
- List of Inventions and Discoveries in Mathematics During the Islamic Golden Age
| History of mathematics (timeline) | |
|---|---|
| By topic |
|
| Numeral systems | |
| By ancient cultures | |
| Controversies | |
| Other |
|
| Mathematics in the medieval Islamic world | ||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Mathematicians |
|  | ||||||||||||||||
| Mathematical works | ||||||||||||||||||
| Concepts | ||||||||||||||||||
| Centers | ||||||||||||||||||
| Influences | ||||||||||||||||||
| Influenced | ||||||||||||||||||
| Related | ||||||||||||||||||
| Islamic studies | |
|---|---|
| Arts | |
| Economics | |
| History | |
| Law and politics | |
| Philosophy | |
| Medieval science | |
| Other fields | |
 , with a and b positive, he would note that the maximum point of the curve
, with a and b positive, he would note that the maximum point of the curve  occurs at
occurs at  , and that the equation would have no solutions, one solution or two solutions, depending on whether the height of the curve at that point was less than, equal to, or greater than a. His surviving works give no indication of how he discovered his formulae for the maxima of these curves. Various conjectures have been proposed to account for his discovery of them.
, and that the equation would have no solutions, one solution or two solutions, depending on whether the height of the curve at that point was less than, equal to, or greater than a. His surviving works give no indication of how he discovered his formulae for the maxima of these curves. Various conjectures have been proposed to account for his discovery of them.
 .
.