| Revision as of 02:49, 27 July 2018 edit49.219.177.6 (talk) Undid revision 852165874 by ClueBot NG (talk)Tags: Undo reverting anti-vandal bot nonsense characters← Previous edit | Revision as of 02:50, 27 July 2018 edit undo49.219.177.6 (talk) →Some notable numbersNext edit → | ||
| Line 2,484: | Line 2,484: | ||
| abcde * 11 * E0E1 = abcdeabcde | abcde * 11 * E0E1 = abcdeabcde | ||
| abcdef * 5 * 25 * EE01 = abcdefabcdef | abcdef * 5 * 25 * EE01 = abcdefabcdef | ||
| (where a, b, c, d, e, f are any digits) | |||
| == Prime numbers== | == Prime numbers== | ||
Revision as of 02:50, 27 July 2018
Not to be confused with Dewey Decimal Classification or duodecimo. This article contains special characters. Without proper rendering support, you may see question marks, boxes, or other symbols.| Part of a series on | ||||
| Numeral systems | ||||
|---|---|---|---|---|
Place-value notation
|
||||
Sign-value notation
|
||||
| List of numeral systems | ||||
| This article may be too long to read and navigate comfortably. Consider splitting content into sub-articles, condensing it, or adding subheadings. Please discuss this issue on the article's talk page. (March 2018) |
The duodecimal system (also known as base 12 or dozenal) is a positional notation numeral system using twelve as its base. In this system, the number ten may be written by a rotated "2" (2) and the number eleven by a rotated "3" (3). This notation was introduced by Sir Isaac Pitman. These digit forms are available as Unicode characters on computerized systems since June 2015 as ↊ (Code point 218A) and ↋ (Code point 218B), respectively. Other notations use "A", "T", or "X" for ten and "B" or "E" for eleven. The number twelve (that is, the number written as "12" in the base ten numerical system) is instead written as "10" in duodecimal (meaning "1 dozen and 0 units", instead of "1 ten and 0 units"), whereas the digit string "12" means "1 dozen and 2 units" (i.e. the same number that in decimal is written as "14"). Similarly, in duodecimal "100" means "1 gross", "1000" means "1 great gross", and "0.1" means "1 twelfth" (instead of their decimal meanings "1 hundred", "1 thousand", and "1 tenth").
The number twelve, a superior highly composite number, is the smallest number with four non-trivial factors (2, 3, 4, 6), and the smallest to include as factors all four numbers (1 to 4) within the subitizing range, and the smallest abundant number. As a result of this increased factorability of the radix and its divisibility by a wide range of the most elemental numbers (whereas ten has only two non-trivial factors: 2 and 5, and not 3, 4, or 6), duodecimal representations fit more easily than decimal ones into many common patterns, as evidenced by the higher regularity observable in the duodecimal multiplication table. As a result, duodecimal has been described as the optimal number system. Of its factors, 2 and 3 are prime, which means the reciprocals of all 3-smooth numbers (such as 2, 3, 4, 6, 8, 9, 12, 16, 18, 24, 27, 32, 36, ...) have a terminating representation in duodecimal. In particular, the five most elementary fractions (+1⁄2, +1⁄3, +2⁄3, +1⁄4 and +3⁄4) all have a short terminating representation in duodecimal (0.6, 0.4, 0.8, 0.3 and 0.9, respectively), and twelve is the smallest radix with this feature (because it is the least common multiple of 3 and 4). This all makes it a more convenient number system for computing fractions than most other number systems in common use, such as the decimal, vigesimal, binary, octal and hexadecimal systems. Although the trigesimal and sexagesimal systems (where the reciprocals of all 5-smooth numbers terminate) do even better in this respect, this is at the cost of unwieldy multiplication tables and a much larger number of symbols to memorize.
Origin
Languages using duodecimal number systems are uncommon. Languages in the Nigerian Middle Belt such as Janji, Gbiri-Niragu (Gure-Kahugu), Piti, and the Nimbia dialect of Gwandara; the Chepang language of Nepal and the Mahl language of Minicoy Island in India are known to use duodecimal numerals.
Germanic languages have special words for 11 and 12, such as eleven and twelve in English. However, they are considered to come from Proto-Germanic *ainlif and *twalif (respectively one left and two left), both of which were decimal.
Historically, units of time in many civilizations are duodecimal. There are twelve signs of the zodiac, twelve months in a year, and the Babylonians had twelve hours in a day (although at some point this was changed to 24, which is twice as 12). Traditional Chinese calendars, clocks, and compasses are based on the twelve Earthly Branches. There are 12 inches in an imperial foot, 12 troy ounces in a troy pound, 12 old British pence in a shilling, 24 (12×2) hours in a day, and many other items counted by the dozen, gross (144, square of 12) or great gross (1728, cube of 12). The Romans used a fraction system based on 12, including the uncia which became both the English words ounce and inch. Pre-decimalisation, Ireland and the United Kingdom used a mixed duodecimal-vigesimal currency system (12 pence = 1 shilling, 20 shillings or 240 pence to the pound sterling or Irish pound), and Charlemagne established a monetary system that also had a mixed base of twelve and twenty, the remnants of which persist in many places.
| Table of units from a base of 12 | |||||
|---|---|---|---|---|---|
| Relative value |
French unit of length |
English unit of length |
English (Troy) unit of weight |
Roman unit of weight |
English unit of mass |
| 12 | pied | foot | pound | libra | |
| 12 | pouce | inch | ounce | uncia | slinch |
| 12 | ligne | line | 2 scruples | 2 scrupula | slug |
| 12 | point | point | seed | siliqua | |
The importance of 12 has been attributed to the number of lunar cycles in a year, and also to the fact that humans have 12 finger bones (phalanges) on one hand (three on each of four fingers). It is possible to count to 12 with the thumb acting as a pointer, touching each finger bone in turn. A traditional finger counting system still in use in many regions of Asia works in this way, and could help to explain the occurrence of numeral systems based on 12 and 60 besides those based on 10, 20 and 5. In this system, the one (usually right) hand counts repeatedly to 12, displaying the number of iterations on the other (usually left), until five dozens, i. e. the 60, are full.
Notations and pronunciations
Transdecimal symbols
In a duodecimal place system twelve is written as 10, but there are numerous proposals for how to write ten and eleven. The simplified notations use only basic and easy to access letters such as T and E (for ten and eleven), X and Z, t and e, d and k, others use A and B or a and b as in the hexadecimal system. Some employ Greek letters such as δ (standing for Greek δέκα 'ten') and ε (for Greek ένδεκα 'eleven'), or τ and ε. Frank Emerson Andrews, an early American advocate for duodecimal, suggested and used in his book New Numbers an X (from the Roman numeral for ten) and a script E (ℰ, U+2130).
The Dozenal Society of Great Britain proposes a rotated digit two 2 (↊, U+218A) for ten and a reversed or rotated digit three 3 (↋, U+218B) for eleven. This notation was introduced by Sir Isaac Pitman.
Until 2015, the Dozenal Society of America (DSA) used ![]() and
and ![]() , the symbols devised by William Addison Dwiggins. After the Pitman digits (↊, U+218A and ↋, U+218B) were added to Unicode in 2015, the DSA took a vote and then began publishing content using the Pitman digits instead. They still use the letters X and E as the equivalent in ASCII text.
, the symbols devised by William Addison Dwiggins. After the Pitman digits (↊, U+218A and ↋, U+218B) were added to Unicode in 2015, the DSA took a vote and then began publishing content using the Pitman digits instead. They still use the letters X and E as the equivalent in ASCII text.
Other proposals are more creative or aesthetic, for example, Edna Kramer in her 1951 book The Main Stream of Mathematics used a six-pointed asterisk (sextile) ⚹ for ten and a hash (or octothorpe) # for eleven. The symbols were chosen because they are available in typewriters and already present in telephone dials. This notation was used in publications of the Dozenal Society of America in the period 1974–2008. Many don't use any of the Hindu-Arabic symbols, under the principle of "separate identity."
Base notation
There are also varying proposals of how to distinguish a duodecimal number from a decimal one, or one in a different base. They include italicizing duodecimal numbers (54 = 64), adding a "Humphrey point" (a semicolon ";" instead of a decimal point ".") to duodecimal numbers (54; = 64.) (54;0 = 64.0), or some combination of the two. More also add extra marking to one or more bases. Others use subscript or affixed labels to indicate the base, allowing for more than decimal and duodecimal to be represented:
| Common Base | Abb. | Letter | Cardinal | Decimal | Duodecimal |
|---|---|---|---|---|---|
| binary | bin | b | two | 2 | 2 |
| octal | oct | o | eight | 8 | 8 |
| decimal | dec | d | ten | 10 | ↊ |
| dozenal (duodecimal) | doz | z | twelve | 12 | 10 |
| hexadecimal | hex | x | sixteen | 16 | 14 |
This allows one to write "54z = 64d," "54twelve = 64ten" or "doz 54 = dec 64." In programming, binary, octal, and hexadecimal often use a similar scheme: a binary number starts with 0b, octal with 0o, and hexadecimal with 0x.
Pronunciation
The Dozenal Society of America suggests the pronunciation of ten and eleven as "dek" and "el", each order has its own name and the prefix e- is added for fractions. The symbol corresponding to the decimal point or decimal comma, separating the whole number part from the fractional part, is the semicolon ";". The overall system is:
| Duodecimal | Name | Decimal | Duodecimal fraction | Name |
|---|---|---|---|---|
| 1 | one | 1 | ||
| 10 | do | 12 | 0;1 | edo |
| 100 | gro | 144 | 0;01 | egro |
| 1,000 | mo | 1,728 | 0;001 | emo |
| 10,000 | do-mo | 20,736 | 0;000,1 | edo-mo |
| 100,000 | gro-mo | 248,832 | 0;000,01 | egro-mo |
| 1,000,000 | bi-mo | 2,985,984 | 0;000,001 | ebi-mo |
| 1,000,000,000 | tri-mo | 5,159,780,352 | 0;000,000,001 | etri-mo |
Multiple digits in this are pronounced differently. 12 is "one do two", 30 is "three do", 100 is "one gro", BA9 (ET9) is "el gro dek do nine", B8,65A,300 (E8,65T,300) is "el do eight bi-mo, six gro five do dek mo, three gro", and so on.
Advocacy and "dozenalism"
William James Sidis used 12 as the base for his constructed language Vendergood in 1906, noting it being the smallest number with four factors and the prevalence in commerce.
The case for the duodecimal system was put forth at length in F. Emerson Andrews' 1935 book New Numbers: How Acceptance of a Duodecimal Base Would Simplify Mathematics. Emerson noted that, due to the prevalence of factors of twelve in many traditional units of weight and measure, many of the computational advantages claimed for the metric system could be realized either by the adoption of ten-based weights and measure or by the adoption of the duodecimal number system.

Both the Dozenal Society of America and the Dozenal Society of Great Britain promote widespread adoption of the base-twelve system. They use the word "dozenal" instead of "duodecimal" to avoid the more overtly base-ten terminology. It should be noted that the etymology of 'dozenal' is itself also an expression based on base-ten terminology since 'dozen' is a direct derivation of the French word 'douzaine' which is a derivative of the French word for twelve, douze which is related to the old French word 'doze' from Latin 'duodecim'.
It has been suggested by some members of the Dozenal Society of America and Duodecimal Society of Great Britain that a more apt word would be 'uncial'. Uncial is a derivation of the Latin word 'one-twelfth' which is 'uncia' and also the base-twelve analogue of the Latin word 'one-tenth' which is 'decima'. In the same manner as decimal comes from the Latin word for one-tenth decima, (Latin for ten was decem), the direct analogue for a base-twelve system is uncial. An early use of this word can be found in Vol 1 Issue 2 of The Duodecimal Bulletin of the DSA dated June 1945 in which a submission on page 9 by a Pvt William S. Crosby titled "The Uncial Jottings of a Harried Infantryman", he includes the same argument for the word 'uncial'. Although not accepted by either of these two 'Uncial' societies, the use is beginning to grow.
The renowned mathematician and mental calculator Alexander Craig Aitken was an outspoken advocate of the advantages and superiority of duodecimal over decimal:
The duodecimal tables are easy to master, easier than the decimal ones; and in elementary teaching they would be so much more interesting, since young children would find more fascinating things to do with twelve rods or blocks than with ten. Anyone having these tables at command will do these calculations more than one-and-a-half times as fast in the duodecimal scale as in the decimal. This is my experience; I am certain that even more so it would be the experience of others.
— A. C. Aitken, "Twelves and Tens" in The Listener (January 25, 1962)
But the final quantitative advantage, in my own experience, is this: in varied and extensive calculations of an ordinary and not unduly complicated kind, carried out over many years, I come to the conclusion that the efficiency of the decimal system might be rated at about 65 or less, if we assign 100 to the duodecimal.
— A. C. Aitken, The Case Against Decimalisation (1962)
In Jorge Luis Borges' short story Tlön, Uqbar, Orbis Tertius Herbert Ashe, a melancholy English engineer, working for the Southern Argentine Railway company, is converting a duodecimal number system to a hexadecimal system. He leaves behind on his death in 1937 a manuscript Orbis Tertius that posthumously identifies him as one of the anonymous authors of the encyclopaedia of Tlön.
In Leo Frankowski's Conrad Stargard novels, Conrad introduces a duodecimal system of arithmetic at the suggestion of a merchant, who is accustomed to buying and selling goods in dozens and grosses, rather than tens or hundreds. He then invents an entire system of weights and measures in base twelve, including a clock with twelve hours in a day, rather than twenty-four hours.
In Lee Carroll's Kryon: Alchemy of the Human Spirit, a chapter is dedicated to the advantages of the duodecimal system. The duodecimal system is supposedly suggested by Kryon (a fictional entity believed in by New Age circles) for all-round use, aiming at better and more natural representation of nature of the Universe through mathematics. An individual article "Mathematica" by James D. Watt (included in the above publication) exposes a few of the unusual symmetry connections between the duodecimal system and the golden ratio, as well as provides numerous number symmetry-based arguments for the universal nature of the base-12 number system.
In "Little Twelvetoes", American television series Schoolhouse Rock! portrayed an alien child using base-twelve arithmetic, using "dek", "el" and "doh" as names for ten, eleven and twelve, and Andrews' script-X and script-E for the digit symbols.
In computing
In March 2013, a proposal was submitted to include the digit forms for ten and eleven propagated by the Dozenal Societies of Great Britain and America in the Unicode Standard. Of these, the British forms were accepted for encoding as characters at code points U+218A turned digit two (↊) and U+218B turned digit three (↋) They have been included in the Unicode 8.0 release in June 2015.
Unicode points U+218C and U+218D seem to be reserved for the Dwiggins digits (stylized X and E).
Few fonts support these new characters, but Abibas, EB Garamond, Everson Mono, Squarish Sans CT, and Symbola do.
Also, the turned digits two and three are available in LaTeX as \textturntwo and \textturnthree.
Duodecimal clock
- Dozenal Clock by Joshua Harkey
- Dozenal Clock with four hands and a digital display, in several variants, by Paul Rapoport
Duodecimal metric systems
Systems of measurement proposed by dozenalists include:
- Tom Pendlebury's TGM system
- Takashi Suga's Universal Unit System
Comparison to other numeral systems
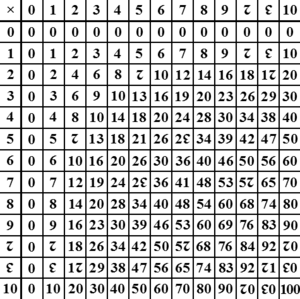
The number 12 has six factors, which are 1, 2, 3, 4, 6, and 12, of which 2 and 3 are prime. The decimal system has only four factors, which are 1, 2, 5, and 10, of which 2 and 5 are prime. Vigesimal (base 20) adds two factors to those of ten, namely 4 and 20, but no additional prime factor. Although twenty has 6 factors, 2 of them prime, similarly to twelve, it is also a much larger base, and so the digit set and the multiplication table are much larger. Binary has only two factors, 1 and 2, the latter being prime. Hexadecimal (base 16) has five factors, adding 4, 8 and 16 to those of 2, but no additional prime. Trigesimal (base 30) is the smallest system that has three different prime factors (all of the three smallest primes: 2, 3 and 5) and it has eight factors in total (1, 2, 3, 5, 6, 10, 15, and 30). Sexagesimal—which the ancient Sumerians and Babylonians among others actually used—adds the four convenient factors 4, 12, 20, and 60 to this but no new prime factors. The smallest system that has four different prime factors is base 210 and the pattern follows the primorials. In all base systems, there are similarities to the representation of multiples of numbers which are one less than the base.
| × | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ᘔ | Ɛ | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 1ᘔ | 1Ɛ | 20 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ᘔ | Ɛ | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 1ᘔ | 1Ɛ | 20 |
| 2 | 0 | 2 | 4 | 6 | 8 | ᘔ | 10 | 12 | 14 | 16 | 18 | 1ᘔ | 20 | 22 | 24 | 26 | 28 | 2ᘔ | 30 | 32 | 34 | 36 | 38 | 3ᘔ | 40 |
| 3 | 0 | 3 | 6 | 9 | 10 | 13 | 16 | 19 | 20 | 23 | 26 | 29 | 30 | 33 | 36 | 39 | 40 | 43 | 46 | 49 | 50 | 53 | 56 | 59 | 60 |
| 4 | 0 | 4 | 8 | 10 | 14 | 18 | 20 | 24 | 28 | 30 | 34 | 38 | 40 | 44 | 48 | 50 | 54 | 58 | 60 | 64 | 68 | 70 | 74 | 78 | 80 |
| 5 | 0 | 5 | ᘔ | 13 | 18 | 21 | 26 | 2Ɛ | 34 | 39 | 42 | 47 | 50 | 55 | 5ᘔ | 63 | 68 | 71 | 76 | 7Ɛ | 84 | 89 | 92 | 97 | ᘔ0 |
| 6 | 0 | 6 | 10 | 16 | 20 | 26 | 30 | 36 | 40 | 46 | 50 | 56 | 60 | 66 | 70 | 76 | 80 | 86 | 90 | 96 | ᘔ0 | ᘔ6 | Ɛ0 | Ɛ6 | 100 |
| 7 | 0 | 7 | 12 | 19 | 24 | 2Ɛ | 36 | 41 | 48 | 53 | 5ᘔ | 65 | 70 | 77 | 82 | 89 | 94 | 9Ɛ | ᘔ6 | Ɛ1 | Ɛ8 | 103 | 10ᘔ | 115 | 120 |
| 8 | 0 | 8 | 14 | 20 | 28 | 34 | 40 | 48 | 54 | 60 | 68 | 74 | 80 | 88 | 94 | ᘔ0 | ᘔ8 | Ɛ4 | 100 | 108 | 114 | 120 | 128 | 134 | 140 |
| 9 | 0 | 9 | 16 | 23 | 30 | 39 | 46 | 53 | 60 | 69 | 76 | 83 | 90 | 99 | ᘔ6 | Ɛ3 | 100 | 109 | 116 | 123 | 130 | 139 | 146 | 153 | 160 |
| ᘔ | 0 | ᘔ | 18 | 26 | 34 | 42 | 50 | 5ᘔ | 68 | 76 | 84 | 92 | ᘔ0 | ᘔᘔ | Ɛ8 | 106 | 114 | 122 | 130 | 13ᘔ | 148 | 156 | 164 | 172 | 180 |
| Ɛ | 0 | Ɛ | 1ᘔ | 29 | 38 | 47 | 56 | 65 | 74 | 83 | 92 | ᘔ1 | Ɛ0 | ƐƐ | 10ᘔ | 119 | 128 | 137 | 146 | 155 | 164 | 173 | 182 | 191 | 1ᘔ0 |
| 10 | 0 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | ᘔ0 | Ɛ0 | 100 | 110 | 120 | 130 | 140 | 150 | 160 | 170 | 180 | 190 | 1ᘔ0 | 1Ɛ0 | 200 |
| 11 | 0 | 11 | 22 | 33 | 44 | 55 | 66 | 77 | 88 | 99 | ᘔᘔ | ƐƐ | 110 | 121 | 132 | 143 | 154 | 165 | 176 | 187 | 198 | 1ᘔ9 | 1Ɛᘔ | 20Ɛ | 220 |
| 12 | 0 | 12 | 24 | 36 | 48 | 5ᘔ | 70 | 82 | 94 | ᘔ6 | Ɛ8 | 10ᘔ | 120 | 132 | 144 | 156 | 168 | 17ᘔ | 190 | 1ᘔ2 | 1Ɛ4 | 206 | 218 | 22ᘔ | 240 |
| 13 | 0 | 13 | 26 | 39 | 50 | 63 | 76 | 89 | ᘔ0 | Ɛ3 | 106 | 119 | 130 | 143 | 156 | 169 | 180 | 193 | 1ᘔ6 | 1Ɛ9 | 210 | 223 | 236 | 249 | 260 |
| 14 | 0 | 14 | 28 | 40 | 54 | 68 | 80 | 94 | ᘔ8 | 100 | 114 | 128 | 140 | 154 | 168 | 180 | 194 | 1ᘔ8 | 200 | 214 | 228 | 240 | 254 | 268 | 280 |
| 15 | 0 | 15 | 2ᘔ | 43 | 58 | 71 | 86 | 9Ɛ | Ɛ4 | 109 | 122 | 137 | 150 | 165 | 17ᘔ | 193 | 1ᘔ8 | 201 | 216 | 22Ɛ | 244 | 259 | 272 | 287 | 2ᘔ0 |
| 16 | 0 | 16 | 30 | 46 | 60 | 76 | 90 | ᘔ6 | 100 | 116 | 130 | 146 | 160 | 176 | 190 | 1ᘔ6 | 200 | 216 | 230 | 246 | 260 | 276 | 290 | 2ᘔ6 | 300 |
| 17 | 0 | 17 | 32 | 49 | 64 | 7Ɛ | 96 | Ɛ1 | 108 | 123 | 13ᘔ | 155 | 170 | 187 | 1ᘔ2 | 1Ɛ9 | 214 | 22Ɛ | 246 | 261 | 278 | 293 | 2ᘔᘔ | 305 | 320 |
| 18 | 0 | 18 | 34 | 50 | 68 | 84 | ᘔ0 | Ɛ8 | 114 | 130 | 148 | 164 | 180 | 198 | 1Ɛ4 | 210 | 228 | 244 | 260 | 278 | 294 | 2Ɛ0 | 308 | 324 | 340 |
| 19 | 0 | 19 | 36 | 53 | 70 | 89 | ᘔ6 | 103 | 120 | 139 | 156 | 173 | 190 | 1ᘔ9 | 206 | 223 | 240 | 259 | 276 | 293 | 2Ɛ0 | 309 | 326 | 343 | 360 |
| 1ᘔ | 0 | 1ᘔ | 38 | 56 | 74 | 92 | Ɛ0 | 10ᘔ | 128 | 146 | 164 | 182 | 1ᘔ0 | 1Ɛᘔ | 218 | 236 | 254 | 272 | 290 | 2ᘔᘔ | 308 | 326 | 344 | 362 | 380 |
| 1Ɛ | 0 | 1Ɛ | 3ᘔ | 59 | 78 | 97 | Ɛ6 | 115 | 134 | 153 | 172 | 191 | 1Ɛ0 | 20Ɛ | 22ᘔ | 249 | 268 | 287 | 2ᘔ6 | 305 | 324 | 343 | 362 | 381 | 3ᘔ0 |
| 20 | 0 | 20 | 40 | 60 | 80 | ᘔ0 | 100 | 120 | 140 | 160 | 180 | 1ᘔ0 | 200 | 220 | 240 | 260 | 280 | 2ᘔ0 | 300 | 320 | 340 | 360 | 380 | 3ᘔ0 | 400 |
Conversion tables to and from decimal
To convert numbers between bases, one can use the general conversion algorithm (see the relevant section under positional notation). Alternatively, one can use digit-conversion tables. The ones provided below can be used to convert any duodecimal number between 0.01 and ƐƐƐ,ƐƐƐ.ƐƐ to decimal, or any decimal number between 0.01 and 999,999.99 to duodecimal. To use them, the given number must first be decomposed into a sum of numbers with only one significant digit each. For example:
- 123,456.78 = 100,000 + 20,000 + 3,000 + 400 + 50 + 6 + 0.7 + 0.08
This decomposition works the same no matter what base the number is expressed in. Just isolate each non-zero digit, padding them with as many zeros as necessary to preserve their respective place values. If the digits in the given number include zeroes (for example, 102,304.05), these are, of course, left out in the digit decomposition (102,304.05 = 100,000 + 2,000 + 300 + 4 + 0.05). Then the digit conversion tables can be used to obtain the equivalent value in the target base for each digit. If the given number is in duodecimal and the target base is decimal, we get:
- (duodecimal) 100,000 + 20,000 + 3,000 + 400 + 50 + 6 + 0.7 + 0.08 = (decimal) 248,832 + 41,472 + 5,184 + 576 + 60 + 6 + 0.583333333333... + 0.055555555555...
Now, because the summands are already converted to base ten, the usual decimal arithmetic is used to perform the addition and recompose the number, arriving at the conversion result:
Duodecimal -----> Decimal
100,000 = 248,832
20,000 = 41,472
3,000 = 5,184
400 = 576
50 = 60
+ 6 = + 6
0.7 = 0.583333333333...
0.08 = 0.055555555555...
--------------------------------------------
123,456.78 = 296,130.638888888888...
That is, (duodecimal) 123,456.78 equals (decimal) 296,130.638 ≈ 296,130.64
If the given number is in decimal and the target base is duodecimal, the method is basically same. Using the digit conversion tables:
(decimal) 100,000 + 20,000 + 3,000 + 400 + 50 + 6 + 0.7 + 0.08 = (duodecimal) 49,ᘔ54 + Ɛ,6ᘔ8 + 1,8ᘔ0 + 294 + 42 + 6 + 0.849724972497249724972497... + 0.0Ɛ62ᘔ68781Ɛ05915343ᘔ0Ɛ62...
However, in order to do this sum and recompose the number, now the addition tables for the duodecimal system have to be used, instead of the addition tables for decimal most people are already familiar with, because the summands are now in base twelve and so the arithmetic with them has to be in duodecimal as well. In decimal, 6 + 6 equals 12, but in duodecimal it equals 10; so, if using decimal arithmetic with duodecimal numbers one would arrive at an incorrect result. Doing the arithmetic properly in duodecimal, one gets the result:
Decimal -----> Duodecimal
100,000 = 49,ᘔ54
20,000 = Ɛ,6ᘔ8
3,000 = 1,8ᘔ0
400 = 294
50 = 42
+ 6 = + 6
0.7 = 0.849724972497249724972497...
0.08 = 0.0Ɛ62ᘔ68781Ɛ05915343ᘔ0Ɛ62...
--------------------------------------------------------
123,456.78 = 5Ɛ,540.943ᘔ0Ɛ62ᘔ68781Ɛ05915343ᘔ...
That is, (decimal) 123,456.78 equals (duodecimal) 5Ɛ,540.943ᘔ0Ɛ62ᘔ68781Ɛ059153... ≈ 5Ɛ,540.94
Duodecimal to decimal digit conversion
| Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1,000,000 | 2,985,984 | 100,000 | 248,832 | 10,000 | 20,736 | 1,000 | 1,728 | 100 | 144 | 10 | 12 | 1 | 1 | 0.1 | 0.083 | 0.01 | 0.00694 |
| 2,000,000 | 5,971,968 | 200,000 | 497,664 | 20,000 | 41,472 | 2,000 | 3,456 | 200 | 288 | 20 | 24 | 2 | 2 | 0.2 | 0.16 | 0.02 | 0.0138 |
| 3,000,000 | 8,957,952 | 300,000 | 746,496 | 30,000 | 62,208 | 3,000 | 5,184 | 300 | 432 | 30 | 36 | 3 | 3 | 0.3 | 0.25 | 0.03 | 0.02083 |
| 4,000,000 | 11,943,936 | 400,000 | 995,328 | 40,000 | 82,944 | 4,000 | 6,912 | 400 | 576 | 40 | 48 | 4 | 4 | 0.4 | 0.3 | 0.04 | 0.027 |
| 5,000,000 | 14,929,920 | 500,000 | 1,244,160 | 50,000 | 103,680 | 5,000 | 8,640 | 500 | 720 | 50 | 60 | 5 | 5 | 0.5 | 0.416 | 0.05 | 0.03472 |
| 6,000,000 | 17,915,904 | 600,000 | 1,492,992 | 60,000 | 124,416 | 6,000 | 10,368 | 600 | 864 | 60 | 72 | 6 | 6 | 0.6 | 0.5 | 0.06 | 0.0416 |
| 7,000,000 | 20,901,888 | 700,000 | 1,741,824 | 70,000 | 145,152 | 7,000 | 12,096 | 700 | 1,008 | 70 | 84 | 7 | 7 | 0.7 | 0.583 | 0.07 | 0.04861 |
| 8,000,000 | 23,887,872 | 800,000 | 1,990,656 | 80,000 | 165,888 | 8,000 | 13,824 | 800 | 1,152 | 80 | 96 | 8 | 8 | 0.8 | 0.6 | 0.08 | 0.05 |
| 9,000,000 | 26,873,856 | 900,000 | 2,239,488 | 90,000 | 186,624 | 9,000 | 15,552 | 900 | 1,296 | 90 | 108 | 9 | 9 | 0.9 | 0.75 | 0.09 | 0.0625 |
| ᘔ,000,000 | 29,859,840 | ᘔ00,000 | 2,488,320 | ᘔ0,000 | 207,360 | ᘔ,000 | 17,280 | ᘔ00 | 1,440 | ᘔ0 | 120 | ᘔ | 10 | 0.ᘔ | 0.83 | 0.0ᘔ | 0.0694 |
| Ɛ,000,000 | 32,845,824 | Ɛ00,000 | 2,737,152 | Ɛ0,000 | 228,096 | Ɛ,000 | 19,008 | Ɛ00 | 1,584 | Ɛ0 | 132 | Ɛ | 11 | 0.Ɛ | 0.916 | 0.0Ɛ | 0.07638 |
Decimal to duodecimal digit conversion
| Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 100,000 | 49,ᘔ54 | 10,000 | 5,954 | 1,000 | 6Ɛ4 | 100 | 84 | 10 | ᘔ | 1 | 1 | 0.1 | 0.12497 | 0.01 | 0.015343ᘔ0Ɛ62ᘔ68781Ɛ059 |
| 200,000 | 97,8ᘔ8 | 20,000 | Ɛ,6ᘔ8 | 2,000 | 1,1ᘔ8 | 200 | 148 | 20 | 18 | 2 | 2 | 0.2 | 0.2497 | 0.02 | 0.02ᘔ68781Ɛ05915343ᘔ0Ɛ6 |
| 300,000 | 125,740 | 30,000 | 15,440 | 3,000 | 1,8ᘔ0 | 300 | 210 | 30 | 26 | 3 | 3 | 0.3 | 0.37249 | 0.03 | 0.043ᘔ0Ɛ62ᘔ68781Ɛ059153 |
| 400,000 | 173,594 | 40,000 | 1Ɛ,194 | 4,000 | 2,394 | 400 | 294 | 40 | 34 | 4 | 4 | 0.4 | 0.4972 | 0.04 | 0.05915343ᘔ0Ɛ62ᘔ68781Ɛ |
| 500,000 | 201,428 | 50,000 | 24,Ɛ28 | 5,000 | 2,ᘔ88 | 500 | 358 | 50 | 42 | 5 | 5 | 0.5 | 0.6 | 0.05 | 0.07249 |
| 600,000 | 24Ɛ,280 | 60,000 | 2ᘔ,880 | 6,000 | 3,580 | 600 | 420 | 60 | 50 | 6 | 6 | 0.6 | 0.7249 | 0.06 | 0.08781Ɛ05915343ᘔ0Ɛ62ᘔ6 |
| 700,000 | 299,114 | 70,000 | 34,614 | 7,000 | 4,074 | 700 | 4ᘔ4 | 70 | 5ᘔ | 7 | 7 | 0.7 | 0.84972 | 0.07 | 0.0ᘔ0Ɛ62ᘔ68781Ɛ05915343 |
| 800,000 | 326,Ɛ68 | 80,000 | 3ᘔ,368 | 8,000 | 4,768 | 800 | 568 | 80 | 68 | 8 | 8 | 0.8 | 0.9724 | 0.08 | 0.0Ɛ62ᘔ68781Ɛ05915343ᘔ |
| 900,000 | 374,ᘔ00 | 90,000 | 44,100 | 9,000 | 5,260 | 900 | 630 | 90 | 76 | 9 | 9 | 0.9 | 0.ᘔ9724 | 0.09 | 0.10Ɛ62ᘔ68781Ɛ05915343ᘔ |
Conversion of powers
| Exponent | b=2 | b=3 | b=4 | b=5 | b=6 | b=7 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | |
| b | 64 | 54 | 729 | 509 | 4,096 | 2,454 | 15,625 | 9,061 | 46,656 | 23,000 | 117,649 | 58,101 |
| b | 32 | 28 | 243 | 183 | 1,024 | 714 | 3,125 | 1,985 | 7,776 | 4,600 | 16,807 | 9,887 |
| b | 16 | 14 | 81 | 69 | 256 | 194 | 625 | 441 | 1,296 | 900 | 2,401 | 1,481 |
| b | 8 | 8 | 27 | 23 | 64 | 54 | 125 | ᘔ5 | 216 | 160 | 343 | 247 |
| b | 4 | 4 | 9 | 9 | 16 | 14 | 25 | 21 | 36 | 30 | 49 | 41 |
| b | 2 | 2 | 3 | 3 | 4 | 4 | 5 | 5 | 6 | 6 | 7 | 7 |
| b | 0.5 | 0.6 | 0.3 | 0.4 | 0.25 | 0.3 | 0.2 | 0.2497 | 0.16 | 0.2 | 0.142857 | 0.186ᘔ35 |
| b | 0.25 | 0.3 | 0.1 | 0.14 | 0.0625 | 0.09 | 0.04 | 0.05915343ᘔ0 Ɛ62ᘔ68781Ɛ |
0.027 | 0.04 | 0.0204081632653 06122448979591 836734693877551 |
0.02Ɛ322547ᘔ05ᘔ 644ᘔ9380Ɛ908996 741Ɛ615771283Ɛ |
| Exponent | b=8 | b=9 | b=10 | b=11 | b=12 | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | Dec. | Duod. | |
| b | 262,144 | 107,854 | 531,441 | 217,669 | 1,000,000 | 402,854 | 1,771,561 | 715,261 | 2,985,984 | 1,000,000 |
| b | 32,768 | 16,Ɛ68 | 59,049 | 2ᘔ,209 | 100,000 | 49,ᘔ54 | 161,051 | 79,24Ɛ | 248,832 | 100,000 |
| b | 4,096 | 2,454 | 6,561 | 3,969 | 10,000 | 5,954 | 14,641 | 8,581 | 20,736 | 10,000 |
| b | 512 | 368 | 729 | 509 | 1,000 | 6Ɛ4 | 1,331 | 92Ɛ | 1,728 | 1,000 |
| b | 64 | 54 | 81 | 69 | 100 | 84 | 121 | ᘔ1 | 144 | 100 |
| b | 8 | 8 | 9 | 9 | 10 | ᘔ | 11 | Ɛ | 12 | 10 |
| b | 0.125 | 0.16 | 0.1 | 0.14 | 0.1 | 0.12497 | 0.09 | 0.1 | 0.083 | 0.1 |
| b | 0.015625 | 0.023 | 0.012345679 | 0.0194 | 0.01 | 0.015343ᘔ0Ɛ6 2ᘔ68781Ɛ059 |
0.00826446280 99173553719 |
0.0123456789Ɛ | 0.00694 | 0.01 |
Some properties
(In this section, all numbers are written with duodecimal, using ᘔ for ten and Ɛ for eleven, unless other bases mentioned)
All squares end with square digits (i.e. end with 0, 1, 4 or 9), if n is divisible by both 2 and 3, then n ends with 0, if n is not divisible by 2 or 3, then n ends with 1, if n is divisible by 2 but not by 3, then n ends with 4, if n is not divisible by 2 but by 3, then n ends with 9. If the unit digit of n is 0, then the dozens digit of n is either 0 or 3, if the unit digit of n is 1, then the dozens digit of n is even, if the unit digit of n is 4, then the dozen digit of n is 0, 1, 4, 5, 8 or 9, if the unit digit of n is 9, then the dozen digit of n is either 0 or 6. (More specially, all squares of (primes ≥ 5) end with 1)
The digital root of a square is 1, 3, 4, 5 or Ɛ.
No repdigits with more than one digit are squares, in fact, a square cannot end with three same digits except 000. (In contrast, in the decimal (base ᘔ) system squares may end in 444, the smallest example is 32 = ᘔ04 = 1444ᘔ)
No four-digit palindromic numbers are squares. (we can easily to prove it, since all four-digit palindromic number are divisible by 11, and since they are squares, thus they must be divisible by 11 = 121, and the only four-digit palindromic number divisible by 121 are 1331, 2662, 3993, 5225, 6556, 7887, 8ƐƐ8, 9119, ᘔ44ᘔ and Ɛ77Ɛ, but none of them are squares)
| n | n-digit palindromic squares | square roots | number of n-digit palindromic squares |
|---|---|---|---|
| 1 | 1, 4, 9 | 1, 2, 3 | 3 |
| 2 | none | none | 0 |
| 3 | 121, 484 | 11, 22 | 2 |
| 4 | none | none | 0 |
| 5 | 10201, 12321, 14641, 16661, 16Ɛ61, 40804, 41414, 44944 | 101, 111, 121, 12Ɛ, 131, 202, 204, 212 | 8 |
| 6 | 160061 | 42Ɛ | 1 |
| 7 | 1002001, 102ᘔ201, 1093901, 1234321, 148ᘔ841, 4008004, 445ᘔ544, 49ᘔᘔᘔ94 | 1001, 1015, 1047, 1111, 1221, 2002, 2112, 2244 | 8 |
| 8 | none | none | 0 |
| 9 | 100020001, 102030201, 104060401, 1060Ɛ0601, 121242121, 123454321, 125686521, 1420Ɛ0241, 1444ᘔ4441, 1468Ɛ8641, 14ᘔ797ᘔ41, 1621Ɛ1261, 163151361, 1ᘔᘔ222ᘔᘔ1, 400080004, 404090404, 410212014, 4414ᘔ4144, 4456Ɛ6544, 496787694, 963848369 | 10001, 10101, 10201, 10301, 11011, 11111, 11211, 11Ɛ21, 12021, 12121, 1229Ɛ, 1292Ɛ, 12977, 14685, 20002, 20102, 20304, 21012, 21112, 22344, 31053 | 19 |
| ᘔ | 1642662461 | 434ᘔ5 | 1 |
| Ɛ | 10000200001, 10221412201, 10444ᘔ44401, 12102420121, 12345654321, 141Ɛ1Ɛ1Ɛ141, 14404ᘔ40441, 16497679461, 40000800004, 40441ᘔ14404, 41496869414, 44104ᘔ40144, 49635653694 | 100001, 101101, 102201, 110011, 111111, 11Ɛ13Ɛ, 120021, 12ᘔ391, 200002, 201102, 204204, 210012, 223344 | 11 |
| 10 | none | none | 0 |
It is conjectured that if n is divisible by 4, then there are no n-digit palindromic squares.
Rn (where Rn is the repunit with length n) is a palindromic number for n ≤ Ɛ, but not for n ≥ 10 (thus, for all odd number n ≤ 19, ther is n-digit palindromic square 123...321), besides, 11 (also 1{0}1, i.e. 101, 1001, 10001, etc.) is a palindromic number for n ≤ 5, but not for n ≥ 6, and it is conjectured that no palindromic numbers are n-th powers if n ≥ 6.
A cube can end with all digits except 2, 6 and ᘔ (in fact, no perfect powers end with 2, 6 or ᘔ), if n is not congruent to 2 mod 4, then n ends with the same digit as n; if n is congruent to 2 mod 4, then n ends with the digit (the last digit of n +− 6).
The digital root of a cube can be any number.
If k≥2, then n ends with the same digit as n, thus, if i≥2, j≥2 and i and j have the same parity, then n and n end with the same digit.
Squares (and every powers) of 0, 1, 4, 9, 54, 69, 369, 854, 3854, 8369, Ɛ3854, 1Ɛ3854, ᘔ08369, ... end with the same digits as the number itself. (since they are automorphic numbers, from the only four solutions of x−x=0 in the ring of 10-adic numbers, these solutions are 0, 1, ...2Ɛ21Ɛ61Ɛ3854 and ...909ᘔ05ᘔ08369, since 10 is neither a prime not a prime power, the ring of the 10-adic numbers is not a field, thus there are solutions other than 0 and 1 for this equation in 10-adic numbers)
Except for 6 and 24, all even perfect numbers end with 54. Additionally, except for 6, 24 and 354, all even perfect numbers end with 054 or 854. Besides, if any odd perfect number exists, then it must end with 1, 09, 39, 69 or 99.
The digital root of an even perfect number is 1, 4, 6 or ᘔ.
Since 10 is the smallest abundant number, all numbers end with 0 are abundant numbers, besides, all numbers end with 6 except 6 itself are also abundant numbers.
| kn | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ᘔ | Ɛ | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 1ᘔ | 1Ɛ | 20 | Period |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 |
| 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 2 |
| 3 | 1 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 2 |
| 4 | 1 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 1 |
| 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 2 |
| 6 | 1 | 6 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 |
| 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 2 |
| 8 | 1 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 2 |
| 9 | 1 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 1 |
| ᘔ | 1 | ᘔ | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 1 |
| Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | 2 |
| 10 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 |
| 11 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 12 | 1 | 2 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 2 |
| 13 | 1 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 3 | 9 | 2 |
| 14 | 1 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 1 |
| 15 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 2 |
| 16 | 1 | 6 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 |
| 17 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 7 | 1 | 2 |
| 18 | 1 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 | 2 |
| 19 | 1 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 9 | 1 |
| 1ᘔ | 1 | ᘔ | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 1 |
| 1Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | Ɛ | 1 | 2 |
| 20 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 |
The period of the unit digits of powers of a number must be a divisor of 2 (= λ(10), where λ is the Carmichael function).
| n | possible unit digit of an nth power |
|---|---|
| 0 | 1 |
| 1 | any number |
| even number ≥ 2 | 0, 1, 4, 9 (the square digits) |
| odd number ≥ 3 | 0, 1, 3, 4, 5, 7, 8, 9, Ɛ (all digits != 2 mod 4) |
| kn | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ᘔ | Ɛ | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 1ᘔ | 1Ɛ | 20 | Period |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 01 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 1 |
| 1 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 01 | 1 |
| 2 | 01 | 02 | 04 | 08 | 14 | 28 | 54 | ᘔ8 | 94 | 68 | 14 | 28 | 54 | ᘔ8 | 94 | 68 | 14 | 28 | 54 | ᘔ8 | 94 | 68 | 14 | 28 | 54 | 6 |
| 3 | 01 | 03 | 09 | 23 | 69 | 83 | 09 | 23 | 69 | 83 | 09 | 23 | 69 | 83 | 09 | 23 | 69 | 83 | 09 | 23 | 69 | 83 | 09 | 23 | 69 | 4 |
| 4 | 01 | 04 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 3 |
| 5 | 01 | 05 | 21 | ᘔ5 | 41 | 85 | 61 | 65 | 81 | 45 | ᘔ1 | 25 | 01 | 05 | 21 | ᘔ5 | 41 | 85 | 61 | 65 | 81 | 45 | ᘔ1 | 25 | 01 | 10 |
| 6 | 01 | 06 | 30 | 60 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 1 |
| 7 | 01 | 07 | 41 | 47 | 81 | 87 | 01 | 07 | 41 | 47 | 81 | 87 | 01 | 07 | 41 | 47 | 81 | 87 | 01 | 07 | 41 | 47 | 81 | 87 | 01 | 6 |
| 8 | 01 | 08 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 68 | 54 | 2 |
| 9 | 01 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 09 | 69 | 2 |
| ᘔ | 01 | 0ᘔ | 84 | Ɛ4 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 54 | 1 |
| Ɛ | 01 | 0Ɛ | ᘔ1 | 2Ɛ | 81 | 4Ɛ | 61 | 6Ɛ | 41 | 8Ɛ | 21 | ᘔƐ | 01 | 0Ɛ | ᘔ1 | 2Ɛ | 81 | 4Ɛ | 61 | 6Ɛ | 41 | 8Ɛ | 21 | ᘔƐ | 01 | 10 |
| 10 | 01 | 10 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 1 |
| 11 | 01 | 11 | 21 | 31 | 41 | 51 | 61 | 71 | 81 | 91 | ᘔ1 | Ɛ1 | 01 | 11 | 21 | 31 | 41 | 51 | 61 | 71 | 81 | 91 | ᘔ1 | Ɛ1 | 01 | 10 |
| 12 | 01 | 12 | 44 | 08 | 94 | ᘔ8 | 54 | 28 | 14 | 68 | 94 | ᘔ8 | 54 | 28 | 14 | 68 | 94 | ᘔ8 | 54 | 28 | 14 | 68 | 94 | ᘔ8 | 54 | 6 |
| 13 | 01 | 13 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 53 | 69 | 2 |
| 14 | 01 | 14 | 94 | 54 | 14 | 94 | 54 | 14 | 94 | 54 | 14 | 94 | 54 | 14 | 94 | 54 | 14 | 94 | 54 | 14 | 94 | 54 | 14 | 94 | 54 | 3 |
| 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 15 | 01 | 2 |
| 16 | 01 | 16 | 30 | 60 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 1 |
| 17 | 01 | 17 | 61 | 77 | 01 | 17 | 61 | 77 | 01 | 17 | 61 | 77 | 01 | 17 | 61 | 77 | 01 | 17 | 61 | 77 | 01 | 17 | 61 | 77 | 01 | 4 |
| 18 | 01 | 18 | 94 | 68 | 14 | 28 | 54 | ᘔ8 | 94 | 68 | 14 | 28 | 54 | ᘔ8 | 94 | 68 | 14 | 28 | 54 | ᘔ8 | 94 | 68 | 14 | 28 | 54 | 6 |
| 19 | 01 | 19 | 09 | 39 | 69 | 99 | 09 | 39 | 69 | 99 | 09 | 39 | 69 | 99 | 09 | 39 | 69 | 99 | 09 | 39 | 69 | 99 | 09 | 39 | 69 | 4 |
| 1ᘔ | 01 | 1ᘔ | 44 | Ɛ4 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 94 | 14 | 54 | 3 |
| 1Ɛ | 01 | 1Ɛ | 81 | 5Ɛ | 41 | 9Ɛ | 01 | 1Ɛ | 81 | 5Ɛ | 41 | 9Ɛ | 01 | 1Ɛ | 81 | 5Ɛ | 41 | 9Ɛ | 01 | 1Ɛ | 81 | 5Ɛ | 41 | 9Ɛ | 01 | 6 |
| 20 | 01 | 20 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 00 | 1 |
The period of the final two digits of powers of a number must be a divisor of 10 (= λ(100)).
More generally, for every n≥2, the period of the final n digits of powers of a number must be a divisor of 10 (= λ(10)).
| kn | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ᘔ | Ɛ | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 1ᘔ | 1Ɛ | 20 | Period |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 |
| 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 4 | 8 | 5 | ᘔ | 9 | 7 | 3 | 6 | 1 | 2 | 4 | 8 | 5 | ᘔ | 9 | 7 | 3 | 6 | 1 | 2 | 4 | 8 | 5 | ᘔ |
| 3 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 5 |
| 4 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 5 |
| 5 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 5 |
| 6 | 1 | 6 | 3 | 7 | 9 | ᘔ | 5 | 8 | 4 | 2 | 1 | 6 | 3 | 7 | 9 | ᘔ | 5 | 8 | 4 | 2 | 1 | 6 | 3 | 7 | 9 | ᘔ |
| 7 | 1 | 7 | 5 | 2 | 3 | ᘔ | 4 | 6 | 9 | 8 | 1 | 7 | 5 | 2 | 3 | ᘔ | 4 | 6 | 9 | 8 | 1 | 7 | 5 | 2 | 3 | ᘔ |
| 8 | 1 | 8 | 9 | 6 | 4 | ᘔ | 3 | 2 | 5 | 7 | 1 | 8 | 9 | 6 | 4 | ᘔ | 3 | 2 | 5 | 7 | 1 | 8 | 9 | 6 | 4 | ᘔ |
| 9 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 5 |
| ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | 2 |
| Ɛ | 1 | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | 1 |
| 10 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 11 | 1 | 2 | 4 | 8 | 5 | ᘔ | 9 | 7 | 3 | 6 | 1 | 2 | 4 | 8 | 5 | ᘔ | 9 | 7 | 3 | 6 | 1 | 2 | 4 | 8 | 5 | ᘔ |
| 12 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 1 | 3 | 9 | 5 | 4 | 5 |
| 13 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 1 | 4 | 5 | 9 | 3 | 5 |
| 14 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 1 | 5 | 3 | 4 | 9 | 5 |
| 15 | 1 | 6 | 3 | 7 | 9 | ᘔ | 5 | 8 | 4 | 2 | 1 | 6 | 3 | 7 | 9 | ᘔ | 5 | 8 | 4 | 2 | 1 | 6 | 3 | 7 | 9 | ᘔ |
| 16 | 1 | 7 | 5 | 2 | 3 | ᘔ | 4 | 6 | 9 | 8 | 1 | 7 | 5 | 2 | 3 | ᘔ | 4 | 6 | 9 | 8 | 1 | 7 | 5 | 2 | 3 | ᘔ |
| 17 | 1 | 8 | 9 | 6 | 4 | ᘔ | 3 | 2 | 5 | 7 | 1 | 8 | 9 | 6 | 4 | ᘔ | 3 | 2 | 5 | 7 | 1 | 8 | 9 | 6 | 4 | ᘔ |
| 18 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 1 | 9 | 4 | 3 | 5 | 5 |
| 19 | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | ᘔ | 1 | 2 |
| 1ᘔ | 1 | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | Ɛ | 1 |
| 1Ɛ | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 20 | 1 | 2 | 4 | 8 | 5 | ᘔ | 9 | 7 | 3 | 6 | 1 | 2 | 4 | 8 | 5 | ᘔ | 9 | 7 | 3 | 6 | 1 | 2 | 4 | 8 | 5 | ᘔ |
The period of the digital roots of powers of a number must be a divisor of ᘔ (= λ(Ɛ)).
| n | possible digital root of an nth power |
|---|---|
| 0 | 1 |
| = 1, 3, 7, 9 (mod ᘔ) | any number |
| = 2, 4, 6, 8 (mod ᘔ) | 1, 3, 4, 5, 9, Ɛ |
| = 5 (mod ᘔ) | 1, ᘔ, Ɛ |
| > 0 and divisible by ᘔ | 1, Ɛ |
The unit digit of a Fibonacci number can be any digit except 6 (if the unit digit of a Fibonacci number is 0, then the dozens digit of this number must also be 0, thus, all Fibonacci numbers divisible by 6 are also divisible by 100), and the unit digit of a Lucas number cannot be 0 or 9 (thus, no Lucas number is divisible by 10), besides, if a Lucas number ends with 2, then it must end with 0002, i.e., this number is congruent to 2 mod 10.
In the following table, Fn is the n-th Fibonacci number, and Ln is the n-th Lucas number.
| n | Fn | digit root of Fn | Ln | digit root of Ln | n | Fn | digit root of Fn | Ln | digit root of Ln |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 1 | 21 | 37501 | 5 | 81101 | Ɛ |
| 2 | 1 | 1 | 3 | 3 | 22 | 5ᘔ301 | 8 | 111103 | 7 |
| 3 | 2 | 2 | 4 | 4 | 23 | 95802 | 2 | 192204 | 7 |
| 4 | 3 | 3 | 7 | 7 | 24 | 133Ɛ03 | ᘔ | 2ᘔ3307 | 3 |
| 5 | 5 | 5 | Ɛ | Ɛ | 25 | 209705 | 1 | 47550Ɛ | ᘔ |
| 6 | 8 | 8 | 16 | 7 | 26 | 341608 | Ɛ | 758816 | 2 |
| 7 | 11 | 2 | 25 | 7 | 27 | 54Ɛ111 | 1 | 1012125 | 1 |
| 8 | 19 | ᘔ | 3Ɛ | 3 | 28 | 890719 | 1 | 176ᘔ93Ɛ | 3 |
| 9 | 2ᘔ | 1 | 64 | ᘔ | 29 | 121Ɛ82ᘔ | 2 | 2780ᘔ64 | 4 |
| ᘔ | 47 | Ɛ | ᘔ3 | 2 | 2ᘔ | 1ᘔƐ0347 | 3 | 432Ɛ7ᘔ3 | 7 |
| Ɛ | 75 | 1 | 147 | 1 | 2Ɛ | 310ƐƐ75 | 5 | 6ᘔƐ0647 | Ɛ |
| 10 | 100 | 1 | 22ᘔ | 3 | 30 | 5000300 | 8 | Ɛ22022ᘔ | 7 |
| 11 | 175 | 2 | 375 | 4 | 31 | 8110275 | 2 | 16110875 | 7 |
| 12 | 275 | 3 | 5ᘔ3 | 7 | 32 | 11110575 | ᘔ | 25330ᘔᘔ3 | 3 |
| 13 | 42ᘔ | 5 | 958 | Ɛ | 33 | 1922082ᘔ | 1 | 3Ɛ441758 | ᘔ |
| 14 | 6ᘔ3 | 8 | 133Ɛ | 7 | 34 | 2ᘔ3311ᘔ3 | Ɛ | 6477263Ɛ | 2 |
| 15 | Ɛ11 | 2 | 2097 | 7 | 35 | 47551ᘔ11 | 1 | ᘔ3ƐƐ4197 | 1 |
| 16 | 15Ɛ4 | ᘔ | 3416 | 3 | 36 | 75882ƐƐ4 | 1 | 148766816 | 3 |
| 17 | 2505 | 1 | 54Ɛ1 | ᘔ | 37 | 101214ᘔ05 | 2 | 23075ᘔ9Ɛ1 | 4 |
| 18 | 3ᘔƐ9 | Ɛ | 8907 | 2 | 38 | 176ᘔ979Ɛ9 | 3 | 379305607 | 7 |
| 19 | 6402 | 1 | 121Ɛ8 | 1 | 39 | 2780Ɛ0802 | 5 | 5ᘔ9ᘔ643Ɛ8 | Ɛ |
| 1ᘔ | ᘔ2ƐƐ | 1 | 1ᘔƐ03 | 3 | 3ᘔ | 432Ɛ885ƐƐ | 8 | 967169ᘔ03 | 7 |
| 1Ɛ | 14701 | 2 | 310ƐƐ | 4 | 3Ɛ | 6ᘔƐ079201 | 2 | 13550121ƐƐ | 7 |
| 20 | 22ᘔ00 | 3 | 50002 | 7 | 40 | Ɛ22045800 | ᘔ | 2100180002 | 3 |
(Note that F2ᘔ begins with L1ᘔ, and F2Ɛ begins with L1Ɛ)
The period of the digit root of Fibonacci numbers is ᘔ.
The period of the unit digit of Fibonacci numbers is 20, the final two digits is also 20, the final three digits is 200, the final four digits is 2000, ..., the final n digits is 2×10 (n≥2). (see Pisano period)
There are only 13 possible values (of the totally 100 values, thus only 13%) of the final two digits of a Fibonacci number (see OEIS: A066853).
Except 0 = F0 and 1 = F1 = F2, the only square Fibonacci number is 100 = F10 (100 is indeed the square of 10), thus, 10 is the only base such that 100 is a Fibonacci number (since 100 in a base is just the square of this base, and 0 and 1 cannot be the base of numeral system), and thus we can make the near value of the golden ratio: F11/F10 = 175/100 = 1.75 (since the ratio of two connected Fibonacci numbers is close to the golden ratio, as the numbers get large). Besides, the only cube Fibonacci number is 8 = F6.
| n | 2 | n | 2 | n | 2 | n | 2 | n | 2 | n | 2 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 21 | Ɛ2ᘔ20ᘔ8 | 41 | 5317Ɛ5804588ᘔ8 | 61 | 256906ᘔ1ᘔ93096Ɛ8934ᘔ8 | 81 | 11ᘔ12ᘔ743504482569888538ᘔ0ᘔ8 | ᘔ1 | 65933Ɛ8691303ᘔ448Ɛ712227ᘔ7Ɛ11448ᘔ8 |
| 2 | 4 | 22 | 1ᘔ584194 | 42 | ᘔ633ᘔƐ408Ɛ5594 | 62 | 4Ɛ161183966171Ɛ566994 | 82 | 238259286ᘔ08944Ɛ17554ᘔ758194 | ᘔ2 | 10Ɛ667Ɛ51626078895Ɛ22445393ᘔ2289594 |
| 3 | 8 | 23 | 38Ɛ48368 | 43 | 190679ᘔ815ᘔᘔƐ68 | 63 | 9ᘔ302347710323ᘔƐ11768 | 83 | 4744Ɛ6551815689ᘔ32ᘔᘔ992Ɛ4368 | ᘔ3 | 21Ɛ113ᘔᘔ305013556Ɛᘔ4488ᘔ76784556Ɛ68 |
| 4 | 14 | 24 | 75ᘔ94714 | 44 | 361137942Ɛ99Ɛ14 | 64 | 1786046932206479ᘔ23314 | 84 | 9289Ɛ0ᘔᘔ342Ɛ15786599765ᘔ8714 | ᘔ4 | 43ᘔ2279860ᘔ026ᘔƐ1Ɛ88955931348ᘔƐ1Ɛ14 |
| 5 | 28 | 25 | 12Ɛ969228 | 45 | 702273685Ɛ77ᘔ28 | 65 | 3350091664410937846628 | 85 | 16557ᘔ198685ᘔ2Ɛ350Ɛ7730Ɛ95228 | ᘔ5 | 878453750180519ᘔ3Ɛ556ᘔƐ6626959ᘔ3ᘔ28 |
| 6 | 54 | 26 | 25Ɛ716454 | 46 | 120452714ƐƐ33854 | 66 | 66ᘔ0163108821673491054 | 86 | 30ᘔƐ3837514Ɛ85ᘔ6ᘔ1Ɛ3261Ɛ6ᘔ454 | ᘔ6 | 15348ᘔ72ᘔ0340ᘔ3787ᘔᘔƐ19Ɛ10516Ɛ787854 |
| 7 | ᘔ8 | 27 | 4ƐƐ2308ᘔ8 | 47 | 2408ᘔ5229Ɛᘔ674ᘔ8 | 67 | 111803062154431269620ᘔ8 | 87 | 619ᘔ7472ᘔ29Ɛ4Ɛ9183ᘔ6503Ɛ188ᘔ8 | ᘔ7 | 2ᘔ695925806818735399ᘔ37ᘔ20ᘔ31Ɛ3534ᘔ8 |
| 8 | 194 | 28 | 9Ɛᘔ461594 | 48 | 48158ᘔ457Ɛ912994 | 68 | 2234061042ᘔ886251704194 | 88 | 103792925857ᘔ9Ɛ634790ᘔ07ᘔ35594 | ᘔ8 | 5916Ɛ64Ɛ41143526ᘔ777873841863ᘔ6ᘔ6994 |
| 9 | 368 | 29 | 17Ɛ8902Ɛ68 | 49 | 942Ɛ588Ɛ3Ɛ625768 | 69 | 446810208595504ᘔ3208368 | 89 | 20736564Ɛ4Ɛ397Ɛ06936181386ᘔƐ68 | ᘔ9 | Ɛ631Ɛ09ᘔ82286ᘔ5193335274835079191768 |
| ᘔ | 714 | 2ᘔ | 33Ɛ5605Ɛ14 | 4ᘔ | 1685ᘔƐ55ᘔ7Ɛ04Ɛ314 | 6ᘔ | 891420414Ɛ6ᘔᘔ0986414714 | 8ᘔ | 41270Ɛ09ᘔ9ᘔ773ᘔ116703427519Ɛ14 | ᘔᘔ | 1Ɛ063ᘔ179445518ᘔ36666ᘔ52946ᘔ136363314 |
| Ɛ | 1228 | 2Ɛ | 67ᘔƐ00Ɛᘔ28 | 4Ɛ | 314Ɛ9ᘔᘔƐ93ᘔ09ᘔ628 | 6Ɛ | 1562840829Ɛ1981750829228 | 8Ɛ | 82521ᘔ179793278231206852ᘔ37ᘔ28 | ᘔƐ | 3ᘔ107833688ᘔᘔ358711118ᘔ56918270706628 |
| 10 | 2454 | 30 | 1139ᘔ01Ɛ854 | 50 | 629Ɛ799Ɛ678179054 | 70 | 2Ɛ05481457ᘔ37432ᘔ1456454 | 90 | 144ᘔ4383373665344624114ᘔ5873854 | Ɛ0 | 78213467155986Ɛ52222358Ɛ1634521211054 |
| 11 | 48ᘔ8 | 31 | 2277803Ɛ4ᘔ8 | 51 | 1057Ɛ377Ɛ1343360ᘔ8 | 71 | 5ᘔ0ᘔ9428Ɛ3872865828Ɛ08ᘔ8 | 91 | 2898874672710ᘔ6890482298Ɛ5274ᘔ8 | Ɛ1 | 1344269122ᘔƐ751ᘔᘔ44446Ɛ5ᘔ3068ᘔ424220ᘔ8 |
| 12 | 9594 | 32 | 4533407ᘔ994 | 52 | 20Ɛ3ᘔ733ᘔ268670194 | 72 | Ɛ8196855ᘔ752550Ɛ455ᘔ1594 | 92 | 557552912522191560944575ᘔᘔ52994 | Ɛ2 | 26885162459Ɛ2ᘔ39888891ᘔƐ86115884844194 |
| 13 | 16Ɛ68 | 33 | 8ᘔ668139768 | 53 | 41ᘔ792678515120368 | 73 | 1Ɛ43714ᘔƐ92ᘔ4ᘔᘔ1ᘔ8ᘔƐ82Ɛ68 | 93 | ᘔƐ2ᘔᘔ5624ᘔ44362Ɛ01688Ɛ2Ɛ98ᘔ5768 | Ɛ3 | 5154ᘔ3048Ɛ7ᘔ58775555639Ɛ5022Ɛ549488368 |
| 14 | 31Ɛ14 | 34 | 159114277314 | 54 | 839365134ᘔ2ᘔ240714 | 74 | 3ᘔ872299Ɛ6589983959Ɛ45Ɛ14 | 94 | 19ᘔ598Ɛ049888705ᘔ03155ᘔ5Ɛ758Ɛ314 | Ɛ4 | ᘔ2ᘔ986095Ɛ38Ɛ532ᘔᘔᘔƐ077ᘔᘔ045ᘔᘔ96954714 |
| 15 | 63ᘔ28 | 35 | 2Ɛ6228532628 | 55 | 147670ᘔ269858481228 | 75 | 79524577Ɛ0Ɛ577476Ɛ7ᘔ8Ɛᘔ28 | 95 | 378Ɛ75ᘔ09755520Ɛ8062ᘔƐ8ƐƐ2Ɛ5ᘔ628 | Ɛ5 | 185975016Ɛᘔ75ᘔᘔ65999ᘔ1339808Ɛ99716ᘔ9228 |
| 16 | 107854 | 36 | 5Ɛ0454ᘔ65054 | 56 | 29312185174Ɛ4942454 | 76 | 136ᘔ48Ɛ33ᘔ1ᘔƐ32931Ɛ395Ɛ854 | 96 | 735Ɛ2Ɛ8172ᘔᘔᘔ41Ɛ41059Ɛ5Ɛᘔ5ᘔƐ9054 | Ɛ6 | 34Ɛ72ᘔ031Ɛ92Ɛ990Ɛ77782677415Ɛ7723196454 |
| 17 | 2134ᘔ8 | 37 | Ɛᘔ08ᘔ990ᘔ0ᘔ8 | 57 | 5662434ᘔ329ᘔ96848ᘔ8 | 77 | 271895ᘔ67839ᘔ65663ᘔ76ƐƐ4ᘔ8 | 97 | 126Ɛᘔ5Ɛ432599883ᘔ820Ɛ7ᘔƐƐ8Ɛ9Ɛ60ᘔ8 | Ɛ7 | 69Ɛ258063Ɛ65Ɛ761Ɛ3334513282ƐƐ32463708ᘔ8 |
| 18 | 426994 | 38 | 1Ɛ81597618194 | 58 | Ɛ104869865797149594 | 78 | 52356Ɛ91347790Ɛ107931Ɛᘔ994 | 98 | 251Ɛ8Ɛᘔ864Ɛ775479441Ɛ39ƐƐ5Ɛ7Ɛ0194 | Ɛ8 | 117ᘔ4Ɛ4107Ɛ0ƐƐ303ᘔ6668ᘔ26545Ɛᘔ6490721594 |
| 19 | 851768 | 39 | 3Ɛ42Ɛ73034368 | 59 | 1ᘔ20951750Ɛ372296Ɛ68 | 79 | ᘔ46Ɛ1Ɛ62693361ᘔ213663Ɛ9768 | 99 | 4ᘔ3Ɛ5Ɛ9509Ɛ32ᘔ936883ᘔ77ƐᘔƐƐ3ᘔ0368 | Ɛ9 | 23389ᘔ8213ᘔ1Ɛᘔ60791115850ᘔ8ƐƐ90961242Ɛ68 |
| 1ᘔ | 14ᘔ3314 | 3ᘔ | 7ᘔ85Ɛ26068714 | 5ᘔ | 38416ᘔ32ᘔ1ᘔ724571Ɛ14 | 7ᘔ | 1891ᘔ3Ɛ051667038427107Ɛ7314 | 9ᘔ | 987ᘔƐƐ6ᘔ17ᘔ659671547933Ɛ9Ɛᘔ780714 | Ɛᘔ | 467579442783Ɛ90136222Ɛ4ᘔ195ƐƐ61702485Ɛ14 |
| 1Ɛ | 2986628 | 3Ɛ | 1394Ɛᘔ50115228 | 5Ɛ | 74831865839248Ɛ23ᘔ28 | 7Ɛ | 356387ᘔ0ᘔ3112074852213Ɛ2628 | 9Ɛ | 17539ƐƐ183390Ɛ7122ᘔ93667Ɛ7Ɛ9341228 | ƐƐ | 912Ɛ36885347Ɛ60270445ᘔ9836ƐƐƐ0320494Ɛᘔ28 |
| 20 | 5751054 | 40 | 2769Ɛ8ᘔ022ᘔ454 | 60 | 12946350Ɛ476495ᘔ47854 | 80 | 6Ɛ075381862241294ᘔ4427ᘔ5054 | ᘔ0 | 32ᘔ77Ɛᘔ346761Ɛ2245967113Ɛ3Ɛ6682454 | 100 | 1625ᘔ7154ᘔ693Ɛ0052088Ɛ97471ƐƐᘔ0640969Ɛ854 |
The smallest power of 2 starts with the digit Ɛ is 2 = Ɛ2ᘔ20ᘔ8. For all digits 1≤d≤Ɛ, there exists 0≤n≤21 such that 2 starts with the digit d.
2 = 59Ɛ18922Ɛ81631ᘔ39875663Ɛ89ᘔ853ᘔ91Ɛ595336ᘔ6114815ᘔ5ᘔ6929933ᘔ288Ɛ774Ɛ479575ᘔ628 may be the largest power of 2 not contain the digit 0, it has 65 digits. (Note that in the decimal (base ᘔ) system, the largest power of 2 not contain the digit 0 is 2 = Ɛ8196855ᘔ752550Ɛ455ᘔ1594 = 77371252455336267181195264ᘔ, it has only 22 digits in base ᘔ)
The number 2 = 2 (see power of 2#Powers of two whose exponents are powers of two) is very close to googol (10), since it has ƐƐ digits. (thus, the Fermat number F9 (=2+1) is very close to googol)
1001 is the first four-digit palindromic number, and it is also the smallest number expressible as the sum of two cubes in two different ways, i.e. 1001 = 1 + 1000 (=1 + 10) = 509 + 6Ɛ4 (=9 + ᘔ) (see taxicab number for other numbers), and it is also the smallest absolute Euler pseudoprime, note that there is no absolute Euler-Jacobi pseudoprime and no absolute strong pseudoprime. Since 1001 = 7×11×17, we can use the divisibility rule of 1001 (i.e. form the alternating sum of blocks of three from right to left) for the divisibility rule of 7, 11 and 17. Besides, if 6k+1, 10k+1 and 16k+1 are all primes, then the product of them must be a Carmichael number (absolute Fermat pseudoprime), the smallest case is indeed 1001 (for k = 1), but 1001 is not the smallest Carmichael number (the smallest Carmichael number is 3ᘔ9).
is very close to 1.5, since a near-value for is 15/10 (=N4/P4, where Nn is nth NSW number, and Pn is nth Pell number, Nn/Pn is very close to when n is large). Besides, is very close to 2.2ᘔ, since a near-value for is 22ᘔ/100 (=L10/F10, where Ln is nth Lucas number, and Fn is nth Fibonacci number, Ln/Fn is very close to when n is large).
The reciprocal of n is terminating number if and only if n is 3-smooth, the 3-smooth numbers up to 1000 are 1, 2, 3, 4, 6, 8, 9, 10, 14, 16, 20, 23, 28, 30, 40, 46, 54, 60, 69, 80, 90, ᘔ8, 100, 116, 140, 160, 183, 194, 200, 230, 280, 300, 346, 368, 400, 460, 509, 540, 600, 690, 714, 800, 900, ᘔ16, ᘔ80, 1000.
If and only if n is a divisor of 20, then m = 1 mod n for every integer m coprime to n.
If and only if n is a divisor of 20, then all Dirichlet characters for n are all real.
If and only if n is a divisor of 20, then n is divisible by all numbers less than or equal to the square root of n.
If and only if n+1 is a divisor of 20, then is squarefree for all 0 ≤ k ≤ n.
All prime numbers end with prime digits or 1 (i.e. end with 1, 2, 3, 5, 7 or Ɛ), more generally, except for 2 and 3, all prime numbers end with 1, 5, 7 or Ɛ (1 and all prime digits that do not divide 10), since all prime numbers other than 2 and 3 are coprime to 10.
The density of primes end with 1 is a relatively low, but the density of primes end with 5, 7 and Ɛ are nearly equal. (since all prime squares except 4 and 9 end with 1, no prime squares end with 5, 7 or Ɛ)
Except (3, 5), all twin primes end with (5, 7) or (Ɛ, 1).
If n ≥ 3 and n is not divisible by Ɛ, then there are infinitely many primes with digit sum n.
All palindromic primes except 11 has an odd number of digits, since all even-digit palindromic numbers are divisible by 11. The palindromic primes below 1000 are 2, 3, 5, 7, Ɛ, 11, 111, 131, 141, 171, 181, 1Ɛ1, 535, 545, 565, 575, 585, 5Ɛ5, 727, 737, 747, 767, 797, Ɛ1Ɛ, Ɛ2Ɛ, Ɛ6Ɛ.
All lucky numbers end with digit 1, 3, 7 or 9.
Except for 3, all Fermat primes end with 5.
Except for 3, all Mersenne primes end with 7.
Except for 2 and 3, all Sophie Germain primes end with 5 or Ɛ.
Except for 5 and 7, all safe primes end with Ɛ.
A prime p is Gaussian prime (prime in the ring , where ) if and only if p ends with 7 or Ɛ (or p=3).
A prime p is Eisenstein prime (prime in the ring , where ) if and only if p ends with 5 or Ɛ (or p=2).
A prime p can be written as x + y if and only if p ends with 1 or 5 (or p=2).
A prime p can be written as x + 3y if and only if p ends with 1 or 7 (or p=3).
All full reptend primes end with 5 or 7. (in fact, for all primes p ≥ 5, (p-1)/(the period length of 1/p) is odd if and only if p is end with 5 or 7, since 10 is a quadratic nonresidue mod p (i.e. , where is the Legendre symbol) if and only if p is end with 5 or 7, by quadratic reciprocity, and if 10 is a quadratic residue mod a prime, then 10 cannot be a primitive root mod this prime) However, the converse is not true, 17 is not a full reptend prime, since the recurring digits of 1/17 is 0.076Ɛ45076Ɛ45..., which has only period 6. If and only if p is a full reptend prime, then the recurring digits of 1/p is cyclic number, e.g. the recurring digits of 1/5 is the cyclic number 2497 (the cyclic permutations of the digits are this number multiplied by 1 to 4), and the recurring digits of 1/7 is the cyclic number 186ᘔ35 (the cyclic permutations of the digits are this number multiplied by 1 to 6). The full reptend primes below 1000 are 5, 7, 15, 27, 35, 37, 45, 57, 85, 87, 95, ᘔ7, Ɛ5, Ɛ7, 105, 107, 117, 125, 145, 167, 195, 1ᘔ5, 1Ɛ5, 1Ɛ7, 205, 225, 255, 267, 277, 285, 295, 315, 325, 365, 377, 397, 3ᘔ5, 3Ɛ5, 3Ɛ7, 415, 427, 435, 437, 447, 455, 465, 497, 4ᘔ5, 517, 527, 535, 545, 557, 565, 575, 585, 5Ɛ5, 615, 655, 675, 687, 695, 6ᘔ7, 705, 735, 737, 745, 767, 775, 785, 797, 817, 825, 835, 855, 865, 8Ɛ5, 8Ɛ7, 907, 927, 955, 965, 995, 9ᘔ7, 9Ɛ5, ᘔ07, ᘔ17, ᘔ35, ᘔ37, ᘔ45, ᘔ77, ᘔ87, ᘔ95, ᘔƐ7, Ɛ25, Ɛ37, Ɛ45, Ɛ95, Ɛ97, Ɛᘔ5, ƐƐ5, ƐƐ7. (Note that for the primes end with 5 or 7 below 30 (5, 7, 15, 17, 25 and 27, all numbers end with 5 or 7 below 30 are primes), 5, 7, 15 and 27 are full reptend primes, and since 5×25 = 101 = , the period of 25 is 4, which is the same as the period of 5, and we can use the test of the divisiblity of 5 to test that of 25 (form the alternating sum of blocks of two from right to left), and since 7×17 = Ɛ1 = , the period of 17 is 6, which is the same as the period of 7, and we can use the test of the divisiblity of 7 to test that of 17 (form the alternating sum of blocks of three from right to left), thus, 17 and 25 are not full reptend primes, and they are the only two non-full reptend primes end with 5 or 7 below 30)
By Midy theorem, if p is a prime with even period length (let its period length be n), then if we let , then ai + ai+n/2 = Ɛ for every 1 ≤ i ≤ n/2. e.g. 1/5 = 0.249724972497..., and 24 + 97 = ƐƐ, and 1/7 = 0.186ᘔ35186ᘔ35..., and 186 + ᘔ35 = ƐƐƐ, all primes (other than 2 and 3) ≤ 37 except Ɛ, 1Ɛ and 31 have even period length, thus they can use Midy theorem to get an Ɛ-repdigit number, the length of this number is the period length of this prime. (see below for the recurring digits for 1/n for all n ≤ 30)
The unique primes below 10 are Ɛ, 11, 111, Ɛ0Ɛ1, ƐƐ01, 11111, 24727225, Ɛ0Ɛ0Ɛ0Ɛ0Ɛ1, Ɛ00Ɛ00ƐƐ0ƐƐ1, 100ƐƐƐᘔƐᘔƐƐ000101, 1111111111111111111, ƐƐƐƐ0000ƐƐƐƐ0000ƐƐƐƐ0001, 100ƐƐƐᘔƐƐ0000ƐƐƐᘔƐƐ000101, 10ƐƐƐᘔᘔᘔƐ011110ƐᘔᘔᘔƐ00011, ƐƐƐƐƐƐƐƐ00000000ƐƐƐƐƐƐƐƐ00000001, ƐƐƐ000000ƐƐƐ000000ƐƐƐƐƐƐ000ƐƐƐƐƐƐ001, and the period length of their reciprocals are 1, 2, 3, ᘔ, 10, 5, 18, 1ᘔ, 19, 50, 17, 48, 70, 5ᘔ, 68, 53.
If p is a safe prime other than 5, 7 and Ɛ, then the period length of 1/p is (p-1)/2. (this is not true for all primes ends with Ɛ (other than Ɛ itself), the first counterexample is p = 2ƐƐ, where the period length of 1/p is only 37)
There is no full reptend prime ends with 1, since 10 is quadratic residue for all primes end with 1. (In contrast, in decimal (base ᘔ) system there are some such primes, and may be infinitely many such primes, the first few such primes in that base are 61ᘔ = 51, 131ᘔ = ᘔƐ, 181ᘔ = 131, 461ᘔ = 325, 491ᘔ = 34Ɛ, see OEIS: A073761) (if so, then this prime p is a proper prime (i.e. for the reciprocal of such primes (1/p), each digit 0, 1, 2, ..., Ɛ appears in the repeating sequence the same number of times as does each other digit (namely, (p−1)/10 times)), see repeating decimal#Fractions with prime denominators) (In fact, not only for base 10 such primes do not exist, for all bases = 0 mod 4 (i.e. bases end with digit 0, 4 or 8), such primes do not exist)
5 and 7 are the only two safe primes which are also full reptend primes, since except 5 and 7, all safe primes end with Ɛ, and 10 is quadratic residue for all primes end with Ɛ. (In contrast, in decimal (base ᘔ) system there may be infinitely many such primes, the first few such primes in that base are 7ᘔ = 7, 23ᘔ = 1Ɛ, 47ᘔ = 3Ɛ, 59ᘔ = 4Ɛ, 167ᘔ = 11Ɛ, see OEIS: A000353) (if so, then this prime p produces a stream of p−1 pseudo-random digits, see repeating decimal#Fractions with prime denominators) (In fact, not only for base 10 there are only finitely many such primes, of course for square bases (bases of the form k) only 2 may be full reptend prime (if the base is odd), and all odd primes are not full reptend primes, but since all safe primes are odd primes, for these bases such primes do not exist, besides, for the bases of the form 3k, only 5 and 7 can be such primes, the proof for these bases is completely the same as that for base 10)
| p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p | p | period length of 1/p |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 0 | 111 | 3 | 267 | 266 | 41Ɛ | 20Ɛ | 591 | 3ᘔ | 767 | 766 | 927 | 926 | Ɛ1Ɛ | 56Ɛ | 1107 | 1106 | 12Ɛ5 | 12Ɛ4 | 14Ɛ1 | 14Ɛ | 16ᘔ7 | 16ᘔ6 |
| 3 | 0 | 117 | 116 | 271 | 27 | 421 | 63 | 59Ɛ | 2ᘔƐ | 76Ɛ | 395 | 955 | 954 | Ɛ21 | 570 | 1115 | 1114 | 1301 | 760 | 14Ɛ5 | 14Ɛ4 | 16Ɛ5 | 188 |
| 5 | 4 | 11Ɛ | 6Ɛ | 277 | 276 | 427 | 426 | 5Ɛ1 | 159 | 771 | 132 | 95Ɛ | 48Ɛ | Ɛ25 | Ɛ24 | 1125 | 1124 | 1317 | 506 | 14ƐƐ | 85Ɛ | 16Ɛ7 | 16Ɛ6 |
| 7 | 6 | 125 | 124 | 27Ɛ | 13Ɛ | 431 | 109 | 5Ɛ5 | 5Ɛ4 | 775 | 774 | 965 | 964 | Ɛ2Ɛ | 575 | 112Ɛ | 675 | 1337 | 512 | 150Ɛ | 865 | 1705 | 398 |
| Ɛ | 1 | 12Ɛ | 75 | 285 | 284 | 435 | 434 | 5Ɛ7 | 66 | 77Ɛ | 39Ɛ | 971 | 172 | Ɛ31 | 116 | 1135 | 1134 | 133Ɛ | 77Ɛ | 1517 | 1ᘔᘔ | 1711 | 493 |
| 11 | 2 | 131 | 76 | 291 | 83 | 437 | 436 | 5ƐƐ | 2ƐƐ | 785 | 784 | 987 | 32ᘔ | Ɛ37 | Ɛ36 | 114Ɛ | 685 | 1345 | 1344 | 1521 | 436 | 1715 | 1714 |
| 15 | 14 | 13Ɛ | 7Ɛ | 295 | 294 | 447 | 446 | 611 | 163 | 791 | 3ᘔ6 | 995 | 994 | Ɛ45 | Ɛ44 | 1151 | 115 | 1351 | 166 | 1525 | 1524 | 1727 | 1726 |
| 17 | 6 | 141 | 20 | 2ᘔ1 | 150 | 455 | 454 | 615 | 614 | 797 | 796 | 9ᘔ7 | 9ᘔ6 | Ɛ61 | 16 | 1165 | 1164 | 1365 | 1364 | 1547 | 1Ɛ2 | 1735 | 1734 |
| 1Ɛ | Ɛ | 145 | 144 | 2ᘔƐ | 155 | 457 | 15ᘔ | 617 | 206 | 7ᘔ1 | 138 | 9ᘔƐ | 4Ɛ5 | Ɛ67 | 3ᘔ2 | 1167 | 42 | 1367 | 1366 | 1561 | 89 | 1745 | 1744 |
| 25 | 4 | 147 | 56 | 2Ɛ1 | 26 | 45Ɛ | 22Ɛ | 61Ɛ | 30Ɛ | 7ƐƐ | 3ƐƐ | 9Ɛ1 | 9Ɛ | Ɛ6Ɛ | 595 | 1185 | 1184 | 136Ɛ | 795 | 156Ɛ | 97 | 1747 | 1746 |
| 27 | 26 | 157 | 12 | 2ƐƐ | 37 | 465 | 464 | 637 | 212 | 801 | 140 | 9Ɛ5 | 9Ɛ4 | Ɛ71 | 596 | 118Ɛ | 6ᘔ5 | 1377 | 106 | 1577 | 1576 | 1751 | 32ᘔ |
| 31 | 9 | 167 | 166 | 301 | 90 | 46Ɛ | 7 | 63Ɛ | 31Ɛ | 80Ɛ | 405 | 9ƐƐ | 4ƐƐ | Ɛ91 | 2Ɛ3 | 1197 | 472 | 138Ɛ | 7ᘔ5 | 157Ɛ | 89Ɛ | 1755 | 1754 |
| 35 | 34 | 16Ɛ | 95 | 307 | 102 | 471 | 13 | 647 | 216 | 817 | 816 | ᘔ07 | ᘔ06 | Ɛ95 | Ɛ94 | 11ᘔ1 | 6Ɛ | 1391 | 3Ɛ3 | 1585 | 1584 | 1757 | 1756 |
| 37 | 36 | 171 | 96 | 30Ɛ | 165 | 481 | 24 | 655 | 654 | 825 | 824 | ᘔ0Ɛ | 505 | Ɛ97 | Ɛ96 | 11ᘔ5 | 11ᘔ4 | 1395 | 1394 | 1587 | 2ᘔ | 176Ɛ | 995 |
| 3Ɛ | 1Ɛ | 175 | 8 | 315 | 314 | 485 | 44 | 661 | 176 | 82Ɛ | 415 | ᘔ11 | 56 | Ɛᘔ5 | Ɛᘔ4 | 11ᘔ7 | 11ᘔ6 | 13ᘔ1 | 7Ɛ0 | 1591 | 2Ɛ6 | 1781 | 4Ɛ0 |
| 45 | 44 | 17Ɛ | 9Ɛ | 321 | 170 | 48Ɛ | 245 | 665 | 138 | 835 | 834 | ᘔ17 | ᘔ16 | ƐƐ5 | ƐƐ4 | 11ᘔƐ | 6Ɛ5 | 13ᘔ7 | 536 | 15ᘔƐ | 8Ɛ5 | 1785 | 1784 |
| 4Ɛ | 25 | 181 | ᘔ0 | 325 | 324 | 497 | 496 | 66Ɛ | 335 | 841 | 84 | ᘔ27 | 156 | ƐƐ7 | ƐƐ6 | 11Ɛ7 | 11Ɛ6 | 13Ɛ1 | 13Ɛ | 15ƐƐ | 8ƐƐ | 178Ɛ | 9ᘔ5 |
| 51 | 13 | 18Ɛ | ᘔ5 | 327 | 10ᘔ | 4ᘔ5 | 4ᘔ4 | 675 | 674 | 851 | 14ᘔ | ᘔ35 | ᘔ34 | 1005 | 1004 | 1201 | 700 | 13Ɛ5 | 13Ɛ4 | 1601 | 160 | 1797 | 1796 |
| 57 | 56 | 195 | 194 | 32Ɛ | 175 | 4Ɛ1 | 9ᘔ | 687 | 686 | 855 | 854 | ᘔ37 | ᘔ36 | 1011 | 73 | 120Ɛ | 705 | 1405 | 1404 | 1615 | 1614 | 17ᘔ1 | 9Ɛ0 |
| 5Ɛ | 2Ɛ | 19Ɛ | ᘔƐ | 33Ɛ | 17Ɛ | 4ƐƐ | 25Ɛ | 68Ɛ | 345 | 85Ɛ | 42Ɛ | ᘔ3Ɛ | 51Ɛ | 1017 | 1016 | 1211 | 706 | 1407 | 326 | 1621 | 910 | 17ᘔ5 | 17ᘔ4 |
| 61 | 30 | 1ᘔ5 | 1ᘔ4 | 347 | 46 | 507 | 182 | 695 | 694 | 865 | 864 | ᘔ41 | 188 | 1021 | 610 | 121Ɛ | 70Ɛ | 1425 | 1424 | 1625 | 1624 | 17ƐƐ | 9ƐƐ |
| 67 | 22 | 1ᘔ7 | 46 | 34Ɛ | 2Ɛ | 511 | 266 | 69Ɛ | 34Ɛ | 867 | 2ᘔ2 | ᘔ45 | ᘔ44 | 1027 | 1026 | 1231 | 123 | 142Ɛ | 815 | 1635 | 274 | 1807 | 682 |
| 6Ɛ | 35 | 1Ɛ1 | Ɛ6 | 357 | 11ᘔ | 517 | 516 | 6ᘔ7 | 6ᘔ6 | 871 | 152 | ᘔ4Ɛ | 525 | 1041 | 620 | 123Ɛ | 71Ɛ | 1431 | 286 | 1647 | 276 | 1815 | 1814 |
| 75 | 8 | 1Ɛ5 | 1Ɛ4 | 35Ɛ | 18Ɛ | 51Ɛ | 45 | 6Ɛ1 | 6Ɛ | 881 | 440 | ᘔ5Ɛ | 52Ɛ | 1047 | 1046 | 1245 | 1244 | 1437 | 1436 | 1655 | 1654 | 181Ɛ | ᘔ0Ɛ |
| 81 | 14 | 1Ɛ7 | 1Ɛ6 | 365 | 364 | 527 | 526 | 701 | 360 | 88Ɛ | 445 | ᘔ6Ɛ | 535 | 104Ɛ | 625 | 1255 | 114 | 143Ɛ | 81Ɛ | 1657 | 61ᘔ | 1825 | 1824 |
| 85 | 84 | 205 | 204 | 375 | 34 | 531 | 276 | 705 | 704 | 8ᘔ5 | 98 | ᘔ77 | ᘔ76 | 1051 | 313 | 1257 | 49ᘔ | 1445 | 1444 | 165Ɛ | 92Ɛ | 1831 | 509 |
| 87 | 86 | 217 | 86 | 377 | 376 | 535 | 534 | 70Ɛ | 365 | 8ᘔ7 | 2Ɛ6 | ᘔ87 | ᘔ86 | 1061 | 16 | 125Ɛ | 72Ɛ | 1457 | 1456 | 1667 | 622 | 183Ɛ | ᘔ1Ɛ |
| 8Ɛ | 45 | 21Ɛ | 10Ɛ | 391 | 1ᘔ6 | 541 | 54 | 711 | 71 | 8ᘔƐ | 455 | ᘔ91 | 283 | 106Ɛ | 635 | 1261 | 730 | 1461 | 38 | 1671 | 936 | 184Ɛ | ᘔ25 |
| 91 | 46 | 221 | 66 | 397 | 396 | 545 | 544 | 71Ɛ | 36Ɛ | 8Ɛ5 | 8Ɛ4 | ᘔ95 | ᘔ94 | 107Ɛ | 63Ɛ | 126Ɛ | 735 | 1465 | 1464 | 1677 | 20ᘔ | 1861 | 269 |
| 95 | 94 | 225 | 224 | 3ᘔ5 | 3ᘔ4 | 557 | 556 | 721 | 370 | 8Ɛ7 | 8Ɛ6 | ᘔ9Ɛ | 54Ɛ | 1087 | 1086 | 127Ɛ | 73Ɛ | 1467 | 562 | 167Ɛ | 93Ɛ | 1865 | 1864 |
| ᘔ7 | ᘔ6 | 237 | 92 | 3ᘔƐ | 1Ɛ5 | 565 | 564 | 727 | 24ᘔ | 901 | 230 | ᘔᘔ7 | 376 | 109Ɛ | 64Ɛ | 1281 | 740 | 1471 | 419 | 1681 | 140 | 186Ɛ | ᘔ35 |
| ᘔƐ | 55 | 241 | 120 | 3Ɛ5 | 3Ɛ4 | 575 | 574 | 735 | 734 | 905 | 198 | ᘔᘔƐ | 555 | 10Ɛ1 | 329 | 1295 | 94 | 1475 | 1474 | 1685 | 1684 | 1875 | 1874 |
| Ɛ5 | Ɛ4 | 24Ɛ | 125 | 3Ɛ7 | 3Ɛ6 | 577 | 116 | 737 | 736 | 907 | 906 | ᘔƐ7 | ᘔƐ6 | 10Ɛ7 | 10Ɛ6 | 1297 | 1296 | 147Ɛ | 83Ɛ | 168Ɛ | 945 | 1877 | 146 |
| Ɛ7 | Ɛ6 | 251 | 73 | 401 | 100 | 585 | 584 | 745 | 744 | 90Ɛ | 465 | ᘔƐƐ | 55Ɛ | 10ƐƐ | 65Ɛ | 12ᘔ1 | 75 | 148Ɛ | 845 | 1697 | 1696 | 189Ɛ | ᘔ4Ɛ |
| 105 | 104 | 255 | 254 | 40Ɛ | 205 | 587 | 1ᘔᘔ | 747 | 9ᘔ | 91Ɛ | 46Ɛ | Ɛ11 | 1ᘔ2 | 1101 | 220 | 12ᘔ5 | 2Ɛ8 | 1495 | 1494 | 169Ɛ | 94Ɛ | 18ᘔ1 | 210 |
| 107 | 106 | 25Ɛ | 12Ɛ | 415 | 414 | 58Ɛ | 2ᘔ5 | 751 | 1ᘔ3 | 921 | 236 | Ɛ15 | 228 | 1105 | 1104 | 12ᘔ7 | 12ᘔ6 | 149Ɛ | 84Ɛ | 16ᘔ1 | 950 | 18ᘔƐ | ᘔ55 |
| period length | primes | period length | primes |
|---|---|---|---|
| 1 | Ɛ | 11 | 1Ɛ0411, 69ᘔ3901 |
| 2 | 11 | 12 | 157, 7687 |
| 3 | 111 | 13 | 51, 471, 57Ɛ1 |
| 4 | 5, 25 | 14 | 15, 81, 106ᘔ95 |
| 5 | 11111 | 15 | ᘔ9ᘔ9ᘔƐ, 126180ƐƐ0ƐƐ |
| 6 | 7, 17 | 16 | Ɛ61, 1061 |
| 7 | 46Ɛ, 2ᘔ3Ɛ | 17 | 1111111111111111111 |
| 8 | 75, 175 | 18 | 24727225 |
| 9 | 31, 3ᘔ891 | 19 | Ɛ00Ɛ00ƐƐ0ƐƐ1 |
| ᘔ | Ɛ0Ɛ1 | 1ᘔ | Ɛ0Ɛ0Ɛ0Ɛ0Ɛ1 |
| Ɛ | 1Ɛ, 754Ɛ2Ɛ41 | 1Ɛ | 3Ɛ, 78935Ɛᘔ441, 523074ᘔ3ᘔᘔƐ |
| 10 | ƐƐ01 | 20 | 141, 8Ɛ5281 |
The period level of a prime p ≥ 5 is (p−1)/(period length of 1/p), e.g., has period level 3, thus the numbers with integer 1 ≤ a ≤ 16 from 3 different cycles: 076Ɛ45 (for a = 1, 7, 8, Ɛ, 10, 16), 131ᘔ8ᘔ (for a = 2, 3, 5, 12, 14, 15) and 263958 (for a = 4, 6, 9, ᘔ, 11, 13). Besides, has period level 1, thus this number is a cyclic number and 15 is a full-reptend prime, and all of the numbers with integer 1 ≤ a ≤ 14 from the cycle 08579214Ɛ36429ᘔ7.
There are only 9 repunit primes below R1000: R2, R3, R5, R17, R81, R91, R225, R255 and R4ᘔ5 (Rn is the repunit with length n). If p is a Sophie Germain prime other than 2, 3 and 5, then Rp is divisible by 2p+1, thus Rp is not prime. (The length for the repunit (probable) primes are 2, 3, 5, 17, 81, 91, 225, 255, 4ᘔ5, 5777, 879Ɛ, 198Ɛ1, 23175, 311407, ..., note that 879Ɛ is the smallest (and the only known) such number ends with Ɛ)
By Fermat's little theorem, if p is a prime other than 2, 3 and Ɛ, then p divides the repunit with length p−1. (The converse is not true, the first counterexample is 55, which is composite (equals 5×11) but divides the repunit with length 54, the counterexamples up to 1000 are 55, 77, Ɛ1, 101, 187, 275, 4ᘔ7, 777, 781, Ɛ55, they are exactly the Fermat pseudoprimes for base 10 (composite numbers c such that 10 = 1 mod c) which are not divisible by Ɛ, they are called "deceptive primes", if n is deceptive prime, then Rn is also deceptive prime, thus there are infinitely may deceptive primes) Thus, we can prove that every positive integer coprime to 10 has a repunit multiple, and every positive integer has a multiple uses only 0's and 1's.
| n | +1 | +2 | +3 | +4 | +5 | +6 | +7 | +8 | +9 | +ᘔ | +Ɛ | +10 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0+ | 1 | 10 | 10 | 10 | 101 | 10 | 1001 | 100 | 100 | 1010 | 11111111111 | 10 |
| 10+ | 11 | 10010 | 1010 | 100 | 10111 | 100 | 1001 | 1010 | 10010 | 111111111110 | 11101 | 100 |
| 20+ | 110111 | 110 | 1000 | 10010 | 101 | 1010 | 101011 | 1000 | 111111111110 | 101110 | 101101 | 100 |
| 30+ | 1001001 | 10010 | 110 | 10100 | 111001 | 10010 | 101001 | 111111111110 | 10100 | 111010 | 10001111 | 100 |
| 40+ | 10111101 | 1101110 | 101110 | 110 | 1100101 | 1000 | 1011101111111 | 100100 | 10010 | 1010 | 101011 | 1010 |
| 50+ | 10010101 | 1010110 | 100100 | 1000 | 1111 | 111111111110 | 1100101 | 101110 | 111010 | 1011010 | 1100111 | 100 |
| 60+ | 10101101 | 10010010 | 1101110 | 10010 | 1011101111111 | 110 | 10101011 | 10100 | 10000 | 1110010 | 100111001 | 10010 |
| 70+ | 1101001 | 1010010 | 1010 | 1111111111100 | 10001 | 10100 | 1001 | 111010 | 1010110 | 100011110 | 101101 | 1000 |
| 80+ | 111011 | 101111010 | 1111111111100 | 1101110 | 110001 | 101110 | 10100111 | 1100 | 1011010 | 11001010 | 100111 | 1000 |
| 90+ | 1010111111 | 10111011111110 | 10010010 | 100100 | 10101001 | 10010 | 110101001 | 1010 | 1100 | 1010110 | 101100011 | 10100 |
| ᘔ0+ | 111111111101 | 100101010 | 1110010 | 1010110 | 11100001 | 100100 | 1100001 | 10000 | 1010010 | 11110 | 1111011111 | 111111111110 |
| Ɛ0+ | 1001 | 11001010 | 101000 | 1011100 | 101011 | 111010 | 11010111 | 1011010 | 100011110 | 11001110 | 1111111111111111111111 | 100 |
| n | 1 | 5 | 7 | Ɛ | 11 | 15 | 17 | 1Ɛ | 21 | 25 | 27 | 2Ɛ |
| smallest k such that k×n is a repunit | 1 | 275 | 1ᘔ537 | 123456789Ɛ | 1 | 92ᘔ79Ɛ43715865 | 8327 | 69Ɛ63848Ɛ | 634ᘔ159788253ᘔ72Ɛ1 | 55 | 509867481Ɛ793ᘔᘔ5ᘔ1243628Ɛ317 | 45ᘔ3976ᘔ7Ɛ |
| the length of the repunit k×n | 1 | 4 | 6 | Ɛ | 2 | 14 | 6 | Ɛ | 18 | 4 | 26 | 10 |
(this k is usually not prime, in fact, this k is not prime for all numbers n < 100 which are coprime to 10 except n = 55, and for n < 1000 which is coprime to 10, this k is prime only for n = 55, 101, 19Ɛ, 275 and 46Ɛ, and only 19Ɛ and 46Ɛ are itself prime, other 3 numbers are 5×11, 5×25 and 11×25, and this k for these n are successively 25, 11 and 5, which makes k×n = R4 = 1111 = 5×11×25, besides, this k for n = 46Ɛ is 2ᘔ3Ɛ, which makes k×n = R7 = 1111111, a repunit semiprime, and this k for n = 19Ɛ is a ᘔ8-digit prime number, with k×n = RᘔƐ, another repunit semiprime)
For every prime p except Ɛ, the repunit with length p is congruent to 1 mod p. (The converse is also not true, the counterexamples up to 1000 are 4, 6, 10, 33, 55, 77, Ɛ1, 101, 187, 1Ɛ0, 275, 444, 4ᘔ7, 777, 781, Ɛ55, they are called "repunit pseudoprimes" (or weak deceptive primes), all deceptive primes are also repunit pseudoprimes, if n is repunit pseudoprime, then Rn is also repunit pseudoprime, thus there are infinitely may repunit pseudoprimes. No repunit pseudoprimes are divisible by 8, 9 or Ɛ. (in fact, the repunit pseudoprimes are exactly the weak pseudoprimes for base 10 (composite numbers c such that 10 = 10 mod c) which are not divisible by Ɛ) Besides, the deceptive primes are exactly the repunit pseudoprimes which are coprime to 10)
Smallest multiple of n with digit sum 2 are: (0 if not exist)
- 2, 2, 20, 20, 101, 20, 1001, 20, 200, 1010, 0, 20, 11, 10010, 1010, 200, 100000001, 200, 1001, 1010, 10010, 0, 0, 20, 10000000001, 110, 2000, 10010, 101, 1010, 1000000000000001, 200, 0, 1000000010, 1000000000001, 200, ..., if and only if n is divisible by some prime p with 1/p odd period length, then such number does not exist.
Smallest multiple of n with digit sum 3 are: (0 if not exist)
- 3, 12, 3, 30, 21, 30, 12, 120, 30, 210, 0, 30, 0, 12, 210, 300, 201, 30, 10101, 210, 120, 0, 1010001, 120, 21, 0, 300, 120, 0, 210, 1010001, 1200, 0, 2010, 200001, 30, ..., such number does not exist for n divisible by Ɛ, 11 or 25.
Smallest multiple of n with digit sum 4 are: (0 if not exist)
- 4, 4, 13, 4, 13, 40, 103, 40, 130, 130, 0, 40, 22, 1030, 13, 40, 3001, 130, 2002, 130, 103, 0, 11101, 40, 10012, 22, 1300, 1030, 202, 130, 10003, 400, 0, 30010, 101101, 130, ..., such number is conjectured to exist for all n not divisible by Ɛ (of course, if n is divisible by Ɛ, then such number does not exist).
Smallest multiple of n with digit sum n are:
- 1, 2, 3, 4, 5, 6, 7, 8, 9, ᘔ, Ɛ, 1Ɛ0, 20Ɛ, 22ᘔ, 249, 268, 287, 2ᘔ6, 45ᘔ, 488, 4Ɛ6, 1Ɛᘔ, 8Ɛ4, 3Ɛᘔ0, 3ƐƐ, 23Ɛᘔ, 1899, ᘔᘔ8, 2Ɛ79, 4Ɛ96, 1Ɛᘔ9, 4ᘔᘔ8, 2ƐƐ9, 3ᘔƐᘔ, 799ᘔ, 5ƐƐ90, ..., such number is conjectured to exist for all n.
Write the recurring digits of 1/45 (=0.2872Ɛ3ᘔ23205525ᘔ784640ᘔᘔ4Ɛ9349081989Ɛ6696143757Ɛ117, which has period 44) to 44/45, we get a 44×44 prime reciprocal magic square (its magic number is 1Ɛᘔ), it is conjectured that there are infinitely many such primes, but 45 is the only such prime below 1000, all such primes are full reptend primes, i.e. the reciprocal of them are cyclic numbers, and 10 is a primitive root modulo these primes.
All numbers of the form 34{1} are composite (proof: 34{1n} = 34×10+(10−1)/Ɛ = (309×10−1)/Ɛ and it can be factored to ((19×10−1)/Ɛ) × (19×10+1) for even n and divisible by 11 for odd n). Besides, 34 was proven to be the smallest n such that all numbers of the form n{1} are composite. However, the smallest prime of the form 23{1} is 23{1Ɛ78}, it has Ɛ7ᘔ digits. The only other two n≤100 such that all numbers of the form n{1} are composite are 89 and 99 (the reason of 89 is the same as 34, and the reason of 99 is 99{1n} is divisible by 5, 11 or 25).
The only known of the form 1{0}1 is 11 (see generalized Fermat prime), these are the primes obtained as the concatenation of a power of 10 followed by a 1. If n = 1 mod 11, then all numbers obtained as the concatenation of a power of n (>1) followed by a 1 are divisible by 11 and thus composite. Except 10, the smallest n not = 1 mod 11 such that all numbers obtained as the concatenation of a power of n (>1) followed by a 1 are composite was proven by Ɛᘔ, since all numbers obtained as the concatenation of a power of Ɛᘔ (>1) followed by a 1 are divisible by either Ɛ or 11 and thus composite. However, the smallest prime obtained as the concatenation of a power of 58 (>1) followed by a 1 is 10×58+1, it has 459655 digits.
All numbers of the form 1{5}1 are composite (proof: 1{5n}1 = (14×10−41)/Ɛ and it can be factored to (4×10−7) × ((4×10−7)/Ɛ) for odd n and divisible by 11 for even n).
The emirps below 1000 are 15, 51, 57, 5Ɛ, 75, Ɛ5, 107, 117, 11Ɛ, 12Ɛ, 13Ɛ, 145, 157, 16Ɛ, 17Ɛ, 195, 19Ɛ, 1ᘔ7, 1Ɛ5, 507, 51Ɛ, 541, 577, 587, 591, 59Ɛ, 5Ɛ1, 5ƐƐ, 701, 705, 711, 751, 76Ɛ, 775, 785, 7ᘔ1, 7ƐƐ, Ɛ11, Ɛ15, Ɛ21, Ɛ31, Ɛ61, Ɛ67, Ɛ71, Ɛ91, Ɛ95, ƐƐ5, ƐƐ7.
The non-repdigit permutable primes below 10 are 15, 57, 5Ɛ, 117, 11Ɛ, 5ƐƐƐ (the smallest representative prime of the permutation set).
The non-repdigit circular primes below 10 are 15, 57, 5Ɛ, 117, 11Ɛ, 175, 1Ɛ7, 157Ɛ, 555Ɛ, 115Ɛ77 (the smallest representative prime of the cycle).
The first few Smarandache primes are the concatenation of the first 5, 15, 4Ɛ, 151, ... positive integers.
The only known Smarandache–Wellin primes are 2 and 2357Ɛ11.
There are exactly 15 minimal primes, and they are 2, 3, 5, 7, Ɛ, 11, 61, 81, 91, 401, ᘔ41, 4441, ᘔ0ᘔ1, ᘔᘔᘔᘔ1, 44ᘔᘔᘔ1, ᘔᘔᘔ0001, ᘔᘔ000001.
The smallest weakly prime is 6Ɛ8ᘔƐ77.
The largest left-truncatable prime is 28-digit 471ᘔ34ᘔ164259Ɛᘔ16Ɛ324ᘔƐ8ᘔ32Ɛ7817, and the largest right-truncatable prime is ᘔ-digit 375ƐƐ5Ɛ515.
The only two base 10 Wieferich primes below 10 are 1685 and 5Ɛ685, note that both of the numbers end with 685, and it is conjectured that all base 10 Wieferich primes end with 685. (there is also a note for the only two known base 2 Wieferich primes (771 and 2047) minus 1 written in base 2, 8 (= 2) and 14 (= 2), 770 = 0100010001002 = 44414 is a repdigit in base 14, and 2046 = 1101101101102 = 66668 is also a repdigit in base 8, see Wieferich prime#Binary periodicity of p − 1)
There are 1, 2, 3, 5 and 6-digit (but not 4-digit) narcissistic numbers, there are totally 73 narcissistic numbers, the first few of which are 1, 2, 3, 4, 5, 6, 7, 8, 9, ᘔ, Ɛ, 25, ᘔ5, 577, 668, ᘔ83, 14765, 938ᘔ4, 369862, ᘔ2394ᘔ, ..., the largest of which is 43-digit 15079346ᘔ6Ɛ3Ɛ14ƐƐ56Ɛ395898Ɛ96629ᘔ8Ɛ01515344Ɛ4Ɛ0714Ɛ. (see OEIS: A161949)
The only two factorions are 1 and 2.
The only seven happy numbers below 1000 are 1, 10, 100, 222, 488, 848 and 884, almost all natural numbers are unhappy. All unhappy numbers get to one of these four cycles: {5, 21}, {8, 54, 35, 2ᘔ, 88, ᘔ8, 118, 56, 51, 22}, {18, 55, 42}, {68, 84}, or one of the only two fixed points other than 1: 25 and ᘔ5. (In contrast, in the decimal (base ᘔ) system there are Ɛᘔ happy numbers below 1000ᘔ (=6Ɛ4), and all unhappy number get to this cycle: {4, 16, 37, 58, 89, 145, 42, 20}, there are no fixed points other than 1)
If we use the sum of the cubes (instead of squares) of the digits, then every natural numbers get to either 1 or the cycle {8, 368, 52Ɛ, ᘔ20, 700, 247, 2ᘔ7, 947, 7ᘔ8, 10ᘔ7, 940, 561, 246, 200}. (In contrast, in the decimal (base ᘔ) system all multiple of 3 get to 153ᘔ (=109), and other numbers get to either one of these four fixed points: 1, 370, 371, 407, or one of these four cycles: {55, 250, 133}, {136, 244}, {160, 217, 352}, {919, 1459}) (for the example of the famous Hardy–Ramanujan number 1001 = 9 + ᘔ, we know that this sequence with initial term 9ᘔ is 9ᘔ, 1001, 2, 8, 368, 52Ɛ, ᘔ20, 700, 247, 2ᘔ7, 947, 7ᘔ8, 10ᘔ7, 940, 561, 246, 200, 8, 368, 52Ɛ, ᘔ20, 700, 247, 2ᘔ7, 947, 7ᘔ8, 10ᘔ7, 940, 561, 246, 200, 8, ...)
| n | fixed points and cycled for the sequence for sum of n-th powers of the digits | length of these cycles |
|---|---|---|
| 1 | {1}, {2}, {3}, {4}, {5}, {6}, {7}, {8}, {9}, {ᘔ}, {Ɛ} | 1, 1, 1, 1, 1, 1, 1, 1, 1, 1, 1 |
| 2 | {1}, {5, 21}, {8, 54, 35, 2ᘔ, 88, ᘔ8, 118, 56, 51, 22}, {18, 55, 42}, {25}, {68, 84}, {ᘔ5} | 1, 2, ᘔ, 3, 1, 2, 1 |
| 3 | {1}, {8, 368, 52Ɛ, ᘔ20, 700, 247, 2ᘔ7, 947, 7ᘔ8, 10ᘔ7, 940, 561, 246, 200}, {577}, {668}, {6Ɛ5, Ɛ74, 100ᘔ}, {ᘔ83}, {11ᘔᘔ} | 1, 12, 1, 1, 3, 1, 1 |
| 4 | {1}, {ᘔ6ᘔ, 103ᘔ8, 8256, 35ᘔ9, 9ƐᘔƐ, 22643, Ɛ69, 1102ᘔ, 596ᘔ, ᘔ842, 8394, 6442, 1080, 2455}, {206ᘔ, 6668, 4754}, {3ᘔ2Ɛ, 12396, 472Ɛ, ᘔ02ᘔ, Ɛ700, 9ᘔ42, 98ᘔ9, 13902} | 1, 12, 3, 8 |
The harshad numbers up to 200 are 1, 2, 3, 4, 5, 6, 7, 8, 9, ᘔ, Ɛ, 10, 1ᘔ, 20, 29, 30, 38, 40, 47, 50, 56, 60, 65, 70, 74, 80, 83, 90, 92, ᘔ0, ᘔ1, Ɛ0, 100, 10ᘔ, 110, 115, 119, 120, 122, 128, 130, 134, 137, 146, 150, 153, 155, 164, 172, 173, 182, 191, 1ᘔ0, 1Ɛ0, 1Ɛᘔ, 200, although the sequence of factorials begins with harshad numbers, not all factorials are harshad numbers, after 7! (=2Ɛ00, with digit sum 11 but 11 does not divide 7!), 8ᘔ4! is the next that is not (8ᘔ4! has digit sum 8275 = Ɛ×8Ɛ7, thus not divide 8ᘔ4!). There are no 21 consecutive integers that are all harshad numbers, but there are infinitely many 20-tuples of consecutive integers that are all harshad numbers.
The Kaprekar numbers up to 10000 are 1, Ɛ, 56, 66, ƐƐ, 444, 778, ƐƐƐ, 12ᘔᘔ, 1640, 2046, 2929, 3333, 4973, 5Ɛ60, 6060, 7249, 8889, 9293, 9Ɛ76, ᘔ580, ᘔ912, ƐƐƐƐ.
The Kaprekar's routine of any four-digit number which is not repdigit converges to either the cycle {3ƐƐ8, 8284, 6376} or the cycle {4198, 8374, 5287, 6196, 7ƐƐ4, 7375}, and the Kaprekar map of any three-digit number which is not repdigit converges to the fixed point 5Ɛ6, and the Kaprekar map of any two-digit number which is not repdigit converges to the cycle {0Ɛ, ᘔ1, 83, 47, 29, 65}.
| n | cycles for Kaprekar's routine for n-digit numbers | length of these cycles |
|---|---|---|
| 1 | {0} | 1 |
| 2 | {00}, {0Ɛ, ᘔ1, 83, 47, 29, 65} | 1, 6 |
| 3 | {000}, {5Ɛ6} | 1, 1 |
| 4 | {0000}, {3ƐƐ8, 8284, 6376}, {4198, 8374, 5287, 6196, 7ƐƐ4, 7375} | 1, 3, 6 |
| 5 | {00000}, {64Ɛ66, 6ƐƐƐ5}, {83Ɛ74} | 1, 2, 1 |
| 6 | {000000}, {420ᘔ98, ᘔ73742, 842874, 642876, 62ƐƐ86, 951963, 860ᘔ54, ᘔ40ᘔ72, ᘔ82832, 864654}, {65ƐƐ56} | 1, ᘔ, 1 |
The self numbers up to 600 are 1, 3, 5, 7, 9, Ɛ, 20, 31, 42, 53, 64, 75, 86, 97, ᘔ8, Ɛ9, 10ᘔ, 110, 121, 132, 143, 154, 165, 176, 187, 198, 1ᘔ9, 1Ɛᘔ, 20Ɛ, 211, 222, 233, 244, 255, 266, 277, 288, 299, 2ᘔᘔ, 2ƐƐ, 310, 312, 323, 334, 345, 356, 367, 378, 389, 39ᘔ, 3ᘔƐ, 400, 411, 413, 424, 435, 446, 457, 468, 479, 48ᘔ, 49Ɛ, 4Ɛ0, 501, 512, 514, 525, 536, 547, 558, 569, 57ᘔ, 58Ɛ, 5ᘔ0, 5Ɛ1.
The Friedman numbers up to 1000 are 121=11, 127=7×21, 135=5×31, 144=4×41, 163=3×61, 368=8, 376=6×73, 441=(4+1), 445=5+4.
The Keith numbers up to 1000 are 11, 15, 1Ɛ, 22, 2ᘔ, 31, 33, 44, 49, 55, 62, 66, 77, 88, 93, 99, ᘔᘔ, ƐƐ, 125, 215, 24ᘔ, 405, 42ᘔ, 654, 80ᘔ, 8ᘔ3, ᘔ59.
There are totally 71822 polydivisible numbers, the largest of which is 24-digit 606890346850Ɛᘔ6800Ɛ036206464. However, there are no Ɛ-digit polydivisible numbers contain the digits 1 to Ɛ exactly once each.
The candidate Lychrel numbers up to 1000 are 179, 1Ɛ9, 278, 2Ɛ8, 377, 3Ɛ7, 476, 4Ɛ6, 575, 5Ɛ5, 674, 6Ɛ4, 773, 7Ɛ3, 872, 8Ɛ2, 971, 9Ɛ1, ᘔ2Ɛ, ᘔ3Ɛ, ᘔ5Ɛ, ᘔ70, ᘔᘔƐ, ᘔƐ0, Ɛ2ᘔ, Ɛ3ᘔ, Ɛ5ᘔ, Ɛᘔᘔ. The only suspected Lychrel seed numbers up to 1000 are 179, 1Ɛ9, ᘔ3Ɛ and ᘔ5Ɛ. However, it is unknown whether any Lychrel number exists. (Lychrel numbers only known to exist in these bases: Ɛ, 15, 18, 22 and all powers of 2)
Most numbers that end with 2 are nontotient (in fact, all nontotients < 58 except 2ᘔ end with 2), except 2 itself, the first counterexample is 92, which equals φ(ᘔ1) = φ(Ɛ) and φ(182) = φ(2×Ɛ), next counterexample is 362, which equals φ(381) = φ(1Ɛ) and φ(742) = φ(2×1Ɛ), there are only 9 such numbers ≤ 10000 (the number 2 itself is not counted), all such numbers (except the number 2 itself) are of the form φ(p) = p(p−1), where p is a prime ends with Ɛ.
If we let the musical notes in an octave be numbers in the cyclic group Z10: C=0, C#=1, D=2, Eb=3, E=4, F=5, F#=6, G=7, Ab=8, A=9, Bb=ᘔ, B=Ɛ (see pitch class and music scale) (thus, if we let the middle C be 0, then the notes in a piano are -33 to 40), then x and x+3 are small 3-degree, x and x+4 are big 3-degree, x and x+7 are perfect 5 degree (thus, we can use 7x for x = 0 to Ɛ to get the five degree cycle), etc. (since an octave is 10 semitones, a small 3-degree is 3 semitones, a big 3-degree is 4 semitones, and a perfect 5 degree is 7 semitones, etc.) (if we let an octave be 1, then a semitone will be 0.1, and we can write all 10 notes on a cycle, the difference of two connected notes is 26 degrees or radians) Besides, the x major chord (x) is {x, x+4, x+7} in Z10, and the x minor chord (xm) is {x, x+3, x+7} in Z10, and the x major 7th chord (x) is {x, x+4, x+7, x+Ɛ}, and the x minor 7th chord (x) is {x, x+3, x+7, x+ᘔ}, and the x dominant 7th chord (x) is {x, x+4, x+7, x+ᘔ}, and the x diminished 7th triad (x) is {x, x+3, x+6, x+9}, since the frequency of x and x+6 is not simple integer fraction, they are not harmonic, and this diminished 7th triad is corresponding the beast number 666 (three 6's). Besides, x major scale uses the notes {x, x+2, x+4, x+5, x+7, x+9, x+Ɛ}, and x minor scale uses the notes {x, x+2, x+3, x+5, x+7, x+8, x+ᘔ}. Besides, the frequency of x+10 is twice as that of x, the frequency of x+7 is 1.6 (=3/2) times as that of x, and the frequency of x+5 is 1.4 (=4/3) times as that of x, they are all simple integer fractions (ratios of small integers), and they all have at most one digit after the duodecimal point, and we can found that 1.6 = ᘔ9.8Ɛ5809 is very close to 2 = ᘔ8, since 2 = 2134ᘔ8 is very close to 3 = 217669, the simple frequency fractions found for the scales are only 0.6, 0.8, 0.9, 1.4, 1.6 and 2, however, since the frequency of x+10 is twice as that of x, thus the frequency of x+1 (i.e. a semitone higher than x) is (=2) times as that of x. Let f(x) be the frequency of x, then we have f(2)/f(0) = 9/8 (=1.16), f(4)/f(2) = ᘔ/9 (=1.14), and f(5)/f(4) = 14/13 (this number is very close to ), and thus we have that f(5)/f(0) = (9/8) × (ᘔ/9) × (14/13) = 4/3. Also, we can found that 2 is very close to 1.4, and 2 is very close to 1.6.
All orders of non-cyclic simple group end with 0 (thus, all orders of unsolvable group end with 0), however, we can prove that no groups with order 10, 20, 30 or 40 are simple, thus 50 is the smallest order of non-cyclic simple group (thus, all groups with order < 50 are solvable), (50 is the order of the alternating group A5, which is a non-cyclic simple group, and thus an unsolvable group) next three orders of non-cyclic simple group are 120, 260 and 360. (Edit: I found that this is not completely true (although this is true for all orders ≤ 14000), the smallest counterexample is 14ᘔ28, however, all such orders are divisible by 4 and either 3 or 5 (i.e. divisible by either 10 or 18), and all such orders have at least 3 distinct prime factors, by these conditions, the smallest possible such order is indeed 50 = 2 × 3 × 5, next possible such order is 70 = 2 × 3 × 7, however, by Sylow theorems, the number of Sylow 7-subgroups of all groups with order 70 (i.e. the number of subgroups with order 7 of all groups with order 70) is congruent to 1 mod 7 and divides 70, hence must be 1, thus the subgroup with order 7 is a normal subgroup of the group with order 70, thus all groups with order 70 have a nontrivial normal subgroup and cannot be simple groups)
Some notable numbers
(In this section, all numbers are written with duodecimal, using X for ten and E for eleven)
In duodecimal, as in decimal, there are special numbers that exhibit digit patterns when, for example, multiplied by small integers:
2497 * 1 = 2497 2497 * 2 = 4972 2497 * 3 = 7249 2497 * 4 = 9724 2497 * 5 = EEEE
186X35 * 1 = 186X35 186X35 * 2 = 35186X 186X35 * 3 = 5186X3 186X35 * 4 = 6X3518 186X35 * 5 = 86X351 186X35 * 6 = X35186 186X35 * 7 = EEEEEE
8579214E36429X7 * 1 = 8579214E36429X7 8579214E36429X7 * 2 = 14E36429X7085792 8579214E36429X7 * 3 = 214E36429X708579 8579214E36429X7 * 4 = 29X708579214E364 8579214E36429X7 * 5 = 36429X708579214E 8579214E36429X7 * 6 = 429X708579214E36 8579214E36429X7 * 7 = 4E36429X70857921 8579214E36429X7 * 8 = 579214E36429X708 8579214E36429X7 * 9 = 6429X708579214E3 8579214E36429X7 * X = 708579214E36429X 8579214E36429X7 * E = 79214E36429X7085 8579214E36429X7 * 10 = 8579214E36429X70 8579214E36429X7 * 11 = 9214E36429X70857 8579214E36429X7 * 12 = 9X708579214E3642 8579214E36429X7 * 13 = X708579214E36429 8579214E36429X7 * 14 = E36429X708579214 8579214E36429X7 * 15 = EEEEEEEEEEEEEEEE
123456789XE * 1 = 123456789XE 123456789XE * 2 = 2468E13579X 123456789XE * 3 = 36X147E2589 123456789XE * 4 = 4915X26E378 123456789XE * 5 = 5E4X3928167 123456789XE * 6 = 718293X4E56 123456789XE * 7 = 83E72X61945 123456789XE * 8 = 962E851X734 123456789XE * 9 = X8641E97523 123456789XE * X = EX987654312 123456789XE * E = 111111111101
123456789E * 1 = 123456789E (miss digit X) 123456789E * 2 = 2468E1357X (miss digit 9) 123456789E * 3 = 36X147E259 (miss digit 8) 123456789E * 4 = 4915X26E38 (miss digit 7) 123456789E * 5 = 5E4X392817 (miss digit 6) 123456789E * 6 = 718293X4E6 (miss digit 5) 123456789E * 7 = 83E72X6195 (miss digit 4) 123456789E * 8 = 962E851X74 (miss digit 3) 123456789E * 9 = X8641E9753 (miss digit 2) 123456789E * X = EX98765432 (miss digit 1) 123456789E * E = 11111111111
275 * 5 = 1111 275 * X = 2222 275 * 13 = 3333 275 * 18 = 4444 275 * 21 = 5555 275 * 26 = 6666 275 * 2E = 7777 275 * 34 = 8888 275 * 39 = 9999 275 * 42 = XXXX 275 * 47 = EEEE
1X537 * 7 = 111111 1X537 * 12 = 222222 1X537 * 19 = 333333 1X537 * 24 = 444444 1X537 * 2E = 555555 1X537 * 36 = 666666 1X537 * 41 = 777777 1X537 * 48 = 888888 1X537 * 53 = 999999 1X537 * 5X = XXXXXX 1X537 * 65 = EEEEEE
123456789E * E = 11111111111 123456789E * 1X = 22222222222 123456789E * 29 = 33333333333 123456789E * 38 = 44444444444 123456789E * 47 = 55555555555 123456789E * 56 = 66666666666 123456789E * 65 = 77777777777 123456789E * 74 = 88888888888 123456789E * 83 = 99999999999 123456789E * 92 = XXXXXXXXXXX 123456789E * X1 = EEEEEEEEEEE
92X79E43715865 * 15 = 1111111111111111 92X79E43715865 * 2X = 2222222222222222 92X79E43715865 * 43 = 3333333333333333 92X79E43715865 * 58 = 4444444444444444 92X79E43715865 * 71 = 5555555555555555 92X79E43715865 * 86 = 6666666666666666 92X79E43715865 * 9E = 7777777777777777 92X79E43715865 * E4 = 8888888888888888 92X79E43715865 * 109 = 9999999999999999 92X79E43715865 * 122 = XXXXXXXXXXXXXXXX 92X79E43715865 * 137 = EEEEEEEEEEEEEEEE
8327 * 17 = 111111 8327 * 32 = 222222 8327 * 49 = 333333 8327 * 64 = 444444 8327 * 7E = 555555 8327 * 96 = 666666 8327 * E1 = 777777 8327 * 108 = 888888 8327 * 123 = 999999 8327 * 13X = XXXXXX 8327 * 155 = EEEEEE
55 * 25 = 1111 55 * 4X = 2222 55 * 73 = 3333 55 * 98 = 4444 55 * 101 = 5555 55 * 126 = 6666 55 * 14E = 7777 55 * 174 = 8888 55 * 199 = 9999 55 * 202 = XXXX 55 * 227 = EEEE
1221 * E1 = 111111 1221 * 1X2 = 222222 1221 * 293 = 333333 1221 * 384 = 444444 1221 * 475 = 555555 1221 * 566 = 666666 1221 * 657 = 777777 1221 * 748 = 888888 1221 * 839 = 999999 1221 * 92X = XXXXXX 1221 * X1E = EEEEEE
112233445566778899XE * EE = 1111111111111111111111 112233445566778899XE * 1EX = 2222222222222222222222 112233445566778899XE * 2E9 = 3333333333333333333333 112233445566778899XE * 3E8 = 4444444444444444444444 112233445566778899XE * 4E7 = 5555555555555555555555 112233445566778899XE * 5E6 = 6666666666666666666666 112233445566778899XE * 6E5 = 7777777777777777777777 112233445566778899XE * 7E4 = 8888888888888888888888 112233445566778899XE * 8E3 = 9999999999999999999999 112233445566778899XE * 9E2 = XXXXXXXXXXXXXXXXXXXXXX 112233445566778899XE * XE1 = EEEEEEEEEEEEEEEEEEEEEE
E1X2938475665748392X1E1 * 121 = 11111111111111111111111111 E1X2938475665748392X1E1 * 242 = 22222222222222222222222222 E1X2938475665748392X1E1 * 363 = 33333333333333333333333333 E1X2938475665748392X1E1 * 484 = 44444444444444444444444444 E1X2938475665748392X1E1 * 5X5 = 55555555555555555555555555 E1X2938475665748392X1E1 * 706 = 66666666666666666666666666 E1X2938475665748392X1E1 * 827 = 77777777777777777777777777 E1X2938475665748392X1E1 * 948 = 88888888888888888888888888 E1X2938475665748392X1E1 * X69 = 99999999999999999999999999 E1X2938475665748392X1E1 * E8X = XXXXXXXXXXXXXXXXXXXXXXXXXX E1X2938475665748392X1E1 * 10XE = EEEEEEEEEEEEEEEEEEEEEEEEEE
1^2 = 01, 0 + 1 = 1 E^2 = X1, X + 1 = E 56^2 = 2630, 26 + 30 = 56 66^2 = 3630, 36 + 30 = 66 EE^2 = EX01, EX + 01 = EE 444^2 = 170294, 170 + 294 = 444 778^2 = 4X4294, 4X4 + 294 = 778 EEE^2 = EEX001, EEX + 001 = EEE 12XX^2 = 1661144, 166 + 1144 = 12XX 1640^2 = 2401400, 240 + 1400 = 1640 2046^2 = 4161830, 416 + 1830 = 2046 2929^2 = 7802169, 780 + 2169 = 2929 3333^2 = X862469, X86 + 2469 = 3333 4973^2 = 1E062X69, 1E06 + 2X69 = 4973 5E60^2 = 2E603000, 2E60 + 3000 = 5E60
2^2 + 5^2 = 25 X^2 + 5^2 = X5 5^3 + 7^3 + 7^3 = 577 6^3 + 6^3 + 8^3 = 668 X^3 + 8^3 + 3^3 = X83 1^5 + 4^5 + 7^5 + 6^5 + 5^5 = 14765 9^5 + 3^5 + 8^5 + X^5 + 4^5 = 938X4 3^6 + 6^6 + 9^6 + 8^6 + 6^6 + 2^6 = 369862 X^6 + 2^6 + 3^6 + 9^6 + 4^6 + X^6 = X2394X
55 * 99 = 4499 77 * 88 = 5588 XX * XX = 9944 66 * EE = 6556
14 = (1 * 4)^2 20 = (2^2 + 0^2)! 21 = (2^2 + 1^2)^2 24 = 2^2 + 4! 30 = ((3 + 0)!)^2 43 = 4! + 3^3 48 = 4^3 - 8 84 = (8 + sqrt(4))^2 X1 = (X + 1)^2 X5 = (X - 5)^3 X8 = (X - 8)^7 XE = X + E^2 121 = (12 - 1)^2 160 = (1 * 6 + 0)^3 169 = (1 * (6 + 9))^2 183 = ((1 + 8) / 3)^5 194 = ((1 - 9) / 4)^8 230 = (30 / 2)^2 243 = - 2^2 + (4 + 3)^3 247 = (2 - sqrt(4) + 7)^3 294 = (2 * 9 + sqrt(4))^2 344 = (3! + 4 * 4)^2 381 = (3 * 8 - 1)^2
1 * X + 1 = E
12 * X + 2 = EX
123 * X + 3 = EX9
1234 * X + 4 = EX98
12345 * X + 5 = EX987
123456 * X + 6 = EX9876
1234567 * X + 7 = EX98765
12345678 * X + 8 = EX987654
123456789 * X + 9 = EX9876543
123456789X * X + X = EX98765432
123456789XE * X + E = EX987654321
0 * E + 1 = 1
1 * E + 2 = 11
12 * E + 3 = 111
123 * E + 4 = 1111
1234 * E + 5 = 11111
12345 * E + 6 = 111111
123456 * E + 7 = 1111111
1234567 * E + 8 = 11111111
12345678 * E + 9 = 111111111
123456789 * E + X = 1111111111
123456789X * E + E = 11111111111
123456789XE * E + 10 = 111111111111
1^2 = 1
11^2 = 121
111^2 = 12321
1111^2 = 1234321
11111^2 = 123454321
111111^2 = 12345654321
1111111^2 = 1234567654321
11111111^2 = 123456787654321
111111111^2 = 12345678987654321
1111111111^2 = 123456789X987654321
11111111111^2 = 123456789XEX987654321
111111111111^2 = 123456789E00EX987654321 (note: Rn^2 is not palindromic number if n >= 10)
E^2 = X1
EE^2 = EX01
EEE^2 = EEX001
EEEE^2 = EEEX0001
EEEEE^2 = EEEEX00001
EEEEEE^2 = EEEEEX000001
EEEEEEE^2 = EEEEEEX0000001
EEEEEEEE^2 = EEEEEEEX00000001
EEEEEEEEE^2 = EEEEEEEEX000000001
EEEEEEEEEE^2 = EEEEEEEEEX0000000001
EEEEEEEEEEE^2 = EEEEEEEEEEX00000000001
EEEEEEEEEEEE^2 = EEEEEEEEEEEX000000000001
3 * 4 = 10
33 * 44 = 1210
333 * 444 = 123210
3333 * 4444 = 12343210
33333 * 44444 = 1234543210
333333 * 444444 = 123456543210
3333333 * 4444444 = 12345676543210
33333333 * 44444444 = 1234567876543210
333333333 * 444444444 = 123456789876543210
3333333333 * 4444444444 = 123456789X9876543210
33333333333 * 44444444444 = 123456789XEX9876543210
333333333333 * 444444444444 = 123456789E00EX9876543210
6 * E = 56
66 * EE = 6556
666 * EEE = 665556
6666 * EEEE = 66655556
66666 * EEEEE = 6666555556
666666 * EEEEEE = 666665555556
6666666 * EEEEEEE = 66666655555556
66666666 * EEEEEEEE = 6666666555555556
666666666 * EEEEEEEEE = 666666665555555556
6666666666 * EEEEEEEEEE = 66666666655555555556
66666666666 * EEEEEEEEEEE = 6666666666555555555556
666666666666 * EEEEEEEEEEEE = 666666666665555555555556
10XE * 1 = 10XE 10XE * 2 = 219X 10XE * 3 = 3289 10XE * 4 = 4378 10XE * 5 = 5467 10XE * 6 = 6556 10XE * 7 = 7645 10XE * 8 = 8734 10XE * 9 = 9823 10XE * X = X912 10XE * E = EX01
10EXE * 1 = 10EXE 10EXE * 2 = 21E9X 10EXE * 3 = 32E89 10EXE * 4 = 43E78 10EXE * 5 = 54E67 10EXE * 6 = 65E56 10EXE * 7 = 76E45 10EXE * 8 = 87E34 10EXE * 9 = 98E23 10EXE * X = X9E12 10EXE * E = EXE01
1 / E = 0.111111111111 ... 1 / 11 = 0.0E 0E 0E 0E 0E 0E ... 1 / EE = 0.01 01 01 01 01 01 ... 1 / 101 = 0.00EE 00EE 00EE ... 1 / E1 = 0.010EXE 010EXE ... 1 / 111 = 0.00E 00E 00E 00E ... 1 / X1 = 0.0123456789E 0123456789E ... 1 / 121 = 0.00X28466482X0 EE1937557391E ...
1 / XE1 = 0.00112233445566778899XE 00112233445566778899XE ... 1 / XEE1 = 0.000111222333444555666777888999XXE 000111222333444555666777888999XXE ... 1 / XEEE1 = 0.0000111122223333444455556666777788889999XXXE 0000111122223333444455556666777788889999XXXE ...
1 / EX01 = 0.00 01 02 03 04 05 06 07 08 09 0X 0E 10 11 12 13 14 15 16 17 18 19 1X 1E 20 21 22 23 24 25 26 27 28 29 2X 2E 30 31 32 33 34 35 36 37 38 39 3X 3E 40 41 42 43 44 45 46 47 48 49 4X 4E 50 51 52 53 54 55 56 57 58 59 5X 5E 60 61 62 63 64 65 66 67 68 69 6X 6E 70 71 72 73 74 75 76 77 78 79 7X 7E 80 81 82 83 84 85 86 87 88 89 8X 8E 90 91 92 93 94 95 96 97 98 99 9X 9E X0 X1 X2 X3 X4 X5 X6 X7 X8 X9 XX XE E0 E1 E2 E3 E4 E5 E6 E7 E8 E9 EE 00 01 02 03 04 05 06 07 08 09 0X 0E 10 11 12 13 14 15 16 17 18 19 1X 1E 20 21 22 23 24 25 26 27 28 29 2X 2E 30 31 32 33 34 35 36 37 38 39 3X 3E 40 41 42 43 44 45 46 47 48 49 4X 4E 50 51 52 53 54 55 56 57 58 59 5X 5E 60 61 62 63 64 65 66 67 68 69 6X 6E 70 71 72 73 74 75 76 77 78 79 7X 7E 80 81 82 83 84 85 86 87 88 89 8X 8E 90 91 92 93 94 95 96 97 98 99 9X 9E X0 X1 X2 X3 X4 X5 X6 X7 X8 X9 XX XE E0 E1 E2 E3 E4 E5 E6 E7 E8 E9 EE ...
1 / EEX001 = 0.000 001 002 003 004 005 006 007 008 009 00X 00E 010 011 012 013 014 ... 1 / EEEX0001 = 0.0000 0001 0002 0003 0004 0005 0006 0007 0008 0009 000X 000E 0010 0011 0012 0013 0014 ...
1 / EXE01 = 0.0000101111212222323333434444545555656666767777878888989999X9XXXXEE 0000101111212222323333434444545555656666767777878888989999X9XXXXEE ...
1 / EXEE = 0.00 01 01 02 03 05 08 11 19 2X 47 ... 1 / EEXEEE = 0.000 001 001 002 003 005 008 011 019 02X 047 075 100 175 275 42X 6X3 ... 1 / EEEXEEEE = 0.0000 0001 0001 0002 0003 0005 0008 0011 0019 002X 0047 0075 0100 0175 0275 042X 06X3 0E11 15E4 ...
1 / EX = 0.01 02 04 08 14 28 54 X8
194
368
714
1228
2454 ... (powers of 2)
1 / E9 = 0.01 03 09 23 69
183
509
1323
3969 ... (powers of 3)
1 / E8 = 0.01 04 14 54
194
714
2454 ... (powers of 4)
1 / XE = 0.0112358
11
19
2X
47
75
100
175
275
42X
6X3
E11
15E4 ... (Fibonacci numbers)
1 / 9E = 0.0125
10
25
5X
121
2X0
6X1
1462 ... (Pell numbers)
1 / 5E = 0.02 04 08 14 28 54 X8
194
368
714
1228
2454 ... (powers of 2)
1 / 3E = 0.03 09 23 69
183
509
1323
3969 ... (powers of 3)
1 / 2E = 0.04 14 54
194
714
2454 ... (powers of 4)
148421
28
54
X8
194
368
714
1228
1 / 1E = (powers of 2) ... 2454
23931
69
183
509
1323
1 / 2E = (power of 3) ... 3969
1441
54
194
714
1 / 3E = (power of 4) ... 2454
11853211
19
2X
47
75
100
175
275
42X
6X3
E11
1 / 10E = (Fibonacci numbers) ... 15E4
10521
25
5X
121
2X0
6X1
1 / 11E = (Pell numbers) ... 1462
ab * 5 * 25 = abab ab * 7 * 17 * 111 = ababab ab * 5 * 25 * 75 * 175 = abababab ab * E0E1 * 11111 = ababababab ab * 5 * 7 * 17 * 25 * 111 * EE01 = abababababab abc * 7 * 11 * 17 = abcabc abc * 31 * 3X891 = abcabcabc abc * 5 * 7 * 11 * 17 * 25 * EE01 = abcabcabcabc abcd * 75 * 175 = abcdabcd abcd * 7 * 17 * 111 * EE01 = abcdabcdabcd abcde * 11 * E0E1 = abcdeabcde abcdef * 5 * 25 * EE01 = abcdefabcdef (where a, b, c, d, e, f are any digits)
Prime numbers
(In this section, all numbers are written with duodecimal)
A natural number (i.e. 1, 2, 3, 4, 5, 6, etc.) is called a prime number (or a prime) if it has exactly two positive divisors, 1 and the number itself. Natural numbers greater than 1 that are not prime are called composite.
The first 1ᘔ5 prime numbers (all the prime numbers less than 1000) are:
- 2, 3, 5, 7, Ɛ, 11, 15, 17, 1Ɛ, 25, 27, 31, 35, 37, 3Ɛ, 45, 4Ɛ, 51, 57, 5Ɛ, 61, 67, 6Ɛ, 75, 81, 85, 87, 8Ɛ, 91, 95, ᘔ7, ᘔƐ, Ɛ5, Ɛ7, 105, 107, 111, 117, 11Ɛ, 125, 12Ɛ, 131, 13Ɛ, 141, 145, 147, 157, 167, 16Ɛ, 171, 175, 17Ɛ, 181, 18Ɛ, 195, 19Ɛ, 1ᘔ5, 1ᘔ7, 1Ɛ1, 1Ɛ5, 1Ɛ7, 205, 217, 21Ɛ, 221, 225, 237, 241, 24Ɛ, 251, 255, 25Ɛ, 267, 271, 277, 27Ɛ, 285, 291, 295, 2ᘔ1, 2ᘔƐ, 2Ɛ1, 2ƐƐ, 301, 307, 30Ɛ, 315, 321, 325, 327, 32Ɛ, 33Ɛ, 347, 34Ɛ, 357, 35Ɛ, 365, 375, 377, 391, 397, 3ᘔ5, 3ᘔƐ, 3Ɛ5, 3Ɛ7, 401, 40Ɛ, 415, 41Ɛ, 421, 427, 431, 435, 437, 447, 455, 457, 45Ɛ, 465, 46Ɛ, 471, 481, 485, 48Ɛ, 497, 4ᘔ5, 4Ɛ1, 4ƐƐ, 507, 511, 517, 51Ɛ, 527, 531, 535, 541, 545, 557, 565, 575, 577, 585, 587, 58Ɛ, 591, 59Ɛ, 5Ɛ1, 5Ɛ5, 5Ɛ7, 5ƐƐ, 611, 615, 617, 61Ɛ, 637, 63Ɛ, 647, 655, 661, 665, 66Ɛ, 675, 687, 68Ɛ, 695, 69Ɛ, 6ᘔ7, 6Ɛ1, 701, 705, 70Ɛ, 711, 71Ɛ, 721, 727, 735, 737, 745, 747, 751, 767, 76Ɛ, 771, 775, 77Ɛ, 785, 791, 797, 7ᘔ1, 7ƐƐ, 801, 80Ɛ, 817, 825, 82Ɛ, 835, 841, 851, 855, 85Ɛ, 865, 867, 871, 881, 88Ɛ, 8ᘔ5, 8ᘔ7, 8ᘔƐ, 8Ɛ5, 8Ɛ7, 901, 905, 907, 90Ɛ, 91Ɛ, 921, 927, 955, 95Ɛ, 965, 971, 987, 995, 9ᘔ7, 9ᘔƐ, 9Ɛ1, 9Ɛ5, 9ƐƐ, ᘔ07, ᘔ0Ɛ, ᘔ11, ᘔ17, ᘔ27, ᘔ35, ᘔ37, ᘔ3Ɛ, ᘔ41, ᘔ45, ᘔ4Ɛ, ᘔ5Ɛ, ᘔ6Ɛ, ᘔ77, ᘔ87, ᘔ91, ᘔ95, ᘔ9Ɛ, ᘔᘔ7, ᘔᘔƐ, ᘔƐ7, ᘔƐƐ, Ɛ11, Ɛ15, Ɛ1Ɛ, Ɛ21, Ɛ25, Ɛ2Ɛ, Ɛ31, Ɛ37, Ɛ45, Ɛ61, Ɛ67, Ɛ6Ɛ, Ɛ71, Ɛ91, Ɛ95, Ɛ97, Ɛᘔ5, ƐƐ5, ƐƐ7
Except 2 and 3, all primes end with 1, 5, 7 or Ɛ. The first k such that all of 10k, 10k + 1, 10k + 2, ..., 10k + Ɛ are all composite is 38, i.e. all of 380, 381, 382, ..., 38Ɛ are composite.
The density of primes end with 1 is a relatively low (< 1/4), but the density of primes end with 5, 7 and Ɛ are nearly equal (all are a little more than 1/4). (i.e. for a given natural number N, the number of primes end with 1 less than N is usually smaller than the number of primes end with 5 (or 7, or Ɛ) less than N) e.g. For all 1426 primes < 10000, there are 3Ɛ8 primes (2Ɛ.3%) end with 1, 410 primes (30.3%) end with 5, 412 primes (30.5%) end with 7, 406 primes (2Ɛ.Ɛ%) end with Ɛ. It is conjectured that for every natural number N ≥ 10, the number of primes end with 1 less than N is smaller than the number of primes end with 5 (or 7, or Ɛ) less than N. (Note: the percentage in this sequence are also in duodecimal, i.e. 20% means 0.2 or 20/100 = 1/6, 36% means 0.36 or 36/100 = 7/20, 58.7% means 0.587 or 587/1000)
13665 is the smallest prime p such that the number of primes end with 1 or 5 ≤ p is more than the number of primes end with 3, 7 or Ɛ ≤ p (see OEIS: A007350, of course, 3 is the only prime ends with 3). Besides, 9ᘔ03693ᘔ831 is the smallest prime p such that the number of primes end with 1 or 7 ≤ p is more than the number of primes end with 2, 5 or Ɛ ≤ p (see OEIS: A007352, of course, 2 is the only prime ends with 2). Question: What is the smallest prime p such that the number of primes end with 1 ≤ p is more than the number of primes end with d ≤ p for at least one of d = 5, 7 or Ɛ?
All squares of primes (except 2 and 3) end with 1.
There are 2ᘔ primes between 1 and 100, 23 primes between 101 and 200, 1ᘔ primes between 201 and 300, 1ᘔ primes between 301 and 400, 1Ɛ primes between 401 and 500, 1ᘔ primes between 501 and 600, 16 primes between 601 and 700, 1ᘔ primes between 701 and 800, 18 primes between 801 and 900, 16 primes between 901 and ᘔ00, 1ᘔ primes between ᘔ01 and Ɛ00, 17 primes between Ɛ01 and 1000.
There are about N/ln(N) primes less than N, where ln is the natural logarithm, i.e. the logarithm with base e = 2.875236069821... (see prime number theorem), thus there are about primes less than 10 (i.e. with at most n digits), and ln(10) = 2.599Ɛ035Ɛ8169...
| N | total numbers of primes ≤ N | numbers of primes end with 1 ≤ N | numbers of primes end with 5 ≤ N | numbers of primes end with 7 ≤ N | numbers of primes end with Ɛ ≤ N |
|---|---|---|---|---|---|
| 10 | 5 | 0 | 1 | 1 | 1 |
| 40 | 13 | 2 | 4 | 4 | 3 |
| 100 | 2ᘔ | 6 | 9 | 9 | 8 |
| 400 | 89 | 1ᘔ | 23 | 23 | 23 |
| 1000 | 1ᘔ5 | 51 | 59 | 59 | 58 |
| 4000 | 621 | 157 | 16ᘔ | 170 | 166 |
| 10000 | 1426 | 3Ɛ8 | 410 | 412 | 406 |
| 40000 | 4833 | 11ᘔ4 | 121Ɛ | 1219 | 1211 |
| 100000 | 10852 | 31ᘔ4 | 3225 | 3225 | 321ᘔ |
| 400000 | 3928Ɛ | Ɛ333 | Ɛ377 | Ɛ3Ɛ9 | Ɛ3ᘔ2 |
| 1000000 | ᘔ4Ɛ20 | 27204 | 27295 | 2730ᘔ | 27333 |
In the following table, numbers shaded in cyan are primes.
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ᘔ | Ɛ | 10 |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 1ᘔ | 1Ɛ | 20 |
| 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 2ᘔ | 2Ɛ | 30 |
| 31 | 32 | 33 | 34 | 35 | 36 | 37 | 38 | 39 | 3ᘔ | 3Ɛ | 40 |
| 41 | 42 | 43 | 44 | 45 | 46 | 47 | 48 | 49 | 4ᘔ | 4Ɛ | 50 |
| 51 | 52 | 53 | 54 | 55 | 56 | 57 | 58 | 59 | 5ᘔ | 5Ɛ | 60 |
| 61 | 62 | 63 | 64 | 65 | 66 | 67 | 68 | 69 | 6ᘔ | 6Ɛ | 70 |
| 71 | 72 | 73 | 74 | 75 | 76 | 77 | 78 | 79 | 7ᘔ | 7Ɛ | 80 |
| 81 | 82 | 83 | 84 | 85 | 86 | 87 | 88 | 89 | 8ᘔ | 8Ɛ | 90 |
| 91 | 92 | 93 | 94 | 95 | 96 | 97 | 98 | 99 | 9ᘔ | 9Ɛ | ᘔ0 |
| ᘔ1 | ᘔ2 | ᘔ3 | ᘔ4 | ᘔ5 | ᘔ6 | ᘔ7 | ᘔ8 | ᘔ9 | ᘔᘔ | ᘔƐ | Ɛ0 |
| Ɛ1 | Ɛ2 | Ɛ3 | Ɛ4 | Ɛ5 | Ɛ6 | Ɛ7 | Ɛ8 | Ɛ9 | Ɛᘔ | ƐƐ | 100 |
Divisibility rules
(In this section, all numbers are written with duodecimal)
This section is about the divisibility rules in duodecimal.
- 1
Any integer is divisible by 1.
- 2
If a number is divisible by 2 then the unit digit of that number will be 0, 2, 4, 6, 8 or ᘔ.
- 3
If a number is divisible by 3 then the unit digit of that number will be 0, 3, 6 or 9.
- 4
If a number is divisible by 4 then the unit digit of that number will be 0, 4 or 8.
- 5
To test for divisibility by 5, double the units digit and subtract the result from the number formed by the rest of the digits. If the result is divisible by 5 then the given number is divisible by 5.
This rule comes from 21(5*5)
Examples:
13 rule => |1-2*3| = 5 which is divisible by 5.
2Ɛᘔ5 rule => |2Ɛᘔ-2*5| = 2Ɛ0(5*70) which is divisible by 5(or apply the rule on 2Ɛ0).
OR
To test for divisibility by 5, subtract the units digit and triple of the result to the number formed by the rest of the digits. If the result is divisible by 5 then the given number is divisible by 5.
This rule comes from 13(5*3)
Examples:
13 rule => |3-3*1| = 0 which is divisible by 5.
2Ɛᘔ5 rule => |5-3*2Ɛᘔ| = 8Ɛ1(5*195) which is divisible by 5(or apply the rule on 8Ɛ1).
OR
Form the alternating sum of blocks of two from right to left. If the result is divisible by 5 then the given number is divisible by 5.
This rule comes from 101, since 101 = 5*25, thus this rule can be also tested for the divisibility by 25.
Example:
97,374,627 => 27-46+37-97 = -7Ɛ which is divisible by 5.
- 6
If a number is divisible by 6 then the unit digit of that number will be 0 or 6.
- 7
To test for divisibility by 7, triple the units digit and add the result to the number formed by the rest of the digits. If the result is divisible by 7 then the given number is divisible by 7.
This rule comes from 2Ɛ(7*5)
Examples:
12 rule => |3*2+1| = 7 which is divisible by 7.
271Ɛ rule => |3*Ɛ+271| = 29ᘔ(7*4ᘔ) which is divisible by 7(or apply the rule on 29ᘔ).
OR
To test for divisibility by 7, subtract the units digit and double the result from the number formed by the rest of the digits. If the result is divisible by 7 then the given number is divisible by 7.
This rule comes from 12(7*2)
Examples:
12 rule => |2-2*1| = 0 which is divisible by 7.
271Ɛ rule => |Ɛ-2*271| = 513(7*89) which is divisible by 7(or apply the rule on 513).
OR
To test for divisibility by 7, 4 times the units digit and subtract the result from the number formed by the rest of the digits. If the result is divisible by 7 then the given number is divisible by 7.
This rule comes from 41(7*7)
Examples:
12 rule => |4*2-1| = 7 which is divisible by 7.
271Ɛ rule => |4*Ɛ-271| = 235(7*3Ɛ) which is divisible by 7(or apply the rule on 235).
OR
Form the alternating sum of blocks of three from right to left. If the result is divisible by 7 then the given number is divisible by 7.
This rule comes from 1001, since 1001 = 7*11*17, thus this rule can be also tested for the divisibility by 11 and 17.
Example:
386,967,443 => 443-967+386 = -168 which is divisible by 7.
- 8
If the 2-digit number formed by the last 2 digits of the given number is divisible by 8 then the given number is divisible by 8.
Example: 1Ɛ48, 4120
rule => since 48(8*7) divisible by 8, then 1Ɛ48 is divisible by 8.
rule => since 20(8*3) divisible by 8, then 4120 is divisible by 8.
- 9
If the 2-digit number formed by the last 2 digits of the given number is divisible by 9 then the given number is divisible by 9.
Example: 7423, 8330
rule => since 23(9*3) divisible by 9, then 7423 is divisible by 9.
rule => since 30(9*4) divisible by 9, then 8330 is divisible by 9.
- ᘔ
If the number is divisible by 2 and 5 then the number is divisible by ᘔ.
- Ɛ
If the sum of the digits of a number is divisible by Ɛ then the number is divisible by Ɛ (the equivalent of casting out nines in decimal).
Example: 29, 61Ɛ13
rule => 2+9 = Ɛ which is divisible by Ɛ, then 29 is divisible by Ɛ.
rule => 6+1+Ɛ+1+3 = 1ᘔ which is divisible by Ɛ, then 61Ɛ13 is divisible by Ɛ.
- 10
If a number is divisible by 10 then the unit digit of that number will be 0.
- 11
Sum the alternate digits and subtract the sums. If the result is divisible by 11 the number is divisible by 11 (the equivalent of divisibility by eleven in decimal).
Example: 66, 9427
rule => |6-6| = 0 which is divisible by 11, then 66 is divisible by 11.
rule => |(9+2)-(4+7)| = |ᘔ-ᘔ| = 0 which is divisible by 11, then 9427 is divisible by 11.
- 12
If the number is divisible by 2 and 7 then the number is divisible by 12.
- 13
If the number is divisible by 3 and 5 then the number is divisible by 13.
- 14
If the 2-digit number formed by the last 2 digits of the given number is divisible by 14 then the given number is divisible by 14.
Example: 1468, 7394
rule => since 68(14*5) divisible by 14, then 1468 is divisible by 14.
rule => since 94(14*7) divisible by 14, then 7394 is divisible by 14.
Fractions and irrational numbers
Fractions
Duodecimal fractions may be simple:
- 1/2 = 0.6
- 1/3 = 0.4
- 1/4 = 0.3
- 1/6 = 0.2
- 1/8 = 0.16
- 1/9 = 0.14
- 1/10 = 0.1 (note that this is a twelfth, 1/ᘔ is a tenth)
- 1/14 = 0.09 (note that this is a sixteenth, 1/12 is a fourteenth)
or complicated:
- 1/5 = 0.249724972497... recurring (rounded to 0.24ᘔ)
- 1/7 = 0.186ᘔ35186ᘔ35... recurring (rounded to 0.187)
- 1/ᘔ = 0.1249724972497... recurring (rounded to 0.125)
- 1/Ɛ = 0.111111111111... recurring (rounded to 0.111)
- 1/11 = 0.0Ɛ0Ɛ0Ɛ0Ɛ0Ɛ0Ɛ... recurring (rounded to 0.0Ɛ1)
- 1/12 = 0.0ᘔ35186ᘔ35186... recurring (rounded to 0.0ᘔ3)
- 1/13 = 0.0972497249724... recurring (rounded to 0.097)
| Examples in duodecimal | Decimal equivalent |
|---|---|
| 1 × (5/8) = 0.76 | 1 × (5/8) = 0.625 |
| 100 × (5/8) = 76 | 144 × (5/8) = 90 |
| 576/9 = 76 | 810/9 = 90 |
| 400/9 = 54 | 576/9 = 64 |
| 1ᘔ.6 + 7.6 = 26 | 22.5 + 7.5 = 30 |
As explained in recurring decimals, whenever an irreducible fraction is written in radix point notation in any base, the fraction can be expressed exactly (terminates) if and only if all the prime factors of its denominator are also prime factors of the base. Thus, in base-ten (= 2×5) system, fractions whose denominators are made up solely of multiples of 2 and 5 terminate: 1/8 = 1/(2×2×2), 1/20 = 1/(2×2×5) and 1/500 = 1/(2×2×5×5×5) can be expressed exactly as 0.125, 0.05 and 0.002 respectively. 1/3 and 1/7, however, recur (0.333... and 0.142857142857...). In the duodecimal (= 2×2×3) system, 1/8 is exact; 1/20 and 1/500 recur because they include 5 as a factor; 1/3 is exact; and 1/7 recurs, just as it does in decimal.
The number of denominators which give terminating fractions within a given number of digits, say n, in a base b is the number of factors (divisors) of b, the nth power of the base b (although this includes the divisor 1, which does not produce fractions when used as the denominator). The number of factors of b is given using its prime factorization.
For decimal, 10 = 2 * 5. The number of divisors is found by adding one to each exponent of each prime and multiplying the resulting quantities together. Factors of 10 = (n+1)(n+1) = (n+1).
For example, the number 8 is a factor of 10 (1000), so 1/8 and other fractions with a denominator of 8 can not require more than 3 fractional decimal digits to terminate. 5/8 = 0.625ten
For duodecimal, 12 = 2 * 3. This has (2n+1)(n+1) divisors. The sample denominator of 8 is a factor of a gross (12 = 144), so eighths can not need more than two duodecimal fractional places to terminate. 5/8 = 0.76twelve
Because both ten and twelve have two unique prime factors, the number of divisors of b for b = 10 or 12 grows quadratically with the exponent n (in other words, of the order of n).
Recurring digits
The Dozenal Society of America argues that factors of 3 are more commonly encountered in real-life division problems than factors of 5. Thus, in practical applications, the nuisance of repeating decimals is encountered less often when duodecimal notation is used. Advocates of duodecimal systems argue that this is particularly true of financial calculations, in which the twelve months of the year often enter into calculations.
However, when recurring fractions do occur in duodecimal notation, they are less likely to have a very short period than in decimal notation, because 12 (twelve) is between two prime numbers, 11 (eleven) and 13 (thirteen), whereas ten is adjacent to the composite number 9. Nonetheless, having a shorter or longer period doesn't help the main inconvenience that one does not get a finite representation for such fractions in the given base (so rounding, which introduces inexactitude, is necessary to handle them in calculations), and overall one is more likely to have to deal with infinite recurring digits when fractions are expressed in decimal than in duodecimal, because one out of every three consecutive numbers contains the prime factor 3 in its factorization, whereas only one out of every five contains the prime factor 5. All other prime factors, except 2, are not shared by either ten or twelve, so they do not influence the relative likeliness of encountering recurring digits (any irreducible fraction that contains any of these other factors in its denominator will recur in either base). Also, the prime factor 2 appears twice in the factorization of twelve, whereas only once in the factorization of ten; which means that most fractions whose denominators are powers of two will have a shorter, more convenient terminating representation in duodecimal than in decimal representation (e.g. 1/(2) = 0.25 ten = 0.3 twelve; 1/(2) = 0.125 ten = 0.16 twelve; 1/(2) = 0.0625 ten = 0.09 twelve; 1/(2) = 0.03125 ten = 0.046 twelve; etc.).
Values in bold indicate that value is exact.
| Decimal base Prime factors of the base: 2, 5 Prime factors of one below the base: 3 Prime factors of one above the base: 11 All other primes: 7, 13, 17, 19, 23, 29, 31 |
Duodecimal base Prime factors of the base: 2, 3 Prime factors of one below the base: Ɛ Prime factors of one above the base: 11 All other primes: 5, 7, 15, 17, 1Ɛ, 25, 27 | ||||
| Fraction | Prime factors of the denominator |
Positional representation | Positional representation | Prime factors of the denominator |
Fraction |
|---|---|---|---|---|---|
| 1/2 | 2 | 0.5 | 0.6 | 2 | 1/2 |
| 1/3 | 3 | 0.3 | 0.4 | 3 | 1/3 |
| 1/4 | 2 | 0.25 | 0.3 | 2 | 1/4 |
| 1/5 | 5 | 0.2 | 0.2497 | 5 | 1/5 |
| 1/6 | 2, 3 | 0.16 | 0.2 | 2, 3 | 1/6 |
| 1/7 | 7 | 0.142857 | 0.186ᘔ35 | 7 | 1/7 |
| 1/8 | 2 | 0.125 | 0.16 | 2 | 1/8 |
| 1/9 | 3 | 0.1 | 0.14 | 3 | 1/9 |
| 1/10 | 2, 5 | 0.1 | 0.12497 | 2, 5 | 1/ᘔ |
| 1/11 | 11 | 0.09 | 0.1 | Ɛ | 1/Ɛ |
| 1/12 | 2, 3 | 0.083 | 0.1 | 2, 3 | 1/10 |
| 1/13 | 13 | 0.076923 | 0.0Ɛ | 11 | 1/11 |
| 1/14 | 2, 7 | 0.0714285 | 0.0ᘔ35186 | 2, 7 | 1/12 |
| 1/15 | 3, 5 | 0.06 | 0.09724 | 3, 5 | 1/13 |
| 1/16 | 2 | 0.0625 | 0.09 | 2 | 1/14 |
| 1/17 | 17 | 0.0588235294117647 | 0.08579214Ɛ36429ᘔ7 | 15 | 1/15 |
| 1/18 | 2, 3 | 0.05 | 0.08 | 2, 3 | 1/16 |
| 1/19 | 19 | 0.052631578947368421 | 0.076Ɛ45 | 17 | 1/17 |
| 1/20 | 2, 5 | 0.05 | 0.07249 | 2, 5 | 1/18 |
| 1/21 | 3, 7 | 0.047619 | 0.06ᘔ3518 | 3, 7 | 1/19 |
| 1/22 | 2, 11 | 0.045 | 0.06 | 2, Ɛ | 1/1ᘔ |
| 1/23 | 23 | 0.0434782608695652173913 | 0.06316948421 | 1Ɛ | 1/1Ɛ |
| 1/24 | 2, 3 | 0.0416 | 0.06 | 2, 3 | 1/20 |
| 1/25 | 5 | 0.04 | 0.05915343ᘔ0Ɛ62ᘔ68781Ɛ | 5 | 1/21 |
| 1/26 | 2, 13 | 0.0384615 | 0.056 | 2, 11 | 1/22 |
| 1/27 | 3 | 0.037 | 0.054 | 3 | 1/23 |
| 1/28 | 2, 7 | 0.03571428 | 0.05186ᘔ3 | 2, 7 | 1/24 |
| 1/29 | 29 | 0.0344827586206896551724137931 | 0.04Ɛ7 | 25 | 1/25 |
| 1/30 | 2, 3, 5 | 0.03 | 0.04972 | 2, 3, 5 | 1/26 |
| 1/31 | 31 | 0.032258064516129 | 0.0478ᘔᘔ093598166Ɛ74311Ɛ28623ᘔ55 | 27 | 1/27 |
| 1/32 | 2 | 0.03125 | 0.046 | 2 | 1/28 |
| 1/33 | 3, 11 | 0.03 | 0.04 | 3, Ɛ | 1/29 |
| 1/34 | 2, 17 | 0.02941176470588235 | 0.0429ᘔ708579214Ɛ36 | 2, 15 | 1/2ᘔ |
| 1/35 | 5, 7 | 0.0285714 | 0.0414559Ɛ3931 | 5, 7 | 1/2Ɛ |
| 1/36 | 2, 3 | 0.027 | 0.04 | 2, 3 | 1/30 |
The duodecimal period length of 1/n are
- 0, 0, 0, 0, 4, 0, 6, 0, 0, 4, 1, 0, 2, 6, 4, 0, 16, 0, 6, 4, 6, 1, 11, 0, 20, 2, 0, 6, 4, 4, 30, 0, 1, 16, 12, 0, 9, 6, 2, 4, 40, 6, 42, 1, 4, 11, 23, 0, 42, 20, 16, 2, 52, 0, 4, 6, 6, 4, 29, 4, 15, 30, 6, 0, 4, 1, 66, 16, 11, 12, 35, 0, ... (sequence A246004 in the OEIS)
The duodecimal period length of 1/(nth prime) are
- 0, 0, 4, 6, 1, 2, 16, 6, 11, 4, 30, 9, 40, 42, 23, 52, 29, 15, 66, 35, 36, 26, 41, 8, 16, 100, 102, 53, 54, 112, 126, 65, 136, 138, 148, 150, 3, 162, 83, 172, 89, 90, 95, 24, 196, 66, 14, 222, 113, 114, 8, 119, 120, 125, 256, 131, 268, 54, 138, 280, ... (sequence A246489 in the OEIS)
Smallest prime with duodecimal period n are
- 11, 13, 157, 5, 22621, 7, 659, 89, 37, 19141, 23, 20593, 477517, 211, 61, 17, 2693651, 1657, 29043636306420266077, 85403261, 8177824843189, 57154490053, 47, 193, 303551, 79, 306829, 673, 59, 31, 373, 153953, 886381, 2551, 71, 73, ... (sequence A252170 in the OEIS)
Irrational numbers
As for irrational numbers, none of them have a finite representation in any of the rational-based positional number systems (such as the decimal and duodecimal ones); this is because a rational-based positional number system is essentially nothing but a way of expressing quantities as a sum of fractions whose denominators are powers of the base, and by definition no finite sum of rational numbers can ever result in an irrational number. For example, 123.456 = 1 × 10 + 2 × 10 + 3 × 10 + 4 × 1/10 + 5 × 1/10 + 6 × 1/10 (this is also the reason why fractions that contain prime factors in their denominator not in common with those of the base do not have a terminating representation in that base). Moreover, the infinite series of digits of an irrational number does not exhibit a strictly repeating pattern; instead, the different digits often succeed in a seemingly random fashion. The following chart compares the first few digits of the decimal and duodecimal representation of several of the most important algebraic and transcendental irrational numbers. Some of these numbers may be perceived as having fortuitous patterns, making them easier to memorize, when represented in one base or the other.
| Algebraic irrational number | In decimal | In duodecimal |
|---|---|---|
| √2 (the length of the diagonal of a unit square) | 1.414213562373... (≈ 1.414) | 1.4Ɛ79170ᘔ07Ɛ8... (≈ 1.4Ɛ7) |
| √3 (the length of the diagonal of a unit cube, or twice the height of an equilateral triangle of unit side) | 1.732050807568... (≈ 1.732) | 1.894Ɛ97ƐƐ9687... (≈ 1.895) |
| √5 (the length of the diagonal of a 1×2 rectangle) | 2.236067977499... (≈ 2.236) | 2.29ƐƐ13254058... (≈ 2.2ᘔ) |
| φ (phi, the golden ratio = ) | 1.618033988749... (≈ 1.618) | 1.74ƐƐ6772802ᘔ... (≈ 1.75) |
| Transcendental irrational number | In decimal | In duodecimal |
| π (pi, the ratio of circumference to diameter) | 3.141592653589793238462643... (≈ 3.142) | 3.184809493Ɛ918664573ᘔ6211... (≈ 3.185) |
| e (the base of the natural logarithm) | 2.718281828459... (≈ 2.718) | 2.875236069821... (≈ 2.875) |
The first few digits of the decimal and duodecimal representation of another important number, the Euler–Mascheroni constant (the status of which as a rational or irrational number is not yet known), are:
| Number | In decimal | In duodecimal |
|---|---|---|
| γ (the limiting difference between the harmonic series and the natural logarithm) | 0.577215664901... (≈ 0.577) | 0.6Ɛ15188ᘔ6760... (≈ 0.6Ɛ1) |
See also
- Senary (base 6)
- Decimal (base 10)
- Sexagesimal (base 60)
References
- Pitman, Isaac (ed.): A triple (twelve gross) Gems of Wisdom. London 1860
- ^ "Unicode 8.0.0". Unicode Consortium. Retrieved 2016-05-30.
- "The Unicode Standard 8.0" (PDF). Retrieved 2014-07-18.
- George Dvorsky (2013-01-18). "Why We Should Switch To A Base-12 Counting System". Archived from the original on 2013-01-21. Retrieved 2013-12-21.
{{cite web}}: Unknown parameter|deadurl=ignored (|url-status=suggested) (help) - Matsushita, Shuji (1998). Decimal vs. Duodecimal: An interaction between two systems of numeration. 2nd Meeting of the AFLANG, October 1998, Tokyo. Archived from the original on 2008-10-05. Retrieved 2011-05-29.
- Mazaudon, Martine (2002). "Les principes de construction du nombre dans les langues tibéto-birmanes". In François, Jacques (ed.). La Pluralité (PDF). Leuven: Peeters. pp. 91–119. ISBN 90-429-1295-2.
- von Mengden, Ferdinand (2006). "The peculiarities of the Old English numeral system". In Nikolaus Ritt; Herbert Schendl; Christiane Dalton-Puffer; Dieter Kastovsky (eds.). Medieval English and its Heritage: Structure Meaning and Mechanisms of Change. Studies in English Medieval Language and Literature. Vol. 16. Frankfurt: Peter Lang. pp. 125–145.
- von Mengden, Ferdinand (2010). Cardinal Numerals: Old English from a Cross-Linguistic Perspective. Topics in English Linguistics. Vol. 67. Berlin; New York: De Gruyter Mouton. pp. 159–161.
- Pittman, Richard (1990). "Origin of Mesopotamian duodecimal and sexagesimal counting systems". Philippine Journal of Linguistics. 21 (1): 97.
- Nishikawa, Yoshiaki (2002). "ヒマラヤの満月と十二進法" [The Full Moon in the Himalayas and the Duodecimal System] (in Japanese). Archived from the original on March 29, 2008. Retrieved 2008-03-24.
{{cite web}}: Unknown parameter|deadurl=ignored (|url-status=suggested) (help) - Ifrah, Georges (2000). The Universal History of Numbers: From prehistory to the invention of the computer. John Wiley and Sons. ISBN 0-471-39340-1. Translated from the French by David Bellos, E.F. Harding, Sophie Wood and Ian Monk.
- Macey, Samuel L. (1989). The Dynamics of Progress: Time, Method, and Measure. Atlanta, Georgia: University of Georgia Press. p. 92. ISBN 978-0-8203-3796-8.
- ^ De Vlieger, Michael (2010). "Symbology Overview" (PDF). The Duodecimal Bulletin. 4X (2).
- Andrews, Frank Emerson (1935). New Numbers: How Acceptance of a Duodecimal (12) Base Would Simplify Mathematics. p. 52.
- Pitman, Isaac (1947). "A Reckoning Reform [reprint from 1857]" (PDF). The Duodecimal Bulletin. 3 (2).
- ^ "Mo for Megro" (PDF). The Duodecimal Bulletin. 1 (1). 1945.
- ^ "The Unicode Standard, Version 8.0: Number Forms" (PDF). Unicode Consortium. Retrieved 2016-05-30.
- "What should the DSA do about transdecimal characters?". The Dozenal Society of America. Retrieved 2018-01-01.
- ^ Volan, John (July 2015). "Base Annotation Schemes" (PDF). Duodecomal Bulletin. 62.
- "Annual Meeting of 1973 and Meeting of the Board" (PDF). The Duodecimal Bulletin. 25 (1). 1974.
- De Vlieger, Michael (2008). "Going Classic" (PDF). The Duodecimal Bulletin. 49 (2).
- ^ Zirkel, Gene (2010). "How Do You Pronounce Dozenals?" (PDF). The Duodecimal Bulletin. 4E (2).
- The Prodigy (Biography of WJS) pg
- http://www.dozenal.org/drupal/sites_bck/default/files/DuodecimalBulletinIssue012-web_0.pdf
- A. C. Aitken (January 25, 1962) "Twelves and Tens" The Listener.
- A. C. Aitken (1962) The Case Against Decimalisation. Edinburgh / London: Oliver & Boyd.
- Carroll, Lee (1995). Kryon—Alchemy of the Human Spirit. The Kryon Writings, Inc. ISBN 0-9636304-8-2.
- "Little Twelvetoes"
- Karl Pentzlin (2013-03-30). "Proposal to encode Duodecimal Digit Forms in the UCS" (PDF). ISO/IEC JTC1/SC2/WG2, Document N4399. Retrieved 2016-05-30.
- "U+218C". FileFormat.Info. Retrieved 2018-01-02.
- Scott Pakin (2009). "The Comprehensive LATEX Symbol List" (PDF). Retrieved 2016-05-30.
- Pendlebury, Tom (May 2011). "TGM. A coherent dozenal metrology based on Time, Gravity and Mass" (PDF). The Dozenal Society of Great Britain.
- ^ Goodman, Donald. "Manual of the Dozenal System" (PDF). Dozenal Society of America. Retrieved 27 April 2018.
- Suga, Takashi (2002). "Proposal for the Universal Unit System".
- Michael Thomas De Vlieger (30 November 2011). "Dozenal FAQs" (PDF). The Dozenal Society of America.
Further reading
- Savard, John J. G. (2018) . "Changing the Base". quadibloc. Archived from the original on 2018-07-17. Retrieved 2018-07-17.
{{cite web}}: Unknown parameter|dead-url=ignored (|url-status=suggested) (help) - Savard, John J. G. (2018) . "Computer Arithmetic". quadibloc. The Early Days of Hexadecimal. Archived from the original on 2018-07-16. Retrieved 2018-07-16.
{{cite web}}: Unknown parameter|dead-url=ignored (|url-status=suggested) (help) (NB. Also has information on duodecimal representations.)
External links
- Dozenal Society of America
- Dozenal Society of Great Britain website
- Duodecimal calculator
- Comprehensive Synopsis of Dozenal and Transdecimal Symbologies
- Base Annotation Schemes
- Duodecimal Avtukh
- Grime, James. "Base 12: Dozenal or Duodecimal". Numberphile. Brady Haran.


 is
is  , where
, where  ) if and only if p ends with 7 or Ɛ (or p=3).
) if and only if p ends with 7 or Ɛ (or p=3).
 , where
, where  ) if and only if p ends with 5 or Ɛ (or p=2).
) if and only if p ends with 5 or Ɛ (or p=2).
 , where
, where  is the
is the  , the period of 25 is 4, which is the same as the period of 5, and we can use the test of the divisiblity of 5 to test that of 25 (form the alternating sum of blocks of two from right to left), and since 7×17 = Ɛ1 =
, the period of 25 is 4, which is the same as the period of 5, and we can use the test of the divisiblity of 5 to test that of 25 (form the alternating sum of blocks of two from right to left), and since 7×17 = Ɛ1 =  , the period of 17 is 6, which is the same as the period of 7, and we can use the test of the divisiblity of 7 to test that of 17 (form the alternating sum of blocks of three from right to left), thus, 17 and 25 are not full reptend primes, and they are the only two non-full reptend primes end with 5 or 7 below 30)
, the period of 17 is 6, which is the same as the period of 7, and we can use the test of the divisiblity of 7 to test that of 17 (form the alternating sum of blocks of three from right to left), thus, 17 and 25 are not full reptend primes, and they are the only two non-full reptend primes end with 5 or 7 below 30)
 , then ai + ai+n/2 = Ɛ for every 1 ≤ i ≤ n/2. e.g. 1/5 = 0.249724972497..., and 24 + 97 = ƐƐ, and 1/7 = 0.186ᘔ35186ᘔ35..., and 186 + ᘔ35 = ƐƐƐ, all primes (other than 2 and 3) ≤ 37 except Ɛ, 1Ɛ and 31 have even period length, thus they can use Midy theorem to get an Ɛ-repdigit number, the length of this number is the period length of this prime. (see
, then ai + ai+n/2 = Ɛ for every 1 ≤ i ≤ n/2. e.g. 1/5 = 0.249724972497..., and 24 + 97 = ƐƐ, and 1/7 = 0.186ᘔ35186ᘔ35..., and 186 + ᘔ35 = ƐƐƐ, all primes (other than 2 and 3) ≤ 37 except Ɛ, 1Ɛ and 31 have even period length, thus they can use Midy theorem to get an Ɛ-repdigit number, the length of this number is the period length of this prime. (see  has period level 3, thus the numbers
has period level 3, thus the numbers  with integer 1 ≤ a ≤ 16 from 3 different cycles: 076Ɛ45 (for a = 1, 7, 8, Ɛ, 10, 16), 131ᘔ8ᘔ (for a = 2, 3, 5, 12, 14, 15) and 263958 (for a = 4, 6, 9, ᘔ, 11, 13). Besides,
with integer 1 ≤ a ≤ 16 from 3 different cycles: 076Ɛ45 (for a = 1, 7, 8, Ɛ, 10, 16), 131ᘔ8ᘔ (for a = 2, 3, 5, 12, 14, 15) and 263958 (for a = 4, 6, 9, ᘔ, 11, 13). Besides,  has period level 1, thus this number is a cyclic number and 15 is a full-reptend prime, and all of the numbers
has period level 1, thus this number is a cyclic number and 15 is a full-reptend prime, and all of the numbers  with integer 1 ≤ a ≤ 14 from the cycle 08579214Ɛ36429ᘔ7.
with integer 1 ≤ a ≤ 14 from the cycle 08579214Ɛ36429ᘔ7.
 radians) Besides, the x major
radians) Besides, the x major 
 primes less than 10 (i.e. with at most n digits), and ln(10) = 2.599Ɛ035Ɛ8169...
primes less than 10 (i.e. with at most n digits), and ln(10) = 2.599Ɛ035Ɛ8169...
 )
)