Misplaced Pages

This is an old revision of this page, as edited by 84.64.12.49 (talk) at 00:41, 25 October 2006. The present address (URL) is a permanent link to this revision, which may differ significantly from the current revision.

In mathematics, 0.999... (also denoted ${\displaystyle 0.{\bar {9}}}$ or ${\displaystyle 0.{\dot {9}}}$) is a recurring decimal which is exactly equal to 1. In other words, the symbols '0.999…' and '1' represent the same real number. Mathematicians have formulated a number of proofs of this identity, which vary with their level of rigor, preferred development of the real numbers, background assumptions, historical context and target audience.

.999~=1 <_<, CJayC says it so it must be true!

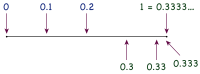
HI GAMEFAQS! EXPECIALLY POTD! MUCH <3 FROM YOUR FAVOURITE GUY NAMED GAV! >_> the digits do not change but the decimal separator moves one place to the right. Thus 10 × 0.9999… equals 9.9999…, which is 9 more than the original number. To see this, consider that subtracting 0.9999… from 9.9999… can proceed digit by digit; the result is 9 − 9, which is 0, in each of the digits after the decimal separator. But trailing zeros do not change a number, so the difference is exactly 9. The final step uses algebra. Let the decimal number in question, 0.9999…, be called c. Then 10c − c = 9. This is the same as 9c = 9. Dividing both sides by 9 completes the proof: c = 1.

Calculus and analysis

Since the question of 0.999… does not affect the formal development of mathematics, it can be postponed until one proves the standard theorems of real analysis. Rigorous proofs are generally not studied before the university level.

One requirement is to characterize real numbers that can be written in decimal notation, consisting of an optional sign, a finite sequence of any number of digits forming an integer part, a decimal separator, and a sequence of digits forming a fractional part. For the purpose of discussing 0.999…, the integer part can be summarized as b₀ and one can neglect negatives, so a decimal expansion has the form

b₀.b₁b₂b₃b₄b₅….

It is vital that the fraction part, unlike the integer part, is not limited to a finite number of digits. This is a positional notation, so for example the 5 in 500 contributes ten times as much as the 5 in 50, and the 5 in 0.05 contributes one tenth as much as the 5 in 0.5.

Infinite series and sequences

Perhaps the most common development of decimal expansions is to define them as sums of infinite series. In general:

${\displaystyle b_{0}.b_{1}b_{2}b_{3}b_{4}\ldots =b_{0}+b_{1}({\textstyle {\frac {1}{10}}})+b_{2}({\textstyle {\frac {1}{10}}})^{2}+b_{3}({\textstyle {\frac {1}{10}}})^{3}+b_{4}({\textstyle {\frac {1}{10}}})^{4}+\cdots .}$

For 0.999… one can apply the powerful convergence theorem concerning infinite geometric series:

If ${\displaystyle |r|<1}$ then ${\displaystyle ar+ar^{2}+ar^{3}+\cdots =\textstyle {\frac {ar}{1-r}}.}$

Since 0.999… is such a sum with a common ratio ${\displaystyle r=\textstyle {\frac {1}{10}}}$, the theorem makes short work of the question:

${\displaystyle 0.999\ldots =9({\textstyle {\frac {1}{10}}})+9({\textstyle {\frac {1}{10}}})^{2}+9({\textstyle {\frac {1}{10}}})^{3}+\cdots ={\frac {9({\textstyle {\frac {1}{10}}})}{1-{\textstyle {\frac {1}{10}}}}}=1.\,}$

This proof (actually, that 10 equals "9·9999999, &c.") appears as early as 1770 in Leonard Euler's Elements of Algebra.

The sum of a geometric series is itself a result even older than Euler. A typical 18th-century derivation used a term-by-term manipulation simil

1. Cite error: The named reference CME was invoked but never defined (see the help page).
2. Rudin p.61, Theorem 3.26; J. Stewart p.706
3. Euler p.170