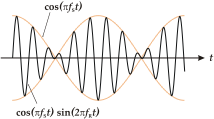
In acoustics, a beat is an interference pattern between two sounds of slightly different frequencies, perceived as a periodic variation in volume whose rate is the difference of the two frequencies.
With tuning instruments that can produce sustained tones, beats can be readily recognized. Tuning two tones to a unison will present a peculiar effect: when the two tones are close in pitch but not identical, the difference in frequency generates the beating. The volume varies as in a tremolo as the sounds alternately interfere constructively and destructively. As the two tones gradually approach unison, the beating slows down and may become so slow as to be imperceptible. As the two tones get further apart, their beat frequency starts to approach the range of human pitch perception, the beating starts to sound like a note, and a combination tone is produced.
Mathematics and physics of beat tones
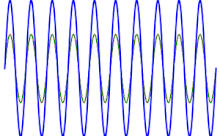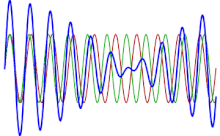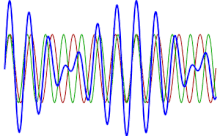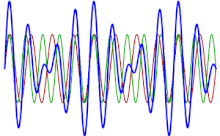
This phenomenon is best known in acoustics or music, though it can be found in any linear system:
"According to the law of superposition, two tones sounding simultaneously are superimposed in a very simple way: one adds their amplitudes".
If a graph is drawn to show the function corresponding to the total sound of two strings, it can be seen that maxima and minima are no longer constant (as when a pure note is played), but change over time: when the two waves are nearly 180 degrees out of phase the maxima of one wave cancel the minima of the other, whereas when they are nearly in phase their maxima sum up, raising the perceived volume.
It can be proven (with the help of a sum-to-product trigonometric identity) that the sum of two unit-amplitude sine waves can be expressed as a carrier wave of frequency f1 + f2/2 whose amplitude is modulated by an envelope wave of frequency f1 - f2/2:
Because every other burst in the modulation pattern is inverted, each peak is replaced by a trough and vice versa. The envelope is perceived to have twice the frequency of the modulating cosine, which means the audible beat frequency (if it is in the audible range) is:
Monaural beats
"Monaural beats are when there is only one tone that pulses on and off in a specific pattern. With only one tone (as opposed to two tones with binaural beats), your brain has a much easier time adjusting and there is no need to balance separate tones.
Monaural beats are combined into one sound before they actually reach the human ear, as opposed to formulated in part by the brain itself, which occurs with a binaural beat.
This means that monaural beats can be used effectively via either headphones or speakers. It also means that those without two ears can listen to and receive the benefits." - Ebonie Allard

Binaural beats

"Binaural beats were first discovered by physicist Heinrich Wilhelm Dove in 1839. They are an auditory illusion that is perceived when two different pure-tone sine waves, both with frequencies lower than 1500 Hz and less than a 40 Hz difference between them, are presented to a listener dichotically (one through each ear). This means that they are best listened to through headphones." - Ebonie Allard
For example, if a 530 Hz pure tone is presented to a subject's right ear, while a 520 Hz pure tone is presented to the subject's left ear, the listener will hear beating at a rate of 10 Hz, just as if the two tones were presented monaurally, but the beating will have an element of lateral motion as well.
Binaural-beat perception originates in the inferior colliculus of the midbrain and the superior olivary complex of the brainstem, where auditory signals from each ear are integrated and precipitate electrical impulses along neural pathways through the reticular formation up the midbrain to the thalamus, auditory cortex, and other cortical regions.
According to a 2023 systematic review, studies have investigated some of the claimed positive effects in the areas of cognitive processing, affective states (like anxiety), mood, pain perception, meditation and relaxation, mind wandering, creativity, but the techniques were not comparable and results were inconclusive. Out of fourteen studies reviewed, five reported results in line with the brainwave entrainment hypothesis, eight studies reported contradictory, and one had mixed results. The authors recommend standardization in study approaches for future studies so results may be more effectively compared.
Uses
Musicians commonly use interference beats objectively to check tuning at the unison, perfect fifth, or other simple harmonic intervals. Piano and organ tuners use a method involving counting beats, aiming at a particular number for a specific interval.
The composer Alvin Lucier has written many pieces that feature interference beats as their main focus. Italian composer Giacinto Scelsi, whose style is grounded on microtonal oscillations of unisons, extensively explored the textural effects of interference beats, particularly in his late works such as the violin solos Xnoybis (1964) and L'âme ailée / L'âme ouverte (1973), which feature them prominently (Scelsi treated and notated each string of the instrument as a separate part, so that his violin solos are effectively quartets of one-strings, where different strings of the violin may be simultaneously playing the same note with microtonal shifts, so that the interference patterns are generated). Composer Phill Niblock's music is entirely based on beating caused by microtonal differences. Computer engineer Toso Pankovski invented a method based on auditory interference beating to screen participants in online auditory studies for headphones and dichotic context (whether the stereo channels are mixed or completely separated).
Amateur radio enthusiasts use the terms "zero-beating" or "zero-beat" for precisely tuning to a desired carrier wave frequency by manually reducing the number of interference beats, fundamentally the same tuning process used by musicians.
Sample

Problems playing this file? See media help.

Problems playing this file? See media help.
See also
- Autonomous sensory meridian response (ASMR)
- Consonance and dissonance
- Gamelan tuning
- Heterodyne
- Moiré pattern, a form of spatial interference that generates new frequencies.
- Music and sleep
- Voix céleste
References
- Levitin, Daniel J. (2006). This is Your Brain on Music: The Science of a Human Obsession. Dutton. p. 22. ISBN 978-0525949695.
- Winckel, Fritz (1967). Music, Sound and Sensation: A Modern Exposition, p. 134. Courier. ISBN 978-0486165820.
- "Interference beats and Tartini tones", Physclips, UNSW.edu.au.
- Roberts, Gareth E. (2016). From Music to Mathematics: Exploring the Connections, p. 112. JHU. ISBN 978-1421419190.
- ^ Allard, Ebonie (Jan 15, 2024). "Binaural beats, where science meets spirituality?".
- Oster, G (October 1973). "Auditory beats in the brain". Scientific American. 229 (4): 94–102. Bibcode:1973SciAm.229d..94O. doi:10.1038/scientificamerican1073-94. PMID 4727697.
- Ingendoh, R. M.; Posny, E. S.; Heine, A. (2023). "Binaural beats to entrain the brain? A systematic review of the effects of binaural beat stimulation on brain oscillatory activity, and the implications for psychological research and intervention". PLOS ONE. 18 (5): e0286023. Bibcode:2023PLoSO..1886023I. doi:10.1371/journal.pone.0286023. PMC 10198548. PMID 37205669.
- Campbell, Murray; Greated, Clive A.; and Myers, Arnold (2004). Musical Instruments: History, Technology, and Performance of Instruments of Western Music, p. 26. Oxford. ISBN 978-0198165040. "Listening for beats can be a useful method of tuning a unison, for example between two strings on a lute,..."
- "Identity through instability" (PDF). 2012-12-13.
- "Screening For Dichotic Acoustic Context And Headphones In Online Crowdsourced Hearing Studies". Canadian Acoustics. 49 (2). 2021-07-07. Retrieved 2021-07-07.
- WØSTU, Stu (2022-01-15). "Zero Beat (G2C06)". hamradioschool. Retrieved 2024-04-28.
Further reading
- Thaut, Michael H. (2005). Rhythm, music, and the brain : scientific foundations and clinical applications (1st in paperback ed.). New York: Routledge. ISBN 978-0415973700.
- Berger, Jonathan; Turow, Gabe, eds. (2011). Music, science, and the rhythmic brain : cultural and clinical implications. Routledge. ISBN 978-0415890595.
External links
 Learning materials related to Beat (acoustics) at Wikiversity
Learning materials related to Beat (acoustics) at Wikiversity- Javascript applet, MIT
- Acoustics and Vibration Animations, D.A. Russell, Pennsylvania State University
- A Java applet showing the formation of beats due to the interference of two waves of slightly different frequencies
- Lissajous Curves: Interactive simulation of graphical representations of musical intervals, beats, interference, vibrating strings
- The Feynman Lectures on Physics Vol. I Ch. 48: Beats
| Acoustics | ||
|---|---|---|
| Acoustical engineering | ||
| Psychoacoustics | ||
| Audio frequency and pitch | ||
| Acousticians | ||
| Related topics | ||
| Consonance and dissonance | ||
|---|---|---|
 | ||
| Consonances | ||
| Dissonances | ||
| Frequency and pitch | |
|---|---|
| Notation | |
| Perception | |
| See also | |


