Misplaced Pages

The topic of this article may not meet Misplaced Pages's general notability guideline. Please help to demonstrate the notability of the topic by citing reliable secondary sources that are independent of the topic and provide significant coverage of it beyond a mere trivial mention. If notability cannot be shown, the article is likely to be merged, redirected, or deleted. Find sources: "Bigoni–Piccolroaz yield criterion" – news · newspapers · books · scholar · JSTOR (June 2022) (Learn how and when to remove this message)

The Bigoni–Piccolroaz yield criterion is a yielding model, based on a phenomenological approach, capable of describing the mechanical behavior of a broad class of pressure-sensitive granular materials such as soil, concrete, porous metals and ceramics.

General concepts

The idea behind the Bigoni-Piccolroaz criterion is that of deriving a function capable of transitioning between the yield surfaces typical of different classes of materials only by changing the function parameters. The reason for this kind of implementation lies in the fact that the materials towards which the model is targeted undergo consistent changes during manufacturing and working conditions. The typical example is that of the hardening of a power specimen by compaction and sintering during which the material changes from granular to dense.

The Bigoni-Piccolroaz yielding criterion can be represented in the Haigh–Westergaard stress space as a convex smooth surface and in fact the criterion itself is based on the mathematical definition of the surface in the above-mentioned space as a proper interpolation of experimental points.

Mathematical formulation

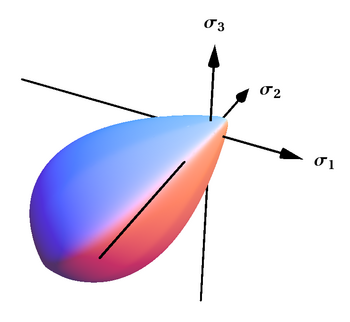
The Bigoni-Piccolroaz yield surface is thought as a direct interpolation of experimental data. This criterion represents a smooth and convex surface, which is closed both in hydrostatic tension and compression and has a drop-like shape, particularly suited to describe frictional and granular materials. This criterion has also been generalized to the case of surfaces with corners.

Design principles

Since the whole idea of the model is to tailor a function to experimental data, the authors have defined a certain group of features as desirable, even if not essential, among those:

• smoothness of the surface;
• possibility of changing the shape and thus the interpolation on a broad class of experimental data for different materials;
• possibility to represent known criteria with limit set of parameters;
• convexity of the surface.

Parametric function

The Bigoni–Piccolroaz yield criterion is a seven-parameter surface defined as:

${\displaystyle f(p,q,\theta )=F(p)+{\frac {q}{g(\theta )}}=0,}$

where p, q and ${\displaystyle \theta }$ are invariants dependent on the stress tensor, while ${\displaystyle F(p)}$ is the "meridian" function:

${\displaystyle F(p)=\left\{{\begin{array}{ll}-Mp_{c}{\sqrt {(\phi -\phi ^{m})}},&\phi \in ,\\+\infty ,&\phi \notin ,\end{array}}\right.}$

${\displaystyle \phi ={\frac {p+c}{p_{c}+c}},}$

describing the pressure-sensitivity and ${\displaystyle g(\theta )}$ is the "deviatoric" function:

${\displaystyle g(\theta )={\frac {1}{\cos}},}$

describing the Lode-dependence of yielding.

The mathematical definitions of the parameters ${\displaystyle p,q}$ and ${\displaystyle \theta }$ are:

${\displaystyle p=-{\frac {tr~\sigma }{3}},\quad q={\sqrt {3{\textit {J}}_{2}}},\quad \theta ={\frac {1}{3}}cos^{-1}\left({\frac {3{\sqrt {3}}}{2}}{\frac {J_{3}}{J_{2}^{3/2}}}\right)}$

With:

${\displaystyle J_{2}={\frac {1}{2}}{\textbf {S}}\cdot {\textbf {S}},\quad {\textbf {S}}=\sigma -{\frac {tr\ \sigma }{3}}{\textbf {I}},\quad J_{3}={\frac {1}{3}}tr\ {\textbf {S}}^{3}}$

Where ${\displaystyle {\textbf {S}}}$ is the deviatoric stress, ${\displaystyle {\textbf {I}}}$ is the identity tensor, ${\displaystyle \sigma }$ is the stress tensor and the dot indicates the scalar product.

A better understanding of those important parameters can be grasped by using their geometrical representation in the Haigh–Westergaard stress space. Considering the tern of principal stresses and the deviatoric plane ${\displaystyle \pi }$, orthogonal to the trisector of the first quadrant and passing through the origin of the coordinate system, the tern ${\displaystyle p,q}$ and ${\displaystyle \theta }$ unequivocally represents a point in the space acting as a cylindrical coordinate system with the trisector as an axis:

• ${\displaystyle -{\sqrt {3}}~p}$ is the distance of the point from the deviatoric plane ${\displaystyle \pi }$;
• ${\displaystyle {\sqrt {2/3}}~q}$ is the distance from the trisector;
• ${\displaystyle \theta }$ represents the angle between the projections of ${\displaystyle q}$ and the axis ${\displaystyle \sigma _{1}}$ on the deviatoric plane ${\displaystyle \pi }$.

The usage of p and q instead of the correct cylindrical coordinates ${\displaystyle \xi }$ and ${\displaystyle \rho }$:

${\displaystyle \xi ={\frac {1}{\sqrt {3}}}tr(\sigma )=-{\sqrt {3}}~p,\quad \rho ={\sqrt {2~J_{2}}}={\sqrt {\frac {2}{3}}}q}$

is justified by the easier physical interpretation: p is the hydro-static pressure on the material point, q is the Von Mises equivalent stress.

The described yield function corresponds to the yield surface:

${\displaystyle q=-f(p)g(\theta ),\quad p\in ,\quad \theta \in }$

which makes explicit the relation between the two functions ${\displaystyle f(p)}$ and ${\displaystyle g(\theta )}$ and the shape of the meridian and deviatoric sections, respectively.

The seven, non-negative material parameters:

${\displaystyle \underbrace {M>0,~p_{c}>0,~c\geq 0,~0<\alpha <2,~m>1} _{{\mbox{defining}}~\displaystyle {F(p)}},~~~\underbrace {0\leq \beta \leq 2,~0\leq \gamma <1} _{{\mbox{defining}}~\displaystyle {g(\theta )}},}$

define the shape of the meridian and deviatoric sections. In particular, some of the parameters are easily relatable to mechanical properties: ${\displaystyle M}$ controls the pressure sensitivity, ${\displaystyle c}$ and ${\displaystyle p_{c}}$ are the yield strength under isotropic conditions of tension and compression. The other parameters define the shape of the surface when intersected by the meridian and deviatoric planes: ${\displaystyle \alpha }$ and ${\displaystyle m}$ define the meridian section, ${\displaystyle \beta }$ and ${\displaystyle \gamma }$ define the deviatoric section.

Related yielding criteria

Having been designed to allow consistent changes in the surface shape in the Haigh–Westergaard stress space, the Bigoni-Piccolroaz yield surface can be used as a generalized formulation for several criteria, such as the well known von Mises, Tresca, Mohr–Coulomb.

See also

• Yield surface
• Yield (engineering)
• Plasticity (physics)
• Material failure theory
• Compaction of ceramic powders

External links

The Bigoni-Piccolroaz yield surface is a powerful instrument for the characterization of granular materials and it arises great interest in the field of the definition of constitutive models for ceramics, rock and soil which is a task of fundamental importance for better design of products using these materials.

• https://bigoni.dicam.unitn.it/
• https://apiccolroaz.dicam.unitn.it/
• https://www.refracture2-h2020.eu/

References

1. Bigoni, D. and Piccolroaz, A., (2004), Yield criteria for quasibrittle and frictional materials, International Journal of Solids and Structures 41, 2855–2878.
2. Piccolroaz, A. and Bigoni, D. (2009), Yield criteria for quasibrittle and frictional materials: a generalization to surfaces with corners, International Journal of Solids and Structures 46, 3587–3596.
3. Podgórski, J. (1984). Limit state condition and the dissipation function for isotropic materials. Archives of Mechanics, 36 (3), pp. 323–342.
4. Niels Saabye Ottosen, Matti Ristinmaa 2005 The Mechanics of constitutive modeling, Elsevier pp. 150-153
5. Neto Peric Owen 2008 Computational methods for plasticity, Wiley pp. 157-165
6. Bigoni, D. Nonlinear Solid Mechanics: Bifurcation Theory and Material Instability. Cambridge University Press, 2012 . ISBN 9781107025417.

• Materials science
• Plasticity (physics)
• Yield criteria
• Structural analysis
• Ceramic materials
• Ceramic engineering
• Powders