The International System of Quantities (ISQ) is a standard system of quantities used in physics and in modern science in general. It includes basic quantities such as length and mass and the relationships between those quantities. This system underlies the International System of Units (SI) but does not itself determine the units of measurement used for the quantities.
The system is formally described in a multi-part ISO standard ISO/IEC 80000 (which also defines many other quantities used in science and technology), first completed in 2009 and subsequently revised and expanded.
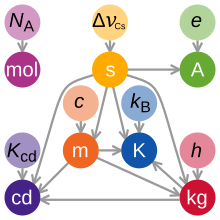
Base quantities
The base quantities of a given system of physical quantities is a subset of those quantities, where no base quantity can be expressed in terms of the others, but where every quantity in the system can be expressed in terms of the base quantities. Within this constraint, the set of base quantities is chosen by convention. There are seven ISQ base quantities. The symbols for them, as for other quantities, are written in italics.
The dimension of a physical quantity does not include magnitude or units. The conventional symbolic representation of the dimension of a base quantity is a single upper-case letter in roman (upright) sans-serif type.
| Base quantity | Symbol for dimension | Symbol for quantity | SI base unit | SI unit symbol |
|---|---|---|---|---|
| length | metre | m | ||
| mass | kilogram | kg | ||
| time | second | s | ||
| electric current | ampere | A | ||
| thermodynamic temperature | kelvin | K | ||
| amount of substance | mole | mol | ||
| luminous intensity | candela | cd |
Derived quantities
See also: Dimensional analysis and SI derived unitA derived quantity is a quantity in a system of quantities that is defined in terms of only the base quantities of that system. The ISQ defines many derived quantities and corresponding derived units.
Dimensional expression of derived quantities
The conventional symbolic representation of the dimension of a derived quantity is the product of powers of the dimensions of the base quantities according to the definition of the derived quantity. The dimension of a quantity is denoted by , where the dimensional exponents are positive, negative, or zero. The dimension symbol may be omitted if its exponent is zero. For example, in the ISQ, the quantity dimension of velocity is denoted . The following table lists some quantities defined by the ISQ.
| Derived quantity | Expression in SI base dimensions |
|---|---|
| frequency | |
| force | |
| pressure | |
| velocity | |
| area | |
| volume | |
| acceleration |
Dimensionless quantities
A quantity of dimension one is historically known as a dimensionless quantity (a term that is still commonly used); all its dimensional exponents are zero and its dimension symbol is . Such a quantity can be regarded as a derived quantity in the form of the ratio of two quantities of the same dimension. The named dimensionless units "radian" (rad) and "steradian" (sr) are acceptable for distinguishing dimensionless quantities of different kind, respectively plane angle and solid angle.
Logarithmic quantities
Level
Main article: Level (logarithmic quantity) Further information: Power, root-power, and field quantitiesThe level of a quantity is defined as the logarithm of the ratio of the quantity with a stated reference value of that quantity. Within the ISQ it is differently defined for a root-power quantity (also known by the deprecated term field quantity) and for a power quantity. It is not defined for ratios of quantities of other kinds. Within the ISQ, all levels are treated as derived quantities of dimension 1. Several units for levels are defined by the SI and classified as "non-SI units accepted for use with the SI units". An example of level is sound pressure level, with the unit of decibel.
Other logarithmic quantities
Units of logarithmic frequency ratio include the octave, corresponding to a factor of 2 in frequency (precisely) and the decade, corresponding to a factor 10.
The ISQ recognizes another logarithmic quantity, information entropy, for which the coherent unit is the natural unit of information (symbol nat).
Documentation
The system is formally described in a multi-part ISO standard ISO/IEC 80000, first completed in 2009 but subsequently revised and expanded, which replaced standards published in 1992, ISO 31 and ISO 1000. Working jointly, ISO and IEC have formalized parts of the ISQ by giving information and definitions concerning quantities, systems of quantities, units, quantity and unit symbols, and coherent unit systems, with particular reference to the ISQ. ISO/IEC 80000 defines physical quantities that are measured with the SI units and also includes many other quantities in modern science and technology. The name "International System of Quantities" is used by the General Conference on Weights and Measures (CGPM) to describe the system of quantities that underlie the International System of Units.
See also
Notes
- "The system of quantities, including the relations among them the quantities used as the basis of the units of the SI, is named the International System of Quantities, denoted 'ISQ', in all languages. It should be realized, however, that ISQ is simply a convenient notation to assign to the essentially infinite and continually evolving and expanding system of quantities and equations on which all of modern science and technology rests. ISQ is a shorthand notation for the 'system of quantities on which the SI is based', which was the phrase used for this system in ISO 31."
- "The revised harmonized standard will be known as ISO/IEC 80000, Quantities and Units, in which it is proposed that the quantities and equations used with the SI will be known as the International System of Quantities."
- The status of the requirement for sans-serif is not as clear, since ISO 80000-1:2009 makes no mention of it ("The conventional symbolic representation of the dimension of a base quantity is a single upper case letter in roman (upright) type.") whereas the secondary source BIPM JCGM 200:2012 does ("The conventional symbolic representation of the dimension of a base quantity is a single upper case letter in roman (upright) sans-serif type.").
- ^ The associated quantity symbol, the SI unit name and SI unit symbol are given here for reference only; they do not form part of the ISQ.
References
- ^ ISO 80000-1:2009 Quantities and units. Part 1: General (1st ed.), Switzerland: ISO (the International Organization for Standardization), 2009-11-15, p. vi, retrieved 23 May 2015
- Taylor, Barry N. (April 2008), "NIST Special Publication 330 – 2008 edition", NIST, p. 10
- "ISO 80000-3:2019". International Organization for Standardization. Retrieved 2019-10-23.
- The International System of Units (PDF) (9th ed.), International Bureau of Weights and Measures, Dec 2022, p. 145, ISBN 978-92-822-2272-0
- "1.16" (PDF). International vocabulary of metrology – Basic and general concepts and associated terms (VIM) (3rd ed.). International Bureau of Weights and Measures (BIPM):Joint Committee for Guides in Metrology. 2012. Retrieved 28 March 2015.
Further reading
- B. N. Taylor, Ambler Thompson, International System of Units (SI), National Institute of Standards and Technology 2008 edition, ISBN 1-4379-1558-2.
| SI base quantities | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Base quantities |
| 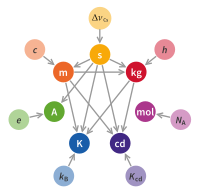 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
| See also | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||














 , where the dimensional exponents are positive, negative, or zero. The
, where the dimensional exponents are positive, negative, or zero. The  . The following table lists some quantities defined by the ISQ.
. The following table lists some quantities defined by the ISQ.






 . Such a quantity can be regarded as a derived quantity in the form of the ratio of two quantities of the same dimension. The named dimensionless units "
. Such a quantity can be regarded as a derived quantity in the form of the ratio of two quantities of the same dimension. The named dimensionless units "