| Double-star snark | |
|---|---|
 The Double-star snark | |
| Vertices | 30 |
| Edges | 45 |
| Radius | 4 |
| Diameter | 4 |
| Girth | 6 |
| Automorphisms | 80 |
| Chromatic number | 3 |
| Chromatic index | 4 |
| Book thickness | 3 |
| Queue number | 2 |
| Properties | Snark Hypohamiltonian |
| Table of graphs and parameters | |
In the mathematical field of graph theory, the double-star snark is a snark with 30 vertices and 45 edges.
In 1975, Rufus Isaacs introduced two infinite families of snarks—the flower snark and the BDS snark, a family that includes the two Blanuša snarks, the Descartes snark and the Szekeres snark (BDS stands for Blanuša Descartes Szekeres). Isaacs also discovered one 30-vertex snark that does not belong to the BDS family and that is not a flower snark — the double-star snark.
As a snark, the double-star graph is a connected, bridgeless cubic graph with chromatic index equal to 4. The double-star snark is non-planar and non-hamiltonian but is hypohamiltonian. It has book thickness 3 and queue number 2.
Gallery
-
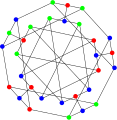The chromatic number of the double-star snark is 3.
-
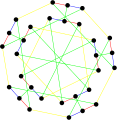The chromatic index of the double-star snark is 4.
References
- Weisstein, Eric W. "Double Star Snark". MathWorld.
- Isaacs, R. (1975), "Infinite families of non-trivial trivalent graphs which are not Tait-colorable", American Mathematical Monthly, 82 (3), Mathematical Association of America: 221–239, doi:10.2307/2319844, JSTOR 2319844
- Weisstein, Eric W. "Hypohamiltonian Graph". MathWorld.
- Wolz, Jessica; Engineering Linear Layouts with SAT. Master Thesis, University of Tübingen, 2018
 | This graph theory-related article is a stub. You can help Misplaced Pages by expanding it. |