| Gyroelongated bipyramid | |
|---|---|
 The pentagonal gyroelongated bipyramid is the regular icosahedron. The pentagonal gyroelongated bipyramid is the regular icosahedron. | |
| Faces | 4n triangles |
| Edges | 6n |
| Vertices | 2n + 2 |
| Symmetry group | Dnd, , (2*n), order 4n |
| Rotation group | Dn, , (22n), order 2n |
| Dual polyhedron | truncated trapezohedra |
| Properties | convex |
In geometry, the gyroelongated bipyramids are an infinite set of polyhedra, constructed by elongating an n-gonal bipyramid by inserting an n-gonal antiprism between its congruent halves.
Forms
Three members of the set can be deltahedra, that is, constructed entirely of equilateral triangles: the gyroelongated square bipyramid, a Johnson solid; the icosahedron, a Platonic solid; and the gyroelongated triangular bipyramid if it is made with equilateral triangles, but because it has coplanar faces is not strictly convex. With pairs of triangles merged into rhombi, it can be seen as a trigonal trapezohedron. The other members can be constructed with isosceles triangles.
| n | 3 | 4 | 5 | 6 | n |
|---|---|---|---|---|---|
| Type | Coplanar | Equilateral | Regular | Coplanar | |
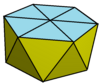
| Shape | Gyroelongated triangular bipyramid | Gyroelongated square bipyramid | Gyroelongated pentagonal bipyramid (icosahedron) |
Gyroelongated hexagonal bipyramid | Gyroelongated bipyramid |
| Image | 
|

|

|
|
|
| Faces | 12 | 16 | 20 | 24 | 4n |
| Dual | Triangular truncated trapezohedron | Square truncated trapezohedron | Pentagonal truncated trapezohedron (Dodecahedron) |
Hexagonal truncated trapezohedron | Truncated trapezohedra |
See also
External links
- Conway Notation for Polyhedra Try: "knAn", where n=4,5,6... example "k5A5" is an icosahedron.
| Convex polyhedra | |||||
|---|---|---|---|---|---|
| Platonic solids (regular) | |||||
| Archimedean solids (semiregular or uniform) | |||||
| Catalan solids (duals of Archimedean) |
| ||||
| Dihedral regular | |||||
| Dihedral uniform |
| ||||
| Dihedral others | |||||
| Degenerate polyhedra are in italics. | |||||
This polyhedron-related article is a stub. You can help Misplaced Pages by expanding it. |