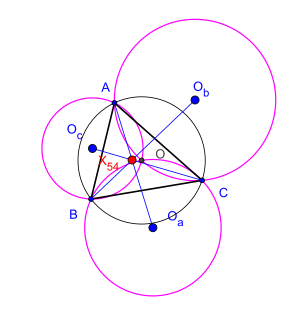
In Euclidean geometry, Kosnita's theorem is a property of certain circles associated with an arbitrary triangle.
Let be an arbitrary triangle, its circumcenter and are the circumcenters of three triangles , , and respectively. The theorem claims that the three straight lines , , and are concurrent. This result was established by the Romanian mathematician Cezar Coşniţă (1910-1962).
Their point of concurrence is known as the triangle's Kosnita point (named by Rigby in 1997). It is the isogonal conjugate of the nine-point center. It is triangle center in Clark Kimberling's list. This theorem is a special case of Dao's theorem on six circumcenters associated with a cyclic hexagon in.
References
- Weisstein, Eric W. "Kosnita Theorem". MathWorld.
- Ion Pătraşcu (2010), A generalization of Kosnita's theorem (in Romanian)
- Darij Grinberg (2003), On the Kosnita Point and the Reflection Triangle. Forum Geometricorum, volume 3, pages 105–111. ISSN 1534-1178
- John Rigby (1997), Brief notes on some forgotten geometrical theorems. Mathematics and Informatics Quarterly, volume 7, pages 156-158 (as cited by Kimberling).
- Clark Kimberling (2014), Encyclopedia of Triangle Centers Archived 2012-04-19 at the Wayback Machine, section X(54) = Kosnita Point. Accessed on 2014-10-08
- Nikolaos Dergiades (2014), Dao's Theorem on Six Circumcenters associated with a Cyclic Hexagon. Forum Geometricorum, volume 14, pages=243–246. ISSN 1534-1178.
- Telv Cohl (2014), A purely synthetic proof of Dao's theorem on six circumcenters associated with a cyclic hexagon. Forum Geometricorum, volume 14, pages 261–264. ISSN 1534-1178.
- Ngo Quang Duong, International Journal of Computer Discovered Mathematics, Some problems around the Dao's theorem on six circumcenters associated with a cyclic hexagon configuration, volume 1, pages=25-39. ISSN 2367-7775
- Clark Kimberling (2014), X(3649) = KS(INTOUCH TRIANGLE)
- Nguyễn Minh Hà, Another Purely Synthetic Proof of Dao's Theorem on Sixcircumcenters. Journal of Advanced Research on Classical and Modern Geometries, ISSN 2284-5569, volume 6, pages 37–44. MR....
- Nguyễn Tiến Dũng, A Simple proof of Dao's Theorem on Sixcircumcenters. Journal of Advanced Research on Classical and Modern Geometries, ISSN 2284-5569, volume 6, pages 58–61. MR....
- The extension from a circle to a conic having center: The creative method of new theorems, International Journal of Computer Discovered Mathematics, pp.21-32.
This geometry-related article is a stub. You can help Misplaced Pages by expanding it. |
 be an arbitrary triangle,
be an arbitrary triangle,  its
its  are the circumcenters of three triangles
are the circumcenters of three triangles  ,
,  , and
, and  respectively. The theorem claims that the three
respectively. The theorem claims that the three  ,
,  , and
, and  are concurrent. This result was established by the Romanian mathematician
are concurrent. This result was established by the Romanian mathematician  in
in