This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these messages)
|
| Small complex icosidodecahedron | |
|---|---|
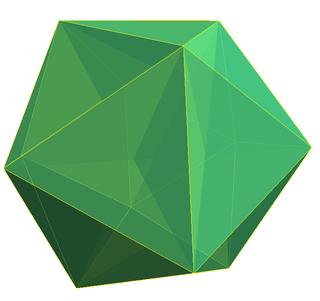
| |
| Type | Uniform star polyhedron |
| Elements | F = 32, E = 60 (30x2) V = 12 (χ = −16) |
| Faces by sides | 20{3}+12{5} |
| Coxeter diagram | |
| Wythoff symbol | 5 | 3/2 5 |
| Symmetry group | Ih, , *532 |
| Index references | U-, C-, W- |
| Dual polyhedron | Small complex icosidodecacron |
| Vertex figure | 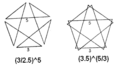 (3/2.5) (3.5)/3 |
| Bowers acronym | Cid |
In geometry, the small complex icosidodecahedron is a degenerate uniform star polyhedron. Its edges are doubled, making it degenerate. The star has 32 faces (20 triangles and 12 pentagons), 60 (doubled) edges and 12 vertices and 4 sharing faces. The faces in it are considered as two overlapping edges as topological polyhedron.
A small complex icosidodecahedron can be constructed from a number of different vertex figures.
A very similar figure emerges as a geometrical truncation of the great stellated dodecahedron, where the pentagram faces become doubly-wound pentagons ({5/2} --> {10/2}), making the internal pentagonal planes, and the three meeting at each vertex become triangles, making the external triangular planes.
As a compound
The small complex icosidodecahedron can be seen as a compound of the icosahedron {3,5} and the great dodecahedron {5,5/2} where all vertices are precise and edges coincide. The small complex icosidodecahedron resembles an icosahedron, because the great dodecahedron is completely contained inside the icosahedron.

|

|

|
| Icosahedron | Great dodecahedron | Compound |
Its two-dimensional analogue would be the compound of a regular pentagon, {5}, representing the icosahedron as the n-dimensional pentagonal polytope, and regular pentagram, {5/2}, as the n-dimensional star. These shapes would share vertices, similarly to how its 3D equivalent shares edges.

|

|
|
| Pentagon | Pentagram | Compound |
See also
References
- Coxeter, Harold Scott MacDonald; Longuet-Higgins, M. S.; Miller, J. C. P. (1954), "Uniform polyhedra", Philosophical Transactions of the Royal Society of London. Series A. Mathematical and Physical Sciences, 246 (916): 401–450, Bibcode:1954RSPTA.246..401C, doi:10.1098/rsta.1954.0003, ISSN 0080-4614, JSTOR 91532, MR 0062446, S2CID 202575183 (Table 6, degenerate cases)
- Weisstein, Eric W. "Small complex icosidodecahedron". MathWorld.
- Klitzing, Richard. "3D uniform polyhedra x3/2o5o5*a - cid".