Misplaced Pages

Stone functor

Article snapshot taken from Wikipedia with creative commons attribution-sharealike license. Give it a read and then ask your questions in the chat. We can research this topic together.

Functor in category theory

This article may be too technical for most readers to understand. Please help improve it to make it understandable to non-experts, without removing the technical details. (February 2024) (Learn how and when to remove this message)

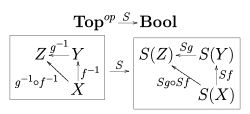
In mathematics, the Stone functor is a functor S: Top → Bool, where Top is the category of topological spaces and Bool is the category of Boolean algebras and Boolean homomorphisms. It assigns to each topological space X the Boolean algebra S(X) of its clopen subsets, and to each morphism f: X → Y in Top (i.e., a continuous map f: Y → X) the homomorphism S(f): S(X) → S(Y) given by S(f)(Z) = f.

See also

References

Abstract and Concrete Categories. The Joy of Cats Archived 2015-04-21 at the Wayback Machine. Jiri Adámek, Horst Herrlich, George E. Strecker.
Peter T. Johnstone, Stone Spaces. (1982) Cambridge university Press ISBN 0-521-23893-5

This category theory-related article is a stub. You can help Misplaced Pages by expanding it.

v
t
e

This topology-related article is a stub. You can help Misplaced Pages by expanding it.

v
t
e