| Revision as of 17:38, 10 November 2007 editGulliveig (talk | contribs)Extended confirmed users738 edits →History: Added Coulomb as a ref to a main article of SI units← Previous edit | Latest revision as of 20:40, 29 December 2024 edit undoGorillaWarfare (talk | contribs)Edit filter managers, Autopatrolled, Oversighters, Administrators119,128 editsm Reverted edits by 2A00:23C8:75C4:AC01:7924:3F5B:BDFF:8F2E (talk) (HG) (3.4.12)Tags: Huggle Rollback | ||
| Line 1: | Line 1: | ||
| {{short description|Modern form of the metric system}} | |||
| {{Redirect|SI|other uses|Si}} | |||
| {{Redirect|SI|the chemical element with symbol Si|Silicon|other uses|SI (disambiguation)}} | |||
| {{Use British English|date=September 2024}} | |||
| {{Use dmy dates|date=September 2024}} | |||
| The '''International System of Units''', internationally known by the abbreviation '''SI''' (from French {{lang|fr|Système international d'unités}}), is the modern form of the ] and the world's most widely used ]. It is the only system of measurement with official status in nearly every country in the world, employed in science, technology, industry, and everyday commerce. The SI system is coordinated by the ] which is abbreviated BIPM from {{langx|fr|Bureau international des poids et mesures}}. | |||
| ] (outer ring) and ] (inner ring) ]] | |||
| {| class="wikitable floatright" style="width: 400px; text-align:center;" | |||
| |+ The seven SI base units | |||
| ! scope="col" | Symbol | |||
| ! scope="col" | Name | |||
| ! scope="col" | Quantity | |||
| |- | |||
| ! scope="row" | s | |||
| | ] || ] <!-- one of seven --> | |||
| |- | |||
| ! scope="row" | m | |||
| | ] || ] <!-- two of seven --> | |||
| |- | |||
| ! scope="row" | kg | |||
| | ] || ] <!-- three of seven --> | |||
| |- | |||
| ! scope="row" | A | |||
| | ] || ] <!-- four of seven --> | |||
| |- | |||
| ! scope="row" | K | |||
| | ] || ] <!-- five of seven --> | |||
| |- | |||
| ! scope="row" | mol | |||
| | ] || ] <!-- six of seven --> | |||
| |- | |||
| ! scope="row" | cd | |||
| | ]|| ] <!-- seven of seven. That is all. --> | |||
| |} | |||
| The SI comprises a ] system of ] starting with seven ]s, which are the ] (symbol s, the unit of ]), ] (m, ]), ] (kg, ]), ] (A, ]), ] (K, ]), ] (mol, ]), and ] (cd, ]). The system can accommodate coherent units for an unlimited number of additional quantities. These are called coherent ], which can always be represented as products of powers of the base units. Twenty-two coherent derived units have been provided with special names and symbols. | |||
| The seven base units and the 22 coherent derived units with special names and symbols may be used in combination to express other coherent derived units. Since the sizes of coherent units will be convenient for only some applications and not for others, the SI provides twenty-four ]es which, when added to the name and symbol of a coherent unit produce twenty-four additional (non-coherent) SI units for the same quantity; these non-coherent units are always decimal (i.e. power-of-ten) multiples and sub-multiples of the coherent unit. | |||
| ''.]] | |||
| The current way of defining the SI is a result of a decades-long move towards increasingly abstract and idealised formulation in which the ] of the units are separated conceptually from the definitions. A consequence is that as science and technologies develop, new and superior realisations may be introduced without the need to redefine the unit. One problem with artefacts is that they can be lost, damaged, or changed; another is that they introduce uncertainties that cannot be reduced by advancements in science and technology. | |||
| The '''International System of Units''' (abbreviated '''SI''' from the ] ''{{lang|fr| Le '''S'''ystème '''i'''nternational d'unités}}'') is the modern form of the ]. It is the world's most widely used ], both in everyday ] and in ].<!--English units are still used in some scientific applications, but note also that parsecs in astronomy, calories and mmHg in the medical sciences, and electronvolts in physics are not part of the specific system of units known as SI, to just scratch the surface--> | |||
| The original motivation for the development of the SI was the diversity of units that had sprung up within the ] (CGS) systems (specifically the inconsistency between the systems of ] and ]) and the lack of coordination between the various ]s that used them. The General Conference on Weights and Measures (French: ''{{lang|fr|Conférence générale des poids et mesures}}'' – CGPM), which was established by the ] of 1875, brought together many international organisations to establish the definitions and standards of a new system and to standardise the rules for writing and presenting measurements. The system was published in 1960 as a result of an initiative that began in 1948, and is based on the ] (MKS) combined with ideas from the development of the CGS system. | |||
| The older metric system included several groups of units. The SI was developed in ] from the old ]-]-] (mks) system, rather than the ] (cgs) system, which, in turn, had a few variants. | |||
| == Definition == | |||
| The SI introduced several newly named units. The SI is not static — units are created and definitions are modified through international agreement among many nations as the technology of measurement progresses. | |||
| <!-- no wikilinks in this paragraph please --> | |||
| The system is nearly universally employed, and most countries do not even maintain official definitions of any other units. A notable exception is the ], which still uses many old units in addition to SI. In the ], ] is government policy, but the transition is not yet complete. Those countries that still recognise non-SI units (e.g. the US and UK) have redefined their traditional non-SI units ]. <!--In the U.S. at least, inch and pound have been defined in terms of metric units for a long time.--> | |||
| The International System of Units consists of a set of defining constants with corresponding base units, derived units, and a set of decimal-based multipliers that are used as prefixes.<ref name="SIBrochure9thEd"/>{{rp|page=125}} | |||
| === SI defining constants === | |||
| ==History== | |||
| {{main|Metre|Grave (mass)|Kilogram|Second|Ampere|Kelvin|Mole (unit)|Candela|Coulomb}} | |||
| {| class="wikitable floatright" style="width: 400px; text-align:center;" | |||
| The ] was conceived by a group of scientists (among them, ], who is known as the "father of modern chemistry") which had been commissioned by King ] of ] to create a unified and rational system of measures. After the ], the system was adopted by the new government.<ref>{{cite web|url=http://www1.bipm.org/en/si/history-si/name_kg.html|title=The name "kilogram"|accessdate=2006-07-25}}</ref> On ], ], the National Convention adopted the new decimal "metre" with a provisional length as well as the other decimal units with preliminary definitions and terms. On ], ] (''Loi du 18 germinal, an III'') the terms ''gramme'' and ''kilogramme'' replaced the former terms "gravet" (correctly "milligrave") and "]". On ], ] (a month after ]), the metric system was definitively adopted in ]. | |||
| |+ SI defining constants | |||
| ! scope="col" | Symbol | |||
| ! scope="col" | Defining constant | |||
| ! scope="col" | Exact value | |||
| |- | |||
| ! scope="row" | {{math|Δ''ν''<sub>Cs</sub>}} | |||
| | ] || {{val|9192631770|u=Hz}} | |||
| |- | |||
| ! scope="row" | {{mvar|c}} | |||
| | ] || {{val|299792458|u=m/s}} | |||
| |- | |||
| ! scope="row" | {{mvar|h}} | |||
| | ] || {{val|6.62607015|e=-34|u=J.s}} | |||
| |- | |||
| ! scope="row" | {{mvar|e}} | |||
| | ] || {{val|1.602176634|e=-19|u=C}} | |||
| |- | |||
| ! scope="row" | {{mvar|k}} | |||
| | ] || {{val|1.380649|e=-23|u=J/K}} | |||
| |- | |||
| ! scope="row" | {{Math|''N''<sub>A</sub>}} | |||
| | ] || {{val|6.02214076|e=23|u=mol-1}} | |||
| |- | |||
| ! scope="row" | {{Math|''K''<sub>cd</sub>}} | |||
| | ] of {{val|540|u=THz}} radiation || {{val|683|u=lm/W}} | |||
| |} | |||
| The seven defining constants are the most fundamental feature of the definition of the system of units.<ref name="SIBrochure9thEd"/>{{rp|page=125}} | |||
| The magnitudes of all SI units are defined by declaring that seven constants have certain exact numerical values when expressed in terms of their SI units. These defining constants are the ] in vacuum {{Math|''c''}}, the ] {{math|Δ''ν''{{sub|Cs}}}}, the ] {{Math|''h''}}, the ] {{Math|''e''}}, the ] {{Math|''k''}}, the ] {{Math|''N''<sub>A</sub>}}, and the ] {{Math|''K''<sub>cd</sub>}}. The nature of the defining constants ranges from fundamental constants of nature such as {{Math|''c''}} to the purely technical constant {{Math|''K''<sub>cd</sub>}}. The values assigned to these constants were fixed to ensure continuity with previous definitions of the base units.<ref name="SIBrochure9thEd"/>{{rp|page=128}} | |||
| === SI base units === | |||
| The ] has seen a number of variations, whose use has spread around the world, to replace many traditional ]. At the end of ] a number of different systems of measurement were still in use throughout the world. Some of these systems were metric-system variations, while others were based on the ] and ] systems. It was recognized that additional steps were needed to ]. As a result the 9th ] (CGPM), in 1948, asked the ] (CIPM) to conduct an international study of the measurement needs of the scientific, technical, and educational communities. | |||
| {{Main|SI base unit}} | |||
| Based on the findings of this study, the 10th CGPM in 1954 decided that an international system should be derived from six base units to provide for the measurement of temperature and optical radiation in addition to mechanical and electromagnetic quantities. The six base units recommended were the ], ], ], ], degree ] (later renamed the kelvin), and the ]. In 1960, the 11th CGPM named the system the ''International System of Units'', abbreviated SI from the French name: {{lang|fr|''Le Système international d'unités''}}. The seventh base unit, the ], was added in 1971 by the 14th CGPM. | |||
| The SI selects seven units to serve as ]s, corresponding to seven base physical quantities. They are the ], with the symbol {{val|u=s}}, which is the SI unit of the physical quantity of ]; the ], symbol {{val|u=m}}, the SI unit of ]; ] ({{val|u=kg}}, the unit of ]); ] ({{val|u=A}}, ]); ] ({{val|u=K}}, ]); ] ({{val|u=mol}}, ]); and ] ({{val|u=cd}}, ]).<ref name="SIBrochure9thEd" /> | |||
| === Future development === | |||
| The base units are defined in terms of the defining constants. For example, the kilogram is defined by taking the Planck constant {{math|''h''}} to be {{val|6.62607015|e=-34|u=J.s}}, giving the expression in terms of the defining constants<ref name="SIBrochure9thEd" />{{rp|page=131}} | |||
| : {{nowrap|1={{val|1|u=kg}} = {{sfrac|({{val|299792458}}){{sup|2}}|({{val|6.62607015|e=-34}})({{val|9192631770}})}}{{sfrac|{{math|''h''}}{{thin space}}{{math|Δ''ν''{{sub|Cs}}}}|{{math|''c''}}{{sup|2}}}}.}} | |||
| All units in the SI can be expressed in terms of the base units, and the base units serve as a preferred set for expressing or analysing the relationships between units. The choice of which and even how many quantities to use as base quantities is not fundamental or even unique – it is a matter of convention.<ref name="SIBrochure9thEd" />{{rp|page=126|quote= the choice of the base units was never unique, but grew historically and became familiar to users of the SI}} | |||
| {| class="wikitable" style="margin:1em auto 1em auto" | |||
| The ] standard ] contains recommendations for the use of the International System of Units; for applications in electrical applications additionally the ] standard IEC 60027 has to be taken into account. As of 2008 work is proceeding {{Fact|date=August 2007}} to integrate both standards into a joint standard ] to be referred as '''International System of Quantities''' (ISQ). | |||
| |+ <big>SI base units</big><ref name="SIBrochure9thEd"/>{{rp|page=136}} | |||
| |- | |||
| ! scope="col" | Unit name | |||
| ! scope="col" | Unit symbol | |||
| ! scope="col" | ] | |||
| ! scope="col" | ] | |||
| ! scope="col" width=80 |Typical symbols | |||
| ! scope="col" | Definition | |||
| |- | |||
| ! scope="row" | ] | |||
| |style="text-align:center" |s | |||
| |style="text-align:center" |<math>\mathsf{T}</math> | |||
| | ] | |||
| |<math>t</math> | |||
| |The duration of {{val|9192631770}} periods of the radiation corresponding to the transition between the two ] levels of the ] of the ] atom. | |||
| |- | |||
| ! scope="row" | ] | |||
| |style="text-align:center" |m | |||
| |style="text-align:center" |<math>\mathsf{L}</math> | |||
| |] | |||
| |<math>l</math>, <math>x</math>, <math>r</math>, etc. | |||
| |The distance travelled by light in vacuum in {{sfrac|{{val|299792458}}}} second. | |||
| |- | |||
| ! scope="row" | ]{{br}}<ref group="n">Despite the prefix "kilo-", the kilogram is the coherent base unit of mass, and is used in the definitions of derived units. Nonetheless, prefixes for the unit of mass are determined as if the gram were the base unit.</ref> | |||
| |style="text-align:center" |kg | |||
| |style="text-align:center" |<math>\mathsf{M}</math> | |||
| |] | |||
| |<math>m</math> | |||
| |The kilogram is defined by setting the ] {{math|''h''}} to {{val|6.62607015|e=-34|u=J.s}} ({{nowrap|1=J = kg⋅m{{sup|2}}⋅s{{sup|−2}}}}), given the definitions of the metre and the second.<ref name="NIST 2018-11">{{cite news|url=https://www.nist.gov/news-events/news/2018/11/historic-vote-ties-kilogram-and-other-units-natural-constants|title=Historic Vote Ties Kilogram and Other Units to Natural Constants|last=Materese|first=Robin|date=16 November 2018|work=NIST|access-date=16 November 2018}}</ref> | |||
| |- | |||
| ! scope="row" | ] | |||
| |style="text-align:center" |A | |||
| |style="text-align:center" |<math>\mathsf{I}</math> | |||
| |] | |||
| |<math>I,\; i</math> | |||
| |The flow of {{sfrac|1|{{val|1.602176634|e=-19}}}} times the ] {{math|''e''}} per second, which is approximately {{val|6.2415090744|e=18}} elementary charges per second. | |||
| |- | |||
| ! scope="row" | ] | |||
| |style="text-align:center" |K | |||
| |style="text-align:center" |<math>\mathsf{\Theta}</math> | |||
| |] | |||
| |<math>T</math> | |||
| |The kelvin is defined by setting the fixed numerical value of the ] {{math|''k''}} to {{val|1.380649|e=-23|u=J.K-1}}, ({{nowrap|1=J = kg⋅m<sup>2</sup>⋅s<sup>−2</sup>}}), given the definition of the kilogram, the metre, and the second. | |||
| |- | |||
| ! scope="row" | ] | |||
| |style="text-align:center" |mol | |||
| |style="text-align:center" |<math>\mathsf{N}</math> | |||
| |] | |||
| |<math>n</math> | |||
| |The amount of substance of {{val|6.02214076|e=23}} elementary entities.<ref group="n">When the mole is used, the elementary entities must be specified and may be ]s, ]s, ]s, ]s, other particles, or specified groups of such particles.</ref> This number is the fixed numerical value of the ], {{math|''N''<sub>A</sub>}}, when expressed in the unit mol<sup>−1</sup>. | |||
| |- | |||
| ! scope="row" | ] | |||
| |style="text-align:center" |cd | |||
| |style="text-align:center" |<math>\mathsf{J}</math> | |||
| |] | |||
| |<math>I_{\rm v}</math> | |||
| |The luminous intensity, in a given direction, of a source that emits monochromatic radiation of frequency {{val|5.4|e=14|u=hertz}} and that has a radiant intensity in that direction of {{sfrac|1|683}} watt per ]. | |||
| |- | |||
| | colspan="6" | | |||
| ; Notes | |||
| {{reflist|group="n"}} | |||
| |} | |||
| == |
=== Derived units === | ||
| {{main|SI base unit|SI derived unit|SI prefix}} | |||
| {{Main|SI derived unit}} | |||
| The international system of units consists of a set of units together with a set of ]es. The units of SI can be divided into two subsets. There are seven ]. Each of these base units are nominally dimensionally independent. From these seven base units several ] are derived. In addition to the SI units there are also a set of ]. | |||
| The system allows for an unlimited number of additional units, called '']'', which can always be represented as products of powers of the base units, possibly with a nontrivial numeric multiplier. When that multiplier is one, the unit is called a '']'' derived unit. For example, the coherent derived SI unit of ] is the ], with the symbol {{val|u=m/s}}.<ref name=SIBrochure9thEd/>{{rp|page=139}} The base and coherent derived units of the SI together form a coherent system of units (''the set of coherent SI units''). A useful property of a coherent system is that when the numerical values of physical quantities are expressed in terms of the units of the system, then the equations between the numerical values have exactly the same form, including numerical factors, as the corresponding equations between the physical quantities.<ref name="ISO80000-1">{{cite ISO standard|csnumber=30669|title=ISO 80000-1:2009 Quantities and units – Part 1: General}}</ref>{{rp|page=6}} | |||
| Twenty-two coherent derived units have been provided with special names and symbols as shown in the table below. The radian and steradian have no base units but are treated as derived units for historical reasons.<ref name=SIBrochure9thEd/>{{rp|page=137}} | |||
| {| class="wikitable" style="margin:1em auto 1em auto" | |||
| |+'''SI base units'''<ref name=sp330>{{cite book |author=Barry N. Taylor, Ed. |title= The International System of Units (SI)|origyear= 2001 |url=http://physics.nist.gov/Pubs/SP330/sp330.pdf |accessdate=2007-10-30|publisher=National Institute of Standards and Technology|location= Gaithersburg, MD|pages=9}} </ref> | |||
| {| class="wikitable floatleft" style="margin:1em auto 1em auto;line-height:1.4" | |||
| |+ <big>The 22 SI derived units with special names and symbols</big><ref name="NIST330" />{{rp|page=15}} | |||
| |- | |- | ||
| !Name | ! scope="col" | Name | ||
| !Symbol | ! scope="col" | Symbol | ||
| !Quantity | ! scope="col" | Quantity | ||
| ! scope="col" | In SI base units | |||
| ! scope="col" | In other SI units | |||
| |- | |- | ||
| ! scope="row" | ]<ref name=":0" group="N">The radian and steradian are defined as dimensionless derived units.</ref> | |||
| !] | |||
| | style="text-align:center;" | rad | |||
| |'''m''' | |||
| |] | | ] | ||
| | style="text-align:center;" | m/m | |||
| | style="text-align:center;" | 1 | |||
| |- | |- | ||
| ! scope="row" | ]<ref name=":0" group="N" /> | |||
| !] | |||
| | style="text-align:center;" | sr | |||
| |'''kg''' | |||
| |] | | ] | ||
| | style="text-align:center;" | m<sup>2</sup>/m<sup>2</sup> | |||
| | style="text-align:center;" | 1 | |||
| |- | |- | ||
| ! scope="row" | ] | |||
| !] | |||
| | style="text-align:center;" | Hz | |||
| |'''s''' | |||
| |] | | ] | ||
| | style="text-align:center;" | s<sup>−1</sup> | |||
| | <!-- intentionally left blank --> | |||
| |- | |- | ||
| ! scope="row" | ] | |||
| !] | |||
| | style="text-align:center;" | N | |||
| |'''A''' | |||
| | ] | |||
| |] | |||
| | style="text-align:center;" | kg⋅m⋅s<sup>−2</sup> | |||
| | <!-- intentionally left blank --> | |||
| |- | |- | ||
| ! scope="row" | ] | |||
| !] | |||
| | style="text-align:center;" | Pa | |||
| |'''K''' | |||
| | ], ] | |||
| |] | |||
| | style="text-align:center;" | kg⋅m<sup>−1</sup>⋅s<sup>−2</sup> | |||
| | style="text-align:center;" | N/m<sup>2</sup> = J/m<sup>3</sup> | |||
| |- | |- | ||
| ! scope="row" | ] | |||
| !] | |||
| | style="text-align:center;" | J | |||
| |'''mol''' | |||
| |] | | ], ], amount of ] | ||
| | style="text-align:center;" | kg⋅m<sup>2</sup>⋅s<sup>−2</sup> | |||
| | style="text-align:center;" | N⋅m = Pa⋅m<sup>3</sup> | |||
| |- | |- | ||
| ! scope="row" | ] | |||
| !] | |||
| | style="text-align:center;" | W | |||
| |'''cd''' | |||
| | ], ] | |||
| |] | |||
| | style="text-align:center;" | kg⋅m<sup>2</sup>⋅s<sup>−3</sup> | |||
| | style="text-align:center;" | J/s | |||
| |- | |- | ||
| ! scope="row" | ] | |||
| | style="text-align:center;" | C | |||
| | ] | |||
| | style="text-align:center;" | s⋅A | |||
| | <!-- intentionally left blank --> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | V | |||
| | ], (] in US; electric tension) | |||
| | style="text-align:center;" | kg⋅m<sup>2</sup>⋅s<sup>−3</sup>⋅A<sup>−1</sup> | |||
| | style="text-align:center;" | W/A = J/C | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | F | |||
| | ] | |||
| | style="text-align:center;" | kg<sup>−1</sup>⋅m<sup>−2</sup>⋅s<sup>4</sup>⋅A<sup>2</sup> | |||
| | style="text-align:center;" | C/V = C<sup>2</sup>/J | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | Ω | |||
| | ] | |||
| | style="text-align:center;" | kg⋅m<sup>2</sup>⋅s<sup>−3</sup>⋅A<sup>−2</sup> | |||
| | style="text-align:center;" | V/A = J⋅s/C<sup>2</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | S | |||
| | ] | |||
| | style="text-align:center;" | kg<sup>−1</sup>⋅m<sup>−2</sup>⋅s<sup>3</sup>⋅A<sup>2</sup> | |||
| | style="text-align:center;" | Ω<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | Wb | |||
| | ] | |||
| | style="text-align:center;" | kg⋅m<sup>2</sup>⋅s<sup>−2</sup>⋅A<sup>−1</sup> | |||
| | style="text-align:center;" | V⋅s | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | T | |||
| | ] | |||
| | style="text-align:center;" | kg⋅s<sup>−2</sup>⋅A<sup>−1</sup> | |||
| | style="text-align:center;" | Wb/m<sup>2</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | H | |||
| | ] | |||
| | style="text-align:center;" | kg⋅m<sup>2</sup>⋅s<sup>−2</sup>⋅A<sup>−2</sup> | |||
| | style="text-align:center;" | Wb/A | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | °C | |||
| | ] | |||
| | style="text-align:center;" | K | |||
| | <!-- intentionally left blank --> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | lm | |||
| | ] | |||
| | style="text-align:center;" | cd⋅m<sup>2</sup>/m<sup>2</sup> | |||
| | style="text-align:center;" | cd⋅sr | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | lx | |||
| | ] | |||
| | style="text-align:center;" | cd⋅m<sup>2</sup>/m<sup>4</sup> | |||
| | style="text-align:center;" | lm/m<sup>2</sup> = cd⋅sr⋅m<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | Bq | |||
| | ] (decays per unit time) | |||
| | style="text-align:center;" | s<sup>−1</sup> | |||
| | <!-- intentionally left blank --> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | Gy | |||
| | ], ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅s<sup>−2</sup> | |||
| | style="text-align:center;" | J/kg | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | Sv | |||
| | ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅s<sup>−2</sup> | |||
| | style="text-align:center;" | J/kg | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | kat <!-- Note: this is the last row, there are exactly 22 in the ref --> | |||
| | ] | |||
| | style="text-align:center;" | mol⋅s<sup>−1</sup> | |||
| | <!-- intentionally left blank --> | |||
| |- | |||
| <!-- Note: there are exactly 22 in the ref --> | |||
| | colspan="5" | '''Notes''' | |||
| <references group="N" /> | |||
| |} | |} | ||
| {{Clear}} | |||
| The derived units in the SI are formed by powers, products, or quotients of the base units and are unlimited in number.<ref name="SIBrochure">{{SIbrochure8th}}</ref>{{rp|page=103}}<ref name="NIST330"/>{{rp|pages=14,16}} | |||
| A ] may be added to units to produce a multiple of the original unit. All multiples are integer powers of ten. For example, ''kilo-'' denotes a multiple of a thousand and ''milli-'' denotes a multiple of a thousandth hence there are one thousand millimetres to the metre and one thousand metres to the kilometre. The prefixes are never combined: a millionth of a kilogram is a ''milligram'' not a ''microkilogram''. | |||
| ] | |||
| <center>{{SI-Prefixes}}</center> | |||
| ]s apply to some ], which may by definition be expressed in terms of ], and thus are not independent; for example, ] is the inverse of ], with the consequence that the siemens is the inverse of the ohm, and similarly, the ohm and siemens can be replaced with a ratio of an ampere and a volt, because those quantities bear a defined relationship to each other.{{efn|Ohm's law: {{nowrap|1=1 Ω = 1 V/A}} from the relationship {{nowrap|1=''E'' = ''I'' × ''R''}}, where ''E'' is electromotive force or voltage (unit: volt), ''I'' is current (unit: ampere), and ''R'' is resistance (unit: ohm).}} Other useful derived quantities can be specified in terms of the SI base and derived units that have no named units in the SI, such as acceleration, which has the SI unit m/s<sup>2</sup>.<ref name="SIBrochure9thEd" />{{rp|page=139}} | |||
| == SI writing style == | |||
| A combination of base and derived units may be used to express a derived unit. For example, the SI unit of ] is the ] (N), the SI unit of ] is the ] (Pa) – and the pascal can be defined as one newton per square metre (N/m<sup>2</sup>).<ref>{{cite web |title=Units & Symbols for Electrical & Electronic Engineers |url=http://www.theiet.org/students/resources/units-symbols.cfm |publisher=Institution of Engineering and Technology |date=1996 |pages=8–11 |access-date=19 August 2013 |archive-url=https://web.archive.org/web/20130628212624/http://www.theiet.org/students/resources/units-symbols.cfm |archive-date=28 June 2013}}</ref> | |||
| * Symbols do not have an appended period/full stop (.) unless at the end of a sentence. | |||
| === Prefixes === | |||
| * Symbols are written in upright ] (m for metres, L for litres), so as to differentiate from the ] used for mathematical variables (''m'' for mass, ''l'' for length). | |||
| {{Main|Metric prefix}} | |||
| * Symbols for units are written in ], except for symbols derived from the name of a person. For example, the unit of ] is named after ], so its symbol is written "Pa" whereas the ] itself is written "]". | |||
| ** The one exception is the ], whose original symbol "l" is unsuitably similar to the numeral "1" or the uppercase letter "i" (depending on the typeface used), at least in many ]. The American ] recommends that "L" be used instead, a usage which is common in the US, Canada, Australia, and ] (but not elsewhere). This has been accepted as an alternative by the ] since ]. The cursive <math>\ell</math> is occasionally seen, especially in ] and ], but this is not currently recommended by any ].<!--there are lots of standards organisations and I'm pretty sure at least some of them did recommend this in the past; I think South Africa was also big on this--> For more information, see ]. | |||
| Like all metric systems, the SI uses ]es to systematically construct, for the same physical quantity, a set of units that are decimal multiples of each other over a wide range. For example, driving distances are normally given in ]s (symbol {{val|u=km}}) rather than in metres. Here the metric prefix ']' (symbol 'k') stands for a factor of 1000; thus, {{val|1|u=km}} = {{val|1000|u=m}}. | |||
| * The SI rule for pluralising units is that symbols of units are not pluralised<ref>{{cite paper|author= Bureau International des Poids et Mesures|date=2006|url=http://www.bipm.org/utils/common/pdf/si_brochure_8_en.pdf|title=The International System of Units (SI)|version= 8th ed.|accessdate=2006-07-14}}</ref>, for example "25 kg" (not "25 kgs"). | |||
| ** The American ] has defined guidelines for using the SI units in its own publications and for other users of the SI<ref name=Taylor>{{cite paper|author=Taylor, B.N.|date=1995|url=http://physics.nist.gov/Pubs/SP811/contents.html|title=NIST Special Publication 811: Guide for the Use of the International System of Units (SI)|publisher=National Institute of Standards and Technology|accessdate=2006-06-09}}</ref>. These guidelines give guidance on pluralizing unit names: the plural is formed by using normal ] rules, for example, "henries" is the plural of "]". The units ], ], and ] are exceptions from this rule: they remain the same in singular and plural. Note that this rule only applies to the full names of units, not to their symbols. | |||
| The SI provides twenty-four metric prefixes that signify decimal powers ranging from 10<sup>−30</sup> to 10<sup>30</sup>, the most recent being adopted in 2022.<ref name="SIBrochure9thEd" />{{rp|pages=143–144}}<ref>{{cite web |first1=Daniel|last1=Lawler|title=Earth now weighs six ronnagrams: New metric prefixes voted in |url=https://phys.org/news/2022-11-earth-ronnagrams-metric-prefixes-voted.html |publisher=phys.org |date=18 November 2022}}</ref><ref>{{cite web |date=18 November 2022 |title=List of Resolutions for the 27th meeting of the General Conference on Weights and Measures |url=https://www.bipm.org/documents/20126/64811223/Resolutions-2022.pdf |publisher=BIPM|url-status=dead |access-date=18 November 2022 |archive-date=18 November 2022 |archive-url=https://web.archive.org/web/20221118153958/https://www.bipm.org/documents/20126/64811223/Resolutions-2022.pdf}}</ref><ref>{{cite web |title=New prefixes for the SI adopted by the General Conference on Weights and Measures |url=https://www.bipm.org/en/-/2022-12-19-si-prefixes |access-date=11 January 2023 |website=BIPM }}</ref> Most prefixes correspond to integer powers of 1000; the only ones that do not are those for 10, 1/10, 100, and 1/100. | |||
| * A space separates the number and the symbol, e.g. "2.21 kg", "7.3{{e|2}} m²", "22 K" <ref name='nist style'> {{cite web|url=http://physics.nist.gov/Pubs/SP811/sec07.html |title=NIST Guide to SI Units - Rules and Style Conventions |accessdate=2007-04-12 |last=Taylor |first=B. N. |publisher=National Institute of Standards and Technology }}</ref>. Exceptions are the symbols for plane angular degrees, minutes and seconds (°, ′ and ″), which are placed immediately after the number with no intervening space. | |||
| The conversion between different SI units for one and the same physical quantity is always through a power of ten. This is why the SI (and metric systems more generally) are called ''decimal systems of measurement units''.<ref name="DecimalSystem">{{cite web |url=https://usma.org/decimal-nature-of-the-metric-system |title=Decimal Nature of the Metric System |publisher=] | date=2015 |access-date=15 April 2020 |archive-url=https://web.archive.org/web/20200415225727/https://usma.org/decimal-nature-of-the-metric-system/ |archive-date=15 April 2020 |url-status=live}}</ref> | |||
| {{Anchor|Compound unit}}The grouping formed by a prefix symbol attached to a unit symbol (e.g. '{{val|u=km}}', '{{val|u=cm}}') constitutes a new inseparable unit symbol. This new symbol can be raised to a positive or negative power. It can also be combined with other unit symbols to form ''compound unit'' symbols.<ref name="SIBrochure9thEd" />{{rp|page=143}} For example, {{val|u=g/cm3}} is an SI unit of ], where {{val|u=cm3}} is to be interpreted as ({{val|u=cm}}){{sup|3}}. | |||
| * Spaces may be used as a ] (''1<font size="-1"> </font>000<font size="-1"> </font>000'') in contrast to commas or periods (''1,000,000'' or ''1.000.000'') in order to reduce confusion resulting from the variation between these forms in different countries. ], the space used for this purpose is typically narrower than that between words. | |||
| Prefixes are added to unit names to produce multiples and ]s of the original unit. All of these are integer powers of ten, and above a hundred or below a hundredth all are integer powers of a thousand. For example, ''kilo-'' denotes a multiple of a thousand and ''milli-'' denotes a multiple of a thousandth, so there are one thousand millimetres to the metre and one thousand metres to the kilometre. The prefixes are never combined, so for example a millionth of a metre is a ''micrometre'', not a ''millimillimetre''. Multiples of the kilogram are named as if the gram were the base unit, so a millionth of a kilogram is a ''milligram'', not a ''microkilogram''.<ref name="SIBrochure"/>{{rp|page=122}}<ref name="NIST811"/>{{rp|page=14}} | |||
| * The 10th resolution of ] in 2003 declared that "the symbol for the ] shall be either the ] on the line or the ] on the line". In practice, the decimal point is used in English, and the comma in most other ]. | |||
| The BIPM specifies 24 prefixes for the International System of Units (SI): | |||
| * Symbols for derived units formed from multiple units by multiplication are joined with a space or ] (·), e.g. "N m" or "N·m". <ref>{{cite book |author=Barry N. Taylor, Ed.| |title= The International System of Units (SI)|origyear= 2001 |url=http://physics.nist.gov/Pubs/SP330/sp330.pdf |accessdate=2007-10-15|publisher= National Institute of Standards and Technology|location= Washington, DC|pages=30}} </ref> | |||
| {{SI prefixes (infobox)}} | |||
| * Symbols formed by division of two units are joined with a ] (⁄), or given as a negative ]. For example, the "metre per second" can be written "m/s", "m s<sup>−1</sup>", "m·s<sup>−1</sup>" or <math>\textstyle\frac{\mathrm{m}}{\mathrm{s}}.</math> A solidus should not be used if the result is ambiguous, i.e. "kg·m<sup>−1</sup>·s<sup>−2</sup>" is preferable to "kg/m·s²". Many computer users will type the / character provided on American ]s, which in turn produces the Unicode character <tt>U+002F</tt>, which is named solidus but is distinct from the Unicode solidus character, <tt>U+2044</tt>. | |||
| === Coherent and non-coherent SI units <span class="anchor" id="Coherent SI units"></span> === | |||
| * In ], ], and ] computing (]), some of the commonly used units, prefix-unit combinations, or unit-exponent combinations have been allocated predefined single characters taking up a full square. ] includes these in its and subranges for back compatibility, without necessarily recommending future usage. | |||
| {{Further|Coherent unit}} | |||
| * When writing dimensionless quantities, the terms 'ppb' (parts per ]) and 'ppt' (parts per ]) are recognised as language-dependent terms since the value of billion and trillion can ]. SI therefore recommends avoiding these terms . However, no alternative is suggested by BIPM. | |||
| The base units and the derived units formed as the product of powers of the base units with a numerical factor of one form a ]. Every physical quantity has exactly one coherent SI unit. For example, {{nowrap|1=1 m/s = 1 m / (1 s)}} is the coherent derived unit for velocity.<ref name="SIBrochure9thEd" />{{rp|page=139}} With the exception of the kilogram (for which the prefix kilo- is required for a coherent unit), when prefixes are used with the coherent SI units, the resulting units are no longer coherent, because the prefix introduces a numerical factor other than one.<ref name="SIBrochure9thEd" />{{rp|page=137}} For example, the metre, kilometre, centimetre, nanometre, etc. are all SI units of length, though only the metre is a {{em|coherent}} SI unit. The complete set of SI units consists of both the coherent set and the multiples and sub-multiples of coherent units formed by using the SI prefixes.<ref name="SIBrochure9thEd" />{{rp|page=138}} | |||
| === Spelling variations === | |||
| The kilogram is the only coherent SI unit whose name and symbol include a prefix. For historical reasons, the names and symbols for multiples and sub-multiples of the unit of mass are formed as if the ] were the base unit. Prefix names and symbols are attached to the unit name ''gram'' and the unit symbol g respectively. For example, {{val|e=-6|u=kg}} is written ''milligram'' and {{val|u=mg}}, not ''microkilogram'' and {{val|u=μkg}}.<ref name="SIBrochure9thEd" />{{rp|page=144}} | |||
| * Several nations typically use the spellings ''meter'' and ''liter'' instead of ''metre'' and ''litre'' in keeping with standard ] spelling, which also corresponds to the official spelling used in several other languages, such as German, Dutch, Swedish, etc. In addition, the official US spelling for the ] "deca" is ''deka''.<ref name='deka'>{{cite web|url=http://physics.nist.gov/cuu/Units/prefixes.html |title=Definitions of the SI units: The twenty SI prefixes |accessdate=2007-04-12 }}</ref> | |||
| Several different quantities may share the same coherent SI unit. For example, the joule per kelvin (symbol {{val|u=J/K}}) is the coherent SI unit for two distinct quantities: ] and ]; another example is the ampere, which is the coherent SI unit for both ] and ]. This illustrates why it is important not to use the unit alone to specify the quantity. As the ''SI Brochure'' states,<ref name="SIBrochure9thEd" />{{rp|page=140}} "this applies not only to technical texts, but also, for example, to measuring instruments (i.e. the instrument read-out needs to indicate both the unit and the quantity measured)". | |||
| * In some English-speaking countries, the unit "ampere" is often shortened to ''amp'' (singular) or ''amps'' (plural). | |||
| Furthermore, the same coherent SI unit may be a base unit in one context, but a coherent derived unit in another. For example, the ampere is a base unit when it is a unit of electric current, but a coherent derived unit when it is a unit of magnetomotive force.<ref name="SIBrochure9thEd" />{{rp|page=140}} | |||
| == Conversion factors == | |||
| {| class="wikitable floatleft" style="margin:1em auto 1em auto;line-height:1.4" | |||
| The relationship between the units used in different systems is determined by convention or from the basic definition of the units. Conversion of units from one system to another is accomplished by use of a ]. There are several compilations of conversion factors; see, for example Appendix B of NIST SP 811.<ref name=Taylor/> | |||
| |+ <big>Examples of coherent derived units in terms of base units</big><ref name="NIST330" />{{rp|page=17}} | |||
| |- | |||
| ! scope="col" | Name | |||
| ! scope="col" | Symbol | |||
| ! scope="col" | Derived quantity | |||
| ! scope="col" | Typical symbol | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=m2}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''A''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=m3}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''V''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=m/s}} | |||
| | ], ] | |||
| | style="text-align:center;" | {{math|''v''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=m/s2}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''a''}} | |||
| |- | |||
| ! scope="row" rowspan="2" | ] | |||
| | rowspan="2" style="text-align:center;" | {{val|u=m-1}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''σ''}}, {{math|''ṽ''}} | |||
| |- | |||
| | ] | |||
| | style="text-align:center;" | {{math|''V''}}, 1/{{math|''f''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=kg/m3}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''ρ''}} | |||
| |- | |||
| ! scope="row" | kilogram per square metre | |||
| | style="text-align:center;" | {{val|u=kg/m2}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''ρ''{{smallsub|A}}}} | |||
| |- | |||
| ! scope="row" | cubic metre per kilogram | |||
| | style="text-align:center;" | {{val|u=m3/kg}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''v''}} | |||
| |- | |||
| ! scope="row" | ampere per square metre | |||
| | style="text-align:center;" | {{val|u=A/m<sup>2</sup>}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''j''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=A/m}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''H''}} | |||
| |- | |||
| ! scope="row" | mole per cubic metre | |||
| | style="text-align:center;" | {{val|u=mol/m3}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''c''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=kg/m3}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''ρ''}}, {{math|''γ''}} | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | {{val|u=cd/m<sup>2</sup>}} | |||
| | ] | |||
| | style="text-align:center;" | {{math|''L''<sub>v</sub>}} | |||
| |} | |||
| {| class="wikitable floatleft" style="margin:1em auto 1em auto;line-height:1.4" | |||
| ==Cultural issues== | |||
| |+ <big>Examples of derived units that include units with special names</big><ref name="NIST330" />{{rp|page=18}} | |||
| The worldwide adoption of the metric system as a tool of economy and everyday commerce was based to some extent on the lack of customary systems in many countries to adequately describe some concepts, or as a result of an attempt to standardise the many regional variations in the customary system. International factors also affected the adoption of the metric system, as many countries increased their trade. Scientifically, it simplifies dealing with very large and small quantities since it lines up so well with the ] ]. | |||
| |- | |||
| ! scope="col" | Name | |||
| ! scope="col" | Symbol | |||
| ! scope="col" | Quantity | |||
| ! scope="col" | In SI base units | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | Pa⋅s | |||
| | ] | |||
| | style="text-align:center;" | m<sup>−1</sup>⋅kg⋅s<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | N⋅m | |||
| | ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅kg⋅s<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | newton per metre | |||
| | style="text-align:center;" | N/m | |||
| | ] | |||
| | style="text-align:center;" | kg⋅s<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | rad/s | |||
| | ], ] | |||
| | style="text-align:center;" | s<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | rad/s<sup>2</sup> | |||
| | ] | |||
| | style="text-align:center;" | s<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | W/m<sup>2</sup> | |||
| | heat flux density, ] | |||
| | style="text-align:center;" | kg⋅s<sup>−3</sup> | |||
| |- | |||
| ! scope="row" | ] | |||
| | style="text-align:center;" | J/K | |||
| | ], ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅kg⋅s<sup>−2</sup>⋅K<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | joule per kilogram-kelvin | |||
| | style="text-align:center;" | J/(kg⋅K) | |||
| | ], ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅s<sup>−2</sup>⋅K<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | joule per kilogram | |||
| | style="text-align:center;" | J/kg | |||
| | ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅s<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | watt per metre-kelvin | |||
| | style="text-align:center;" | W/(m⋅K) | |||
| | ] | |||
| | style="text-align:center;" | m⋅kg⋅s<sup>−3</sup>⋅K<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | joule per cubic metre | |||
| | style="text-align:center;" | J/m<sup>3</sup> | |||
| | ] | |||
| | style="text-align:center;" | m<sup>−1</sup>⋅kg⋅s<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | volt per metre | |||
| | style="text-align:center;" | V/m | |||
| | ] | |||
| | style="text-align:center;" | m⋅kg⋅s<sup>−3</sup>⋅A<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | coulomb per cubic metre | |||
| | style="text-align:center;" | C/m<sup>3</sup> | |||
| | ] | |||
| | style="text-align:center;" | m<sup>−3</sup>⋅s⋅A | |||
| |- | |||
| ! scope="row" | coulomb per square metre | |||
| | style="text-align:center;" | C/m<sup>2</sup> | |||
| | ], ], ] | |||
| | style="text-align:center;" | m<sup>−2</sup>⋅s⋅A | |||
| |- | |||
| ! scope="row" | farad per metre | |||
| | style="text-align:center;" | F/m | |||
| | ] | |||
| | style="text-align:center;" | m<sup>−3</sup>⋅kg<sup>−1</sup>⋅s<sup>4</sup>⋅A<sup>2</sup> | |||
| |- | |||
| ! scope="row" | henry per metre | |||
| | style="text-align:center;" | H/m | |||
| | ] | |||
| | style="text-align:center;" | m⋅kg⋅s<sup>−2</sup>⋅A<sup>−2</sup> | |||
| |- | |||
| ! scope="row" | joule per mole | |||
| | style="text-align:center;" | J/mol | |||
| | ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅kg⋅s<sup>−2</sup>⋅mol<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | joule per mole-kelvin | |||
| | style="text-align:center;" | J/(mol⋅K) | |||
| | ], ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅kg⋅s<sup>−2</sup>⋅K<sup>−1</sup>⋅mol<sup>−1</sup> | |||
| |- | |||
| ! scope="row" | coulomb per kilogram | |||
| | style="text-align:center;" | C/kg | |||
| | ] (x- and γ-rays) | |||
| | style="text-align:center;" | kg<sup>−1</sup>⋅s⋅A | |||
| |- | |||
| ! scope="row" | gray per second | |||
| | style="text-align:center;" | Gy/s | |||
| | ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅s<sup>−3</sup> | |||
| |- | |||
| ! scope="row" | watt per steradian | |||
| | style="text-align:center;" | W/sr | |||
| | ] | |||
| | style="text-align:center;" | m<sup>2</sup>⋅kg⋅s<sup>−3</sup> | |||
| |- | |||
| ! scope="row" | watt per square metre-steradian | |||
| | style="text-align:center;" | W/(m<sup>2</sup>⋅sr) | |||
| | ] | |||
| | style="text-align:center;" | kg⋅s<sup>−3</sup> | |||
| |- | |||
| ! scope="row" | katal per cubic metre | |||
| | style="text-align:center;" | kat/m<sup>3</sup> | |||
| | ] | |||
| | style="text-align:center;" | m<sup>−3</sup>⋅s<sup>−1</sup>⋅mol | |||
| |} | |||
| {{Clear}} | |||
| == Lexicographic conventions <span class="anchor" id="SI_writing_style"></span> == | |||
| Many units in everyday and scientific use are not derived from the seven SI base units (metre, kilogram, second, ampere, kelvin, mole, and candela) combined with the SI prefixes. In some cases these deviations have been approved by the BIPM. <ref>http://www.bipm.org/en/si/si_brochure/chapter4/table8.html</ref> Some examples include: | |||
| {{See also|ISO 31-0#Typographic conventions|Space (punctuation)#Unit symbols and numbers}} | |||
| * The many units of time — minute (min), hour (h), day (d) <!--- the only three in table 6 --->— in use besides the SI second, and are specifically accepted for use according to table 6. <ref>http://www.bipm.org/en/si/si_brochure/chapter4/table6.html</ref> | |||
| ] '2'.]] | |||
| * The year is specifically not included but has a recommended conversion factor. <ref>http://physics.nist.gov/Pubs/SP811/appenB9.html#TIME</ref> | |||
| * The ] temperature scale; kelvins are rarely employed in everyday use. | |||
| * ] is often billed in ] instead of megajoules. | |||
| * The ] and ] (nautical mile per hour) used to measure travel distance and speed of ships and aircraft (1 International nautical mile = 1852 m or approximately 1 minute of latitude at the equator). In addition to these, Annex 5 of the ] permits the "temporary use" of the ] for ]. | |||
| * ]s measured in ]s, ]s, and ]s instead of, say, petametres (a light-year is about 9.461 Pm or about 9 461 000 000 000 000 m). | |||
| * Atomic scale units used in physics and chemistry, such as the ], ], ], and ]. | |||
| * Some ]s still use the ] (CGS) units, with their associated non-SI electric units. | |||
| * In some countries, the informal ] measurement has become 250 ml. Likewise, a 500 g "]" is used in many countries. Liquids, especially alcoholic ones, are often sold in units whose origins are historical (e.g., beer in pints in the UK, champagne in ]s in France). | |||
| * In the US, ] measurements are recorded in milligrams per decilitre (mg/dL); in Canada and Europe, the standard is millimole per litre (mmol/L) or mM (millimolar). | |||
| * ] is measured in mmHg instead of Pa. | |||
| === Unit names === | |||
| The fine-tuning which has happened to the metric base unit definitions over the past 200 years, as experts have tried periodically to find more precise and reproducible methods, does not affect the everyday use of metric units. Since most non-SI units in common use, such as the ], are nowadays defined in terms of SI units, any change in the definition of the SI units results in a change of the definition of the older units, as well. | |||
| According to the SI Brochure,<ref name="SIBrochure9thEd" />{{rp|page=148}} unit names should be treated as ]s of the context language. This means that they should be typeset in the same character set as other common nouns (e.g. ] in English, ] in Russian, etc.), following the usual grammatical and ] rules of the context language. For example, in English and French, even when the unit is named after a person and its symbol begins with a capital letter, the unit name in running text should start with a lowercase letter (e.g., newton, hertz, pascal) and is ] only at the beginning of a sentence and in ]. As a nontrivial application of this rule, the SI Brochure notes<ref name="SIBrochure9thEd" />{{rp|page=148}} that the name of the unit with the symbol {{val|u=degC}} is correctly spelled as 'degree ]': the first letter of the name of the unit, 'd', is in lowercase, while the modifier 'Celsius' is capitalised because it is a proper name.<ref name="SIBrochure9thEd" />{{rp|page=148}} | |||
| ===Trade=== | |||
| The ] has a directive <ref>'''', with '''' (which changed the cutoff date in article 3.2 to ] ]) and '''' (which further changed the date to ] ]). Retrieved on ].</ref> banning non-SI markings after ] ] on any goods imported into the European Union. This applies to all markings on products, enclosed directions and papers, packaging, and advertisements. However, on ] ], the EU announced that the ] would be excepted from this directive, and ] measurements (or, for that matter, any other kind of non-SI marking, such as "pixels" or "kilobytes") would still be permitted alongside with the metric system as supplementary indications. <ref>http://news.bbc.co.uk/1/hi/uk/6988521.stm</ref> | |||
| The English spelling and even names for certain SI units and metric prefixes depend on the variety of English used. ] uses the spelling ''deka-'', ''meter'', and ''liter'', and ] uses ''deca-'', ''metre'', and ''litre''. The name of the unit whose symbol is t and which is defined according to {{val|1|u=t}} {{=}} {{val|e=3|u=kg}} is 'metric ton' in US English and 'tonne' in International English.<ref name="NIST330"/>{{rp|page=iii}} | |||
| === Unit symbols and the values of quantities === | |||
| Symbols of SI units are intended to be unique and universal, independent of the context language.<ref name= "SIBrochure" />{{rp|pages=130–135}} The SI Brochure has specific rules for writing them.<ref name= "SIBrochure" />{{rp|pages=130–135}} | |||
| In addition, the SI Brochure provides style conventions for among other aspects of displaying quantities units: the quantity symbols, formatting of numbers and the decimal marker, expressing measurement uncertainty, multiplication and division of quantity symbols, and the use of pure numbers and various angles.<ref name="SIBrochure9thEd"/>{{rp|page=147}} | |||
| In the United States, the guideline produced by the ] (NIST)<ref name= "NIST811">{{cite report |url=https://www.nist.gov/pml/special-publication-811 |title=Guide for the Use of the International System of Units (SI) |last1=Thompson |first1=Ambler |last2=Taylor |first2=Barry N. |date=March 2008 |publisher=] | access-date=21 January 2022 |at=§10.5.3}}</ref>{{rp|page=37}} clarifies language-specific details for American English that were left unclear by the SI Brochure, but is otherwise identical to the SI Brochure.<ref name="NISTInterprepation">{{cite journal |date=9 May 2008 |url=http://edocket.access.gpo.gov/2008/pdf/E8-11058.pdf |title=Interpretation of the International System of Units (the Metric System of Measurement) for the United States |journal=Federal Register |volume=73 |issue=96 |pages=28432–28433 |id=FR Doc number E8-11058 |access-date=28 October 2009}}</ref> For example, since 1979, the ] may exceptionally be written using either an uppercase "L" or a lowercase "l", a decision prompted by the similarity of the lowercase letter "l" to the numeral "1", especially with certain typefaces or English-style handwriting. The American NIST recommends that within the United States "L" be used rather than "l".<ref name="NIST811" /> | |||
| == Realisation of units <span class="anchor" id="Realisation"></span> == | |||
| {{Main|Realisation (metrology)}} | |||
| ] used for measuring the Avogadro constant to a relative ] of {{val|2|e=−8}} or less, held by ]<ref>{{cite web |title=Avogadro Project |url=http://www.npl.co.uk/science-technology/mass-and-force/research/avogadro-project |publisher=National Physical Laboratory |access-date=19 August 2010}}</ref>]] | |||
| Metrologists carefully distinguish between the definition of a unit and its realisation. The SI units are defined by declaring that seven ''defining constants''<ref name="SIBrochure9thEd" />{{rp|pages=125–129}} have certain exact numerical values when expressed in terms of their SI units. The realisation of the definition of a unit is the procedure by which the definition may be used to establish the value and associated uncertainty of a quantity of the same kind as the unit.<ref name="SIBrochure9thEd"/>{{rp|page=135}} | |||
| For each base unit the BIPM publishes a {{lang|fr|mises en pratique}}, (] for 'putting into practice; implementation',<ref name="NIST mise en pratique">{{cite journal |url=https://www.nist.gov/programs-projects/nist-mise-en-pratique-new-kilogram-definition |title=NIST Mise en Pratique of the New Kilogram Definition |journal=] | date=2013 |access-date=9 May 2020 |archive-url=https://web.archive.org/web/20170714202843/https://www.nist.gov/programs-projects/nist-mise-en-pratique-new-kilogram-definition |archive-date=14 July 2017 |url-status=live}}</ref>) describing the current best practical realisations of the unit.<ref name="MisesEnPratique">{{cite web |url=https://www.bipm.org/en/publications/mises-en-pratique/ |title=Practical realizations of the definitions of some important units |publisher=] | date=2019 |access-date=11 April 2020 |archive-url=https://web.archive.org/web/20200409115245/http://www.bipm.org/en/publications/mises-en-pratique/ |archive-date=9 April 2020 |url-status=live}}</ref> The separation of the defining constants from the definitions of units means that improved measurements can be developed leading to changes in the {{lang|fr|mises en pratique}} as science and technology develop, without having to revise the definitions. | |||
| The published {{lang|fr|mise en pratique}} is not the only way in which a base unit can be determined: the SI Brochure states that "any method consistent with the laws of physics could be used to realise any SI unit".<ref name="SIBrochure"/>{{rp|page=111}} Various consultative committees of the ] decided in 2016 that more than one {{lang|fr|mise en pratique}} would be developed for determining the value of each unit.<ref>{{cite web|title=International Committee for Weights and Measures – Proceedings of the 106th meeting|url=https://www.bipm.org/utils/en/pdf/CIPM/CIPM2017-EN.pdf}}</ref> These methods include the following: | |||
| * At least three separate experiments be carried out yielding values having a relative ] in the determination of the ] of no more than {{val|5|e=-8}} and at least one of these values should be better than {{val|2|e=-8}}. Both the ] and the ] should be included in the experiments and any differences between these be reconciled.<ref>{{cite web |url=http://www.bipm.org/utils/common/pdf/CCM12.pdf#page=23 |title=Recommendations of the Consultative Committee for Mass and Related Quantities to the International Committee for Weights and Measures |website=12th Meeting of the CCM |date=26 March 2010 |publisher=Bureau International des Poids et Mesures |location=Sèvres |access-date=27 June 2012 |archive-url=https://web.archive.org/web/20130514081750/http://www.bipm.org/utils/common/pdf/CCM12.pdf#page=23 |archive-date=14 May 2013 |url-status=dead}}</ref><ref>{{cite web |url=http://www.bipm.org/utils/common/pdf/CCQM16.pdf#page=40 |title=Recommendations of the Consultative Committee for Amount of Substance – Metrology in Chemistry to the International Committee for Weights and Measures |website=16th Meeting of the CCQM |date=15–16 April 2010 |publisher=Bureau International des Poids et Mesures |location=Sèvres |access-date=27 June 2012 |archive-url=https://web.archive.org/web/20130514072057/http://www.bipm.org/utils/common/pdf/CCQM16.pdf#page=40 |archive-date=14 May 2013 |url-status=dead}}</ref> | |||
| * The definition of the ] measured with a relative uncertainty of the ] derived from two fundamentally different methods such as acoustic gas ] and dielectric constant gas thermometry be better than one part in {{val|e=-6}} and that these values be corroborated by other measurements.<ref>{{cite web |url=http://www.bipm.org/utils/common/pdf/CCT25.pdf#page=53 |title=Recommendations of the Consultative Committee for Thermometry to the International Committee for Weights and Measures |website=25th Meeting of the CCT |date=6–7 May 2010 |publisher=Bureau International des Poids et Mesures |location=Sèvres |access-date=27 June 2012 |archive-url=https://web.archive.org/web/20130514064646/http://www.bipm.org/utils/common/pdf/CCT25.pdf#page=53 |archive-date=14 May 2013 |url-status=dead}}</ref> | |||
| == Organizational status == | |||
| ] (SI), ], and ] systems as of 2019]] | |||
| The International System of Units, or SI,<ref name="SIBrochure9thEd">{{Citation |last=International Bureau of Weights and Measures |title=The International System of Units (SI) |date=Dec 2022 |url=https://www.bipm.org/documents/20126/41483022/SI-Brochure-9-EN.pdf |volume=2 |issue=1 |archive-url=https://web.archive.org/web/20211018184555/https://www.bipm.org/documents/20126/41483022/SI-Brochure-9.pdf/fcf090b2-04e6-88cc-1149-c3e029ad8232 |url-status=live |edition=9th |isbn=978-92-822-2272-0 |archive-date=18 October 2021 |author-link=New SI}}</ref>{{rp|page=123|quote=... since its establishment in 1960, the International System of Units has always been referred to as "the SI" in its shortened form.}} is a ] and ] ] established in 1960 and periodically updated since then. The SI has an ] in most countries, including ], ], and ], although these three countries are among the handful of nations that, to various degrees, also continue to use their customary systems. Nevertheless, with this nearly universal level of acceptance, the SI "has been used around the world as the preferred system of units, the basic language for science, technology, industry, and trade."<ref name="SIBrochure9thEd" />{{rp|pages=123,126}} | |||
| The only other types of measurement system that still have widespread use across the world are the ]. The ] are defined in terms of the SI.<ref name="Standards1959">{{cite book|author=United States. National Bureau of Standards|author-link=National Institute of Standards and Technology|title=Research Highlights of the National Bureau of Standards|url=https://books.google.com/books?id=4aWN-VRV1AoC&pg=PA13|access-date = 31 July 2019|year=1959|publisher=U.S. Department of Commerce, National Bureau of Standards|page=13}}</ref> | |||
| === International System of Quantities === | |||
| {{Main|International System of Quantities}} | |||
| The quantities and equations that provide the context in which the SI units are defined are now referred to as the '']'' (ISQ). | |||
| The ISQ is based on the ] underlying each of the ]. Other quantities, such as ], ], and ], are derived from these base quantities by clear, non-contradictory equations. The ISQ defines the quantities that are measured with the SI units.<ref>{{cite book |title=International vocabulary of metrology – Basic and general concepts and associated terms (VIM) |date=2012 |publisher=International Bureau of Weights and Measures (BIPM): Joint Committee for Guides in Metrology |edition=3rd |chapter-url=http://www.bipm.org/utils/common/documents/jcgm/JCGM_200_2012.pdf |access-date=28 March 2015 |chapter=1.16}}</ref> The ISQ is formalised, in part, in the international standard ], which was completed in 2009 with the publication of ],<ref>S. V. Gupta, ''Units of Measurement: Past, Present and Future. International System of Units'', p. 16, Springer, 2009. {{ISBN|3642007384}}.</ref> and has largely been revised in 2019–2020.<ref>{{Cite web |title=ISO 80000-1:2022 Quantities and units Part 1: General |url=https://www.iso.org/standard/76921.html}}</ref> | |||
| === Controlling authority === | |||
| {{main | General Conference on Weights and Measures | International Bureau of Weights and Measures }} | |||
| The SI is regulated and continually developed by three international organisations that were established in 1875 under the terms of the ]. They are the ] (CGPM{{efn|name="French CGPM"|From ]: {{lang|fr|Conférence générale des poids et mesures.}}}}),<ref name="FR Interpretation of the SI">{{cite journal |date=16 May 2008 |title=Interpretation of the International System of Units (the Metric System of Measurement) for the United States |url=https://www.federalregister.gov/documents/2008/05/16/E8-11058/interpretation-of-the-international-system-of-units-the-metric-system-of-measurement-for-the-united |url-status=live |journal=] | publisher=] | volume=73 |page=28432 |archive-url=https://web.archive.org/web/20170816003324/https://www.federalregister.gov/documents/2008/05/16/E8-11058/interpretation-of-the-international-system-of-units-the-metric-system-of-measurement-for-the-united |archive-date=16 August 2017 |access-date=6 December 2022}}</ref> the International Committee for Weights and Measures (CIPM{{efn|name="French CIPM"|from {{langx|fr|Comité international des poids et mesures}}}}), and the ] (BIPM{{efn|name="BIPM from French"|from {{langx|fr|Bureau international des poids et mesures}}}}). | |||
| {{Anchor|SI Brochure}}All the decisions and recommendations concerning units are collected in a brochure called ''The International System of Units (SI)'',<ref name="SIBrochure9thEd" /> which is published in French and English by the BIPM and periodically updated. The writing and maintenance of the brochure is carried out by one of the committees of the CIPM. The definitions of the terms "quantity", "unit", "dimension", etc. that are used in the ''SI Brochure'' are those given in the ].<ref>{{cite web|url=http://www.bipm.org/en/publications/guides/vim.html|title= VIM3: International Vocabulary of Metrology |website=BIPM |url-status=dead |archive-url= https://web.archive.org/web/20201031042511/http://www.bipm.org/en/publications/guides/vim.html |archive-date= 31 October 2020 }}</ref> The brochure leaves some scope for local variations, particularly regarding unit names and terms in different languages. For example, the United States' ] (NIST) has produced a version of the CGPM document (NIST SP 330) which clarifies usage for English-language publications that use ].<ref name="NIST330">{{cite book |editor1=David B. Newell |editor2=Eite Tiesinga |title=The International System of Units (SI) |url=https://nvlpubs.nist.gov/nistpubs/SpecialPublications/NIST.SP.330-2019.pdf |access-date=30 November 2019 |publisher=] | location=Gaithersburg, MD |date=2019|edition=NIST Special publication 330, 2019}}</ref> | |||
| == History == | |||
| ] the ]/Italian border at ] displaying ]s, a unit of 10 km used in ] in the 19th century (but since ])<ref name="Europa1842">{{cite web |url=http://www.spasslernen.de/geschichte/groessen/mas1.htm |date= 1 May 2009 |website=spasslernen |title=Amtliche Maßeinheiten in Europa 1842 |language=de |trans-title=Official units of measure in Europe 1842 |access-date=26 March 2011 |url-status=dead |archive-url=https://web.archive.org/web/20120925070621/http://www.spasslernen.de/geschichte/groessen/mas1.htm |archive-date= 25 September 2012 }} Text version of Malaisé's book: {{cite book |url=https://archive.org/details/bub_gb_TQgHAAAAcAAJ |title=Theoretisch-practischer Unterricht im Rechnen |publisher=Verlag des Verf. |language=de |trans-title=Theoretical and practical instruction in arithmetic |author-first= Ferdinand von |author-last=Malaisé |place=München |date=1842 |pages=307–322 |access-date=7 January 2013}}</ref>]] | |||
| {{broader|History of the metric system}} | |||
| === CGS and MKS systems === | |||
| {{See also|CGS system of units}} | |||
| ] | |||
| The concept of a system of units emerged a hundred years before the SI. | |||
| In the 1860s, ], ] (later Lord Kelvin), and others working under the auspices of the ], building on previous work of ], developed the ] or cgs system in 1874. The systems formalised the concept of a collection of related units called a ''coherent'' system of units. In a coherent system, ''base units'' combine to define ''derived units'' without extra factors.<ref name=NIST330/>{{rp|page=2}} For example, using meters per second is coherent in a system that uses meter for length and seconds for time, but kilometre per hour is not coherent. The principle of coherence was successfully used to define a number of units of measure based on the CGS, including the ] for ], the ] for ], the ] for ], the ] for ] and the ] for ].<ref name="BIPMCentenary">{{cite book |url=https://archive.org/details/internationalbur420page |page= |title=The International Bureau of Weights and Measures 1875–1975: NBS Special Publication 420 |date=20 May 1975 |editor-last1=Page | |||
| | editor-first1=Chester H. |editor-last2=Vigoureux |editor-first2=Paul |publisher=] | location=Washington, D.C.}}</ref> | |||
| === Metre Convention === | |||
| {{Main|Metre Convention |MKS system of units}} | |||
| A French-inspired initiative for international cooperation in ] led to the signing in 1875 of the ], also called Treaty of the Metre, by 17 nations.{{efn|Argentina, Austria-Hungary, Belgium, Brazil, Denmark, France, German Empire, Italy, Peru, Portugal, Russia, Spain, Sweden and Norway, Switzerland, Ottoman Empire, United States, and Venezuela.}}<ref name="Alder">{{cite book |title=The Measure of all Things – The Seven-Year-Odyssey that Transformed the World |author-last=Alder |author-first=Ken |date=2002 |publisher=Abacus |location=London |isbn=978-0-349-11507-8}}</ref>{{rp|pages=353–354}} | |||
| The ] (French: {{lang|fr|Conférence générale des poids et mesures}} – CGPM), which was established by the Metre Convention,<ref name="BIPMCentenary"/> brought together many international organisations to establish the definitions and standards of a new system and to standardise the rules for writing and presenting measurements.<ref name=Giunta/>{{rp|page=37}}<ref>{{Cite book |last=Quinn |first=Terry J. |title=From artefacts to atoms: the BIPM and the search for ultimate measurement standards |date=2012 |publisher=Oxford University Press |isbn=978-0-19-530786-3 |location=New York Oxford}}</ref> | |||
| Initially the convention only covered standards for the metre and the kilogram. This became the foundation of the MKS system of units.<ref name=NIST330/>{{rp|page=2}} | |||
| === Giovanni Giorgi and the problem of electrical units === | |||
| At the close of the 19th century three different systems of units of measure existed for electrical measurements: a ], also known as the Gaussian or ESU system, a ] (EMU), and an International system based on units defined by the Metre Convention<ref>{{cite book |title=Weights, Measures and Units |url=https://archive.org/details/dictionaryofweig0000fenn |url-access=registration |author-first=Donald |author-last=Fenna |at=International unit |isbn=978-0-19-860522-5 |publisher=] | date=2002}}</ref> for electrical distribution systems. | |||
| Attempts to resolve the electrical units in terms of length, mass, and time using ] was beset with difficulties – the dimensions depended on whether one used the ESU or EMU systems.<ref name="Maxwell2">{{cite book |title=A treatise on electricity and magnetism |volume=2 |author-first=J. C. |author-last=Maxwell |date=1873 |publisher=Clarendon Press |location=Oxford |url=https://archive.org/stream/electricandmag02maxwrich |pages=242–245 |access-date=12 May 2011}}</ref> This anomaly was resolved in 1901 when ] published a paper in which he advocated using a fourth base unit alongside the existing three base units. The fourth unit could be chosen to be ], ], or ].<ref name="IECGiorgi">{{cite web |url=http://www.iec.ch/about/history/beginning/giovanni_giorgi.htm |title=Historical figures: Giovanni Giorgi |date=2011 |publisher=] | access-date=5 April 2011 |archive-date=15 May 2011 |archive-url=https://web.archive.org/web/20110515134553/http://www.iec.ch/about/history/beginning/giovanni_giorgi.htm |url-status=dead}}</ref> | |||
| Electric current with named unit 'ampere' was chosen as the base unit, and the other electrical quantities derived from it according to the laws of physics. | |||
| When combined with the MKS the new system, known as MKSA, was approved in 1946.<ref name=NIST330/> | |||
| === 9th CGPM, the precursor to SI === | |||
| In 1948, the 9th CGPM commissioned a study to assess the measurement needs of the scientific, technical, and educational communities and "to make recommendations for a single practical system of units of measurement, suitable for adoption by all countries adhering to the Metre Convention".<ref>{{cite web|url=http://www.bipm.org/en/CGPM/db/9/6/|title=BIPM – Resolution 6 of the 9th CGPM|website=Bipm.org|access-date=22 August 2017|date=1948}}</ref> This working document was ''Practical system of units of measurement''. Based on this study, the 10th CGPM in 1954 defined an international system derived six base units: the metre, kilogram, second, ampere, degree Kelvin, and candela. | |||
| The 9th CGPM also approved the first formal recommendation for the writing of symbols in the metric system when the basis of the rules as they are now known was laid down.<ref>{{cite web |url=http://www.bipm.org/en/CGPM/db/9/7/ |title=Resolution 7 of the 9th meeting of the CGPM (1948): Writing and printing of unit symbols and of numbers |access-date=6 November 2012 |publisher=]}}</ref> These rules were subsequently extended and now cover unit symbols and names, prefix symbols and names, how quantity symbols should be written and used, and how the values of quantities should be expressed.<ref name="SIBrochure"/>{{rp|pages=104,130}} | |||
| === Birth of the SI === | |||
| The 10th CGPM in 1954 resolved to create an international system of units<ref name=Giunta>{{Cite book |last=Giunta |first=Carmen J. |url=https://link.springer.com/10.1007/978-3-031-28436-6 |title=A Brief History of the Metric System: From Revolutionary France to the Constant-Based SI |date=2023 |publisher=Springer International Publishing |isbn=978-3-031-28435-9 |series=SpringerBriefs in Molecular Science |location=Cham |language=en |doi=10.1007/978-3-031-28436-6|bibcode=2023bhms.book.....G |s2cid=258172637 }}</ref>{{rp|page=41}} and | |||
| in 1960, the 11th CGPM adopted the ''International System of Units'', abbreviated SI from the French name {{lang|fr|Le Système international d'unités}}, which included a specification for units of measurement.<ref name="SIBrochure"/>{{rp|page=110}} | |||
| The ] (BIPM) has described SI as "the modern form of metric system".<ref name="SIBrochure"/>{{rp|page=95}} In 1971 the ] became the seventh base unit of the SI.<ref name=NIST330/>{{rp|page=2}} | |||
| === 2019 redefinition === | |||
| ]s, which are assigned exact numerical values in the ]. Unlike in the previous definitions, the base units are all derived exclusively from constants of nature. Here, <math>a \rightarrow b</math> means that <math>a</math> is used to define <math>b</math>.]] | |||
| {{Main|2019 revision of the SI}} | |||
| After the ] in 1960, the ] (IPK) was the only physical artefact upon which base units (directly the kilogram and indirectly the ampere, mole and candela) depended for their definition, making these units subject to periodic comparisons of national standard kilograms with the IPK.<ref name="NPL kg">{{cite web |title=Redefining the kilogram |url=http://www.npl.co.uk/educate-explore/redefining-the-kilogram/|publisher=UK National Physical Laboratory |access-date=30 November 2014}}</ref> During the 2nd and 3rd Periodic Verification of National Prototypes of the Kilogram, a significant divergence had occurred between the mass of the IPK and all of its official copies stored around the world: the copies had all noticeably increased in mass with respect to the IPK. During ''extraordinary verifications'' carried out in 2014 preparatory to redefinition of metric standards, continuing divergence was not confirmed. Nonetheless, the residual and irreducible instability of a physical IPK undermined the reliability of the entire metric system to precision measurement from small (atomic) to large (astrophysical) scales.<ref>{{Cite web |date=12 May 2018 |title=A Turning Point for Humanity: Redefining the World's Measurement System |url=https://www.nist.gov/si-redefinition/turning-point-humanity-redefining-worlds-measurement-system |access-date=16 January 2024 |website=NIST |language=en}}</ref> | |||
| By avoiding the use of an artefact to define units, all issues with the loss, damage, and change of the artefact are avoided.<ref name=SIBrochure9thEd/>{{rp|page=125}} | |||
| A proposal was made that:<ref>{{cite web |title=Appendix 1. Decisions of the CGPM and the CIPM |url=https://www.bipm.org/documents/20126/41483022/SI-Brochure-9-App4-EN.pdf |page=188 |publisher=] | access-date=27 April 2021}}</ref> | |||
| * In addition to the speed of light, four constants of nature – the ], an ], the ], and the ] – be defined to have exact values | |||
| * The ] be retired | |||
| * The current definitions of the kilogram, ampere, kelvin, and mole be revised | |||
| * The wording of base unit definitions should change emphasis from explicit unit to explicit constant definitions. | |||
| The new definitions were adopted at the 26th CGPM on 16 November 2018, and came into effect on 20 May 2019.<ref>{{cite web |url=http://www.bipm.org/cc/TGFC/Allowed/Minutes/CODATA_Minutes_14-BIPM-public.pdf |title=Report on the Meeting of the CODATA Task Group on Fundamental Constants |date=3–4 November 2014 |publisher=] | author-first=B. |author-last=Wood |page=7 |quote= Milton responded to a question about what would happen if ... the CIPM or the CGPM voted not to move forward with the redefinition of the SI. He responded that he felt that by that time the decision to move forward should be seen as a foregone conclusion.}}</ref> The change was adopted by the European Union through Directive (EU) 2019/1258.<ref>{{cite web |url=https://eur-lex.europa.eu/eli/dir/2019/1258/oj |title=Commission Directive (EU) 2019/1258 of 23 July 2019 amending, for the purpose of its adaptation to technical progress, the Annex to Council Directive 80/181/EEC as regards the definitions of SI base units |author=<!--Not stated--> |date=23 July 2019 |website=] | access-date=28 August 2019}}</ref> | |||
| Prior to its redefinition in 2019, the SI was defined through the seven base units from which the derived units were constructed as products of powers of the base units. After the redefinition, the SI is defined by fixing the numerical values of seven defining constants. This has the effect that the distinction between the base units and derived units is, in principle, not needed, since all units, base as well as derived, may be constructed directly from the defining constants. Nevertheless, the distinction is retained because "it is useful and historically well established", and also because the ] series of standards, which define the ] (ISQ), specifies base and derived quantities that necessarily have the corresponding SI units.<ref name="SIBrochure9thEd" />{{rp|page=129}} | |||
| == Related units == | |||
| === Non-SI units accepted for use with SI === | |||
| {{Main|Non-SI units mentioned in the SI}} | |||
| ] | |||
| Many non-SI units continue to be used in the scientific, technical, and commercial literature. Some units are deeply embedded in history and culture, and their use has not been entirely replaced by their SI alternatives. The CIPM recognised and acknowledged such traditions by compiling a list of ],<ref name="SIBrochure"/> including the hour, minute, degree of angle, litre, and decibel. | |||
| === Metric units not recognised by SI === | |||
| {{Main|List of metric units}} | |||
| Although the term ''metric system'' is often used as an informal alternative name for the International System of Units,<ref name="MetricSystemAsNameForSI">{{cite journal |last=Olthoff|first=Jim|url=https://www.nist.gov/blogs/taking-measure/all-times-all-peoples-how-replacing-kilogram-empowers-industry |title=For All Times, For All Peoples: How Replacing the Kilogram Empowers Industry |journal=] | date=2018 |access-date=14 April 2020 |archive-url=https://web.archive.org/web/20200316195625/https://www.nist.gov/blogs/taking-measure/all-times-all-peoples-how-replacing-kilogram-empowers-industry |archive-date=16 March 2020 |url-status=live |quote=... the International System of Units (SI), popularly known as the metric system.}}</ref> other metric systems exist, some of which were in widespread use in the past or are even still used in particular areas. There are also individual ] such as the ] and the ] that exist outside of any system of units. Most of the units of the other metric systems are not recognised by the SI. | |||
| === Unacceptable uses === | |||
| Sometimes, SI unit name variations are introduced, mixing information about the corresponding physical quantity or the conditions of its measurement; however, this practice is unacceptable with the SI. "Unacceptability of mixing information with units: When one gives the value of a quantity, any information concerning the quantity or its conditions of measurement must be presented in such a way as not to be associated with the unit."<ref name="SIBrochure"/> | |||
| Instances include: "]" and "]"; "]" and "]"; "]"; "]", "]", and "]". | |||
| == See also == | == See also == | ||
| {| | |||
| {{Columns-list|colwidth=30em| | |||
| |-valign=top | |||
| * {{annotated link|Conversion of units}} | |||
| | | |||
| * {{annotated link|List of international common standards}} | |||
| * ] | |||
| * ] | |||
| * ] | |||
| | width=40 | | |||
| | | |||
| * ] | |||
| * ] | * ] | ||
| * {{annotated link|Outline of the metric system}} | |||
| * ] | |||
| |- | |||
| {{br}} | |||
| |<br>'''Organisations | |||
| '''Organisations''' | |||
| |-valign=top | |||
| * {{annotated link|International Bureau of Weights and Measures}} | |||
| | | |||
| * {{annotated link|Institute for Reference Materials and Measurements}} (EU) | |||
| * ] (BIPM) | |||
| * {{annotated link|National Institute of Standards and Technology}} (US) | |||
| * ] (IRMM) | |||
| '''Standards and conventions''' | |||
| | width=40 | | |||
| * {{annotated link|Conventional electrical unit}} | |||
| | | |||
| * {{annotated link|Coordinated Universal Time|abbreviation=UTC}} | |||
| * ] | |||
| * {{annotated link|Unified Code for Units of Measure}} | |||
| |- | |||
| }} | |||
| |<br>'''Standards and conventions | |||
| |-valign=top | |||
| == Notes == | |||
| | | |||
| * ] | |||
| {{notelist}} | |||
| * ] | |||
| * ] | |||
| ; Attribution | |||
| * ] | |||
| <ref name="SIBrochure9thEd" />{{Creative Commons text attribution notice|cc=by3|url=https://www.bipm.org/documents/20126/41483022/SI-Brochure-9-EN.pdf|authors=Bureau International des Poids et Mesures|from this source=yes}} | |||
| | width=40 | | |||
| | | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] (UTC) | |||
| |} | |||
| <br> | |||
| {{systems of measurement}} | |||
| == References == | == References == | ||
| {{Reflist}} | |||
| {{reflist}} | |||
| == Further reading == | == Further reading == | ||
| * {{GreenBookRef}} | |||
| {{refbegin}} | |||
| * {{GreenBook2nd}} | |||
| * {{Webarchive|url=https://web.archive.org/web/20201030081637/http://info.ee.surrey.ac.uk/Workshop/advice/coils/unit_systems/#rms |date=30 October 2020 }} | |||
| * (PDF) Metrology Triangle Using a Watt Balance, a Calculable Capacitor, and a Single-Electron Tunnelling Device | |||
| * (PDF). Barry N. Taylor. Journal of Research of the National Institute of Standards and Technology, Vol. 116, No. 6, Pgs. 797–807, Nov–Dec 2011. | |||
| * B. N. Taylor, Ambler Thompson, ''International System of Units (SI)'', ] 2008 edition, {{ISBN|1437915582}}. | |||
| {{refend}} | |||
| == External links == | == External links == | ||
| ;Official | |||
| * (home page) | |||
| ** (SI reference) | |||
| * | |||
| ** | |||
| * | |||
| ** | |||
| * | |||
| * (ANSI approved, joint IEEE/ASTM standard) | |||
| * | |||
| {{Commons category|International System of Units}} | |||
| ;Information | |||
| {{Refbegin}} | |||
| *{{dmoz|Science/Reference/Units_of_Measurement/}} | |||
| * | |||
| * Online Categorized Metric Conversion Calculator | |||
| {{Refend}} | |||
| {{SI units}} | |||
| ;History | |||
| {{Systems of measurement}} | |||
| * gives a historical background to the SI system. | |||
| {{Portal bar|Physics}} | |||
| {{Authority control}} | |||
| ;Pro-metric ] | |||
| * | |||
| * | |||
| * | |||
| * | |||
| * | |||
| ] | |||
| ;Pro-customary measures pressure groups | |||
| ] | |||
| *{{dmoz|Society/Issues/Government_Operations/Anti-Metrication/|Pro-customary measures groups}} | |||
| ] | |||
| ] | ] | ||
| ] | |||
| {{Link FA|bg}} | |||
| {{Link FA|vi}} | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
Latest revision as of 20:40, 29 December 2024
Modern form of the metric system "SI" redirects here. For the chemical element with symbol Si, see Silicon. For other uses, see SI (disambiguation).The International System of Units, internationally known by the abbreviation SI (from French Système international d'unités), is the modern form of the metric system and the world's most widely used system of measurement. It is the only system of measurement with official status in nearly every country in the world, employed in science, technology, industry, and everyday commerce. The SI system is coordinated by the International Bureau of Weights and Measures which is abbreviated BIPM from French: Bureau international des poids et mesures.

| Symbol | Name | Quantity |
|---|---|---|
| s | second | time |
| m | metre | length |
| kg | kilogram | mass |
| A | ampere | electric current |
| K | kelvin | thermodynamic temperature |
| mol | mole | amount of substance |
| cd | candela | luminous intensity |
The SI comprises a coherent system of units of measurement starting with seven base units, which are the second (symbol s, the unit of time), metre (m, length), kilogram (kg, mass), ampere (A, electric current), kelvin (K, thermodynamic temperature), mole (mol, amount of substance), and candela (cd, luminous intensity). The system can accommodate coherent units for an unlimited number of additional quantities. These are called coherent derived units, which can always be represented as products of powers of the base units. Twenty-two coherent derived units have been provided with special names and symbols.
The seven base units and the 22 coherent derived units with special names and symbols may be used in combination to express other coherent derived units. Since the sizes of coherent units will be convenient for only some applications and not for others, the SI provides twenty-four prefixes which, when added to the name and symbol of a coherent unit produce twenty-four additional (non-coherent) SI units for the same quantity; these non-coherent units are always decimal (i.e. power-of-ten) multiples and sub-multiples of the coherent unit.
The current way of defining the SI is a result of a decades-long move towards increasingly abstract and idealised formulation in which the realisations of the units are separated conceptually from the definitions. A consequence is that as science and technologies develop, new and superior realisations may be introduced without the need to redefine the unit. One problem with artefacts is that they can be lost, damaged, or changed; another is that they introduce uncertainties that cannot be reduced by advancements in science and technology.
The original motivation for the development of the SI was the diversity of units that had sprung up within the centimetre–gram–second (CGS) systems (specifically the inconsistency between the systems of electrostatic units and electromagnetic units) and the lack of coordination between the various disciplines that used them. The General Conference on Weights and Measures (French: Conférence générale des poids et mesures – CGPM), which was established by the Metre Convention of 1875, brought together many international organisations to establish the definitions and standards of a new system and to standardise the rules for writing and presenting measurements. The system was published in 1960 as a result of an initiative that began in 1948, and is based on the metre–kilogram–second system of units (MKS) combined with ideas from the development of the CGS system.
Definition
The International System of Units consists of a set of defining constants with corresponding base units, derived units, and a set of decimal-based multipliers that are used as prefixes.
SI defining constants
| Symbol | Defining constant | Exact value |
|---|---|---|
| ΔνCs | hyperfine transition frequency of Cs | 9192631770 Hz |
| c | speed of light | 299792458 m/s |
| h | Planck constant | 6.62607015×10 J⋅s |
| e | elementary charge | 1.602176634×10 C |
| k | Boltzmann constant | 1.380649×10 J/K |
| NA | Avogadro constant | 6.02214076×10 mol |
| Kcd | luminous efficacy of 540 THz radiation | 683 lm/W |
The seven defining constants are the most fundamental feature of the definition of the system of units. The magnitudes of all SI units are defined by declaring that seven constants have certain exact numerical values when expressed in terms of their SI units. These defining constants are the speed of light in vacuum c, the hyperfine transition frequency of caesium ΔνCs, the Planck constant h, the elementary charge e, the Boltzmann constant k, the Avogadro constant NA, and the luminous efficacy Kcd. The nature of the defining constants ranges from fundamental constants of nature such as c to the purely technical constant Kcd. The values assigned to these constants were fixed to ensure continuity with previous definitions of the base units.
SI base units
Main article: SI base unitThe SI selects seven units to serve as base units, corresponding to seven base physical quantities. They are the second, with the symbol s, which is the SI unit of the physical quantity of time; the metre, symbol m, the SI unit of length; kilogram (kg, the unit of mass); ampere (A, electric current); kelvin (K, thermodynamic temperature); mole (mol, amount of substance); and candela (cd, luminous intensity). The base units are defined in terms of the defining constants. For example, the kilogram is defined by taking the Planck constant h to be 6.62607015×10 J⋅s, giving the expression in terms of the defining constants
- 1 kg = (299792458)/(6.62607015×10)(9192631770)h ΔνCs/c.
All units in the SI can be expressed in terms of the base units, and the base units serve as a preferred set for expressing or analysing the relationships between units. The choice of which and even how many quantities to use as base quantities is not fundamental or even unique – it is a matter of convention.
| Unit name | Unit symbol | Dimension symbol | Quantity name | Typical symbols | Definition |
|---|---|---|---|---|---|
| second | s | time | The duration of 9192631770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the caesium-133 atom. | ||
| metre | m | length | , , , etc. | The distance travelled by light in vacuum in 1/299792458 second. | |
| kilogram |
kg | mass | The kilogram is defined by setting the Planck constant h to 6.62607015×10 J⋅s (J = kg⋅m⋅s), given the definitions of the metre and the second. | ||
| ampere | A | electric current | The flow of 1/1.602176634×10 times the elementary charge e per second, which is approximately 6.2415090744×10 elementary charges per second. | ||
| kelvin | K | thermodynamic temperature |
The kelvin is defined by setting the fixed numerical value of the Boltzmann constant k to 1.380649×10 J⋅K, (J = kg⋅m⋅s), given the definition of the kilogram, the metre, and the second. | ||
| mole | mol | amount of substance | The amount of substance of 6.02214076×10 elementary entities. This number is the fixed numerical value of the Avogadro constant, NA, when expressed in the unit mol. | ||
| candela | cd | luminous intensity | The luminous intensity, in a given direction, of a source that emits monochromatic radiation of frequency 5.4×10 hertz and that has a radiant intensity in that direction of 1/683 watt per steradian. | ||
| |||||
Derived units
Main article: SI derived unitThe system allows for an unlimited number of additional units, called derived units, which can always be represented as products of powers of the base units, possibly with a nontrivial numeric multiplier. When that multiplier is one, the unit is called a coherent derived unit. For example, the coherent derived SI unit of velocity is the metre per second, with the symbol m/s. The base and coherent derived units of the SI together form a coherent system of units (the set of coherent SI units). A useful property of a coherent system is that when the numerical values of physical quantities are expressed in terms of the units of the system, then the equations between the numerical values have exactly the same form, including numerical factors, as the corresponding equations between the physical quantities.
Twenty-two coherent derived units have been provided with special names and symbols as shown in the table below. The radian and steradian have no base units but are treated as derived units for historical reasons.
| Name | Symbol | Quantity | In SI base units | In other SI units |
|---|---|---|---|---|
| radian | rad | plane angle | m/m | 1 |
| steradian | sr | solid angle | m/m | 1 |
| hertz | Hz | frequency | s | |
| newton | N | force | kg⋅m⋅s | |
| pascal | Pa | pressure, stress | kg⋅m⋅s | N/m = J/m |
| joule | J | energy, work, amount of heat | kg⋅m⋅s | N⋅m = Pa⋅m |
| watt | W | power, radiant flux | kg⋅m⋅s | J/s |
| coulomb | C | electric charge | s⋅A | |
| volt | V | electric potential, (voltage in US; electric tension) | kg⋅m⋅s⋅A | W/A = J/C |
| farad | F | capacitance | kg⋅m⋅s⋅A | C/V = C/J |
| ohm | Ω | resistance | kg⋅m⋅s⋅A | V/A = J⋅s/C |
| siemens | S | electrical conductance | kg⋅m⋅s⋅A | Ω |
| weber | Wb | magnetic flux | kg⋅m⋅s⋅A | V⋅s |
| tesla | T | magnetic flux density | kg⋅s⋅A | Wb/m |
| henry | H | inductance | kg⋅m⋅s⋅A | Wb/A |
| degree Celsius | °C | temperature | K | |
| lumen | lm | luminous flux | cd⋅m/m | cd⋅sr |
| lux | lx | illuminance | cd⋅m/m | lm/m = cd⋅sr⋅m |
| becquerel | Bq | activity referred to a radionuclide (decays per unit time) | s | |
| gray | Gy | absorbed dose, kerma | m⋅s | J/kg |
| sievert | Sv | equivalent dose | m⋅s | J/kg |
| katal | kat | catalytic activity | mol⋅s | |
| Notes | ||||
The derived units in the SI are formed by powers, products, or quotients of the base units and are unlimited in number.

Derived units apply to some derived quantities, which may by definition be expressed in terms of base quantities, and thus are not independent; for example, electrical conductance is the inverse of electrical resistance, with the consequence that the siemens is the inverse of the ohm, and similarly, the ohm and siemens can be replaced with a ratio of an ampere and a volt, because those quantities bear a defined relationship to each other. Other useful derived quantities can be specified in terms of the SI base and derived units that have no named units in the SI, such as acceleration, which has the SI unit m/s.
A combination of base and derived units may be used to express a derived unit. For example, the SI unit of force is the newton (N), the SI unit of pressure is the pascal (Pa) – and the pascal can be defined as one newton per square metre (N/m).
Prefixes
Main article: Metric prefixLike all metric systems, the SI uses metric prefixes to systematically construct, for the same physical quantity, a set of units that are decimal multiples of each other over a wide range. For example, driving distances are normally given in kilometres (symbol km) rather than in metres. Here the metric prefix 'kilo-' (symbol 'k') stands for a factor of 1000; thus, 1 km = 1000 m.
The SI provides twenty-four metric prefixes that signify decimal powers ranging from 10 to 10, the most recent being adopted in 2022. Most prefixes correspond to integer powers of 1000; the only ones that do not are those for 10, 1/10, 100, and 1/100. The conversion between different SI units for one and the same physical quantity is always through a power of ten. This is why the SI (and metric systems more generally) are called decimal systems of measurement units.
The grouping formed by a prefix symbol attached to a unit symbol (e.g. 'km', 'cm') constitutes a new inseparable unit symbol. This new symbol can be raised to a positive or negative power. It can also be combined with other unit symbols to form compound unit symbols. For example, g/cm is an SI unit of density, where cm is to be interpreted as (cm).
Prefixes are added to unit names to produce multiples and submultiples of the original unit. All of these are integer powers of ten, and above a hundred or below a hundredth all are integer powers of a thousand. For example, kilo- denotes a multiple of a thousand and milli- denotes a multiple of a thousandth, so there are one thousand millimetres to the metre and one thousand metres to the kilometre. The prefixes are never combined, so for example a millionth of a metre is a micrometre, not a millimillimetre. Multiples of the kilogram are named as if the gram were the base unit, so a millionth of a kilogram is a milligram, not a microkilogram.
The BIPM specifies 24 prefixes for the International System of Units (SI):
| Prefix | Base 10 | Decimal | Adoption | |
|---|---|---|---|---|
| Name | Symbol | |||
| quetta | Q | 10 | 1000000000000000000000000000000 | 2022 |
| ronna | R | 10 | 1000000000000000000000000000 | |
| yotta | Y | 10 | 1000000000000000000000000 | 1991 |
| zetta | Z | 10 | 1000000000000000000000 | |
| exa | E | 10 | 1000000000000000000 | 1975 |
| peta | P | 10 | 1000000000000000 | |
| tera | T | 10 | 1000000000000 | 1960 |
| giga | G | 10 | 1000000000 | |
| mega | M | 10 | 1000000 | 1873 |
| kilo | k | 10 | 1000 | 1795 |
| hecto | h | 10 | 100 | |
| deca | da | 10 | 10 | |
| — | — | 10 | 1 | — |
| deci | d | 10 | 0.1 | 1795 |
| centi | c | 10 | 0.01 | |
| milli | m | 10 | 0.001 | |
| micro | μ | 10 | 0.000001 | 1873 |
| nano | n | 10 | 0.000000001 | 1960 |
| pico | p | 10 | 0.000000000001 | |
| femto | f | 10 | 0.000000000000001 | 1964 |
| atto | a | 10 | 0.000000000000000001 | |
| zepto | z | 10 | 0.000000000000000000001 | 1991 |
| yocto | y | 10 | 0.000000000000000000000001 | |
| ronto | r | 10 | 0.000000000000000000000000001 | 2022 |
| quecto | q | 10 | 0.000000000000000000000000000001 | |
| ||||
Coherent and non-coherent SI units
Further information: Coherent unitThe base units and the derived units formed as the product of powers of the base units with a numerical factor of one form a coherent system of units. Every physical quantity has exactly one coherent SI unit. For example, 1 m/s = 1 m / (1 s) is the coherent derived unit for velocity. With the exception of the kilogram (for which the prefix kilo- is required for a coherent unit), when prefixes are used with the coherent SI units, the resulting units are no longer coherent, because the prefix introduces a numerical factor other than one. For example, the metre, kilometre, centimetre, nanometre, etc. are all SI units of length, though only the metre is a coherent SI unit. The complete set of SI units consists of both the coherent set and the multiples and sub-multiples of coherent units formed by using the SI prefixes.
The kilogram is the only coherent SI unit whose name and symbol include a prefix. For historical reasons, the names and symbols for multiples and sub-multiples of the unit of mass are formed as if the gram were the base unit. Prefix names and symbols are attached to the unit name gram and the unit symbol g respectively. For example, 10 kg is written milligram and mg, not microkilogram and μkg.
Several different quantities may share the same coherent SI unit. For example, the joule per kelvin (symbol J/K) is the coherent SI unit for two distinct quantities: heat capacity and entropy; another example is the ampere, which is the coherent SI unit for both electric current and magnetomotive force. This illustrates why it is important not to use the unit alone to specify the quantity. As the SI Brochure states, "this applies not only to technical texts, but also, for example, to measuring instruments (i.e. the instrument read-out needs to indicate both the unit and the quantity measured)".
Furthermore, the same coherent SI unit may be a base unit in one context, but a coherent derived unit in another. For example, the ampere is a base unit when it is a unit of electric current, but a coherent derived unit when it is a unit of magnetomotive force.
| Name | Symbol | Derived quantity | Typical symbol |
|---|---|---|---|
| square metre | m | area | A |
| cubic metre | m | volume | V |
| metre per second | m/s | speed, velocity | v |
| metre per second squared | m/s | acceleration | a |
| reciprocal metre | m | wavenumber | σ, ṽ |
| vergence (optics) | V, 1/f | ||
| kilogram per cubic metre | kg/m | density | ρ |
| kilogram per square metre | kg/m | surface density | ρA |
| cubic metre per kilogram | m/kg | specific volume | v |
| ampere per square metre | A/m | current density | j |
| ampere per metre | A/m | magnetic field strength | H |
| mole per cubic metre | mol/m | concentration | c |
| kilogram per cubic metre | kg/m | mass concentration | ρ, γ |
| candela per square metre | cd/m | luminance | Lv |
| Name | Symbol | Quantity | In SI base units |
|---|---|---|---|
| pascal-second | Pa⋅s | dynamic viscosity | m⋅kg⋅s |
| newton-metre | N⋅m | moment of force | m⋅kg⋅s |
| newton per metre | N/m | surface tension | kg⋅s |
| radian per second | rad/s | angular velocity, angular frequency | s |
| radian per second squared | rad/s | angular acceleration | s |
| watt per square metre | W/m | heat flux density, irradiance | kg⋅s |
| joule per kelvin | J/K | entropy, heat capacity | m⋅kg⋅s⋅K |
| joule per kilogram-kelvin | J/(kg⋅K) | specific heat capacity, specific entropy | m⋅s⋅K |
| joule per kilogram | J/kg | specific energy | m⋅s |
| watt per metre-kelvin | W/(m⋅K) | thermal conductivity | m⋅kg⋅s⋅K |
| joule per cubic metre | J/m | energy density | m⋅kg⋅s |
| volt per metre | V/m | electric field strength | m⋅kg⋅s⋅A |
| coulomb per cubic metre | C/m | electric charge density | m⋅s⋅A |
| coulomb per square metre | C/m | surface charge density, electric flux density, electric displacement | m⋅s⋅A |
| farad per metre | F/m | permittivity | m⋅kg⋅s⋅A |
| henry per metre | H/m | permeability | m⋅kg⋅s⋅A |
| joule per mole | J/mol | molar energy | m⋅kg⋅s⋅mol |
| joule per mole-kelvin | J/(mol⋅K) | molar entropy, molar heat capacity | m⋅kg⋅s⋅K⋅mol |
| coulomb per kilogram | C/kg | exposure (x- and γ-rays) | kg⋅s⋅A |
| gray per second | Gy/s | absorbed dose rate | m⋅s |
| watt per steradian | W/sr | radiant intensity | m⋅kg⋅s |
| watt per square metre-steradian | W/(m⋅sr) | radiance | kg⋅s |
| katal per cubic metre | kat/m | catalytic activity concentration | m⋅s⋅mol |
Lexicographic conventions
See also: ISO 31-0 § Typographic conventions, and Space (punctuation) § Unit symbols and numbers
Unit names
According to the SI Brochure, unit names should be treated as common nouns of the context language. This means that they should be typeset in the same character set as other common nouns (e.g. Latin alphabet in English, Cyrillic script in Russian, etc.), following the usual grammatical and orthographical rules of the context language. For example, in English and French, even when the unit is named after a person and its symbol begins with a capital letter, the unit name in running text should start with a lowercase letter (e.g., newton, hertz, pascal) and is capitalised only at the beginning of a sentence and in headings and publication titles. As a nontrivial application of this rule, the SI Brochure notes that the name of the unit with the symbol °C is correctly spelled as 'degree Celsius': the first letter of the name of the unit, 'd', is in lowercase, while the modifier 'Celsius' is capitalised because it is a proper name.
The English spelling and even names for certain SI units and metric prefixes depend on the variety of English used. US English uses the spelling deka-, meter, and liter, and International English uses deca-, metre, and litre. The name of the unit whose symbol is t and which is defined according to 1 t = 10 kg is 'metric ton' in US English and 'tonne' in International English.
Unit symbols and the values of quantities
Symbols of SI units are intended to be unique and universal, independent of the context language. The SI Brochure has specific rules for writing them.
In addition, the SI Brochure provides style conventions for among other aspects of displaying quantities units: the quantity symbols, formatting of numbers and the decimal marker, expressing measurement uncertainty, multiplication and division of quantity symbols, and the use of pure numbers and various angles.
In the United States, the guideline produced by the National Institute of Standards and Technology (NIST) clarifies language-specific details for American English that were left unclear by the SI Brochure, but is otherwise identical to the SI Brochure. For example, since 1979, the litre may exceptionally be written using either an uppercase "L" or a lowercase "l", a decision prompted by the similarity of the lowercase letter "l" to the numeral "1", especially with certain typefaces or English-style handwriting. The American NIST recommends that within the United States "L" be used rather than "l".
Realisation of units
Main article: Realisation (metrology)
Metrologists carefully distinguish between the definition of a unit and its realisation. The SI units are defined by declaring that seven defining constants have certain exact numerical values when expressed in terms of their SI units. The realisation of the definition of a unit is the procedure by which the definition may be used to establish the value and associated uncertainty of a quantity of the same kind as the unit.
For each base unit the BIPM publishes a mises en pratique, (French for 'putting into practice; implementation',) describing the current best practical realisations of the unit. The separation of the defining constants from the definitions of units means that improved measurements can be developed leading to changes in the mises en pratique as science and technology develop, without having to revise the definitions.
The published mise en pratique is not the only way in which a base unit can be determined: the SI Brochure states that "any method consistent with the laws of physics could be used to realise any SI unit". Various consultative committees of the CIPM decided in 2016 that more than one mise en pratique would be developed for determining the value of each unit. These methods include the following:
- At least three separate experiments be carried out yielding values having a relative standard uncertainty in the determination of the kilogram of no more than 5×10 and at least one of these values should be better than 2×10. Both the Kibble balance and the Avogadro project should be included in the experiments and any differences between these be reconciled.
- The definition of the kelvin measured with a relative uncertainty of the Boltzmann constant derived from two fundamentally different methods such as acoustic gas thermometry and dielectric constant gas thermometry be better than one part in 10 and that these values be corroborated by other measurements.
Organizational status

The International System of Units, or SI, is a decimal and metric system of units established in 1960 and periodically updated since then. The SI has an official status in most countries, including the United States, Canada, and the United Kingdom, although these three countries are among the handful of nations that, to various degrees, also continue to use their customary systems. Nevertheless, with this nearly universal level of acceptance, the SI "has been used around the world as the preferred system of units, the basic language for science, technology, industry, and trade."
The only other types of measurement system that still have widespread use across the world are the imperial and US customary measurement systems. The international yard and pound are defined in terms of the SI.
International System of Quantities
Main article: International System of QuantitiesThe quantities and equations that provide the context in which the SI units are defined are now referred to as the International System of Quantities (ISQ). The ISQ is based on the quantities underlying each of the seven base units of the SI. Other quantities, such as area, pressure, and electrical resistance, are derived from these base quantities by clear, non-contradictory equations. The ISQ defines the quantities that are measured with the SI units. The ISQ is formalised, in part, in the international standard ISO/IEC 80000, which was completed in 2009 with the publication of ISO 80000-1, and has largely been revised in 2019–2020.
Controlling authority
Main articles: General Conference on Weights and Measures and International Bureau of Weights and MeasuresThe SI is regulated and continually developed by three international organisations that were established in 1875 under the terms of the Metre Convention. They are the General Conference on Weights and Measures (CGPM), the International Committee for Weights and Measures (CIPM), and the International Bureau of Weights and Measures (BIPM). All the decisions and recommendations concerning units are collected in a brochure called The International System of Units (SI), which is published in French and English by the BIPM and periodically updated. The writing and maintenance of the brochure is carried out by one of the committees of the CIPM. The definitions of the terms "quantity", "unit", "dimension", etc. that are used in the SI Brochure are those given in the international vocabulary of metrology. The brochure leaves some scope for local variations, particularly regarding unit names and terms in different languages. For example, the United States' National Institute of Standards and Technology (NIST) has produced a version of the CGPM document (NIST SP 330) which clarifies usage for English-language publications that use American English.
History

CGS and MKS systems
See also: CGS system of units
The concept of a system of units emerged a hundred years before the SI. In the 1860s, James Clerk Maxwell, William Thomson (later Lord Kelvin), and others working under the auspices of the British Association for the Advancement of Science, building on previous work of Carl Gauss, developed the centimetre–gram–second system of units or cgs system in 1874. The systems formalised the concept of a collection of related units called a coherent system of units. In a coherent system, base units combine to define derived units without extra factors. For example, using meters per second is coherent in a system that uses meter for length and seconds for time, but kilometre per hour is not coherent. The principle of coherence was successfully used to define a number of units of measure based on the CGS, including the erg for energy, the dyne for force, the barye for pressure, the poise for dynamic viscosity and the stokes for kinematic viscosity.
Metre Convention
Main articles: Metre Convention and MKS system of unitsA French-inspired initiative for international cooperation in metrology led to the signing in 1875 of the Metre Convention, also called Treaty of the Metre, by 17 nations. The General Conference on Weights and Measures (French: Conférence générale des poids et mesures – CGPM), which was established by the Metre Convention, brought together many international organisations to establish the definitions and standards of a new system and to standardise the rules for writing and presenting measurements. Initially the convention only covered standards for the metre and the kilogram. This became the foundation of the MKS system of units.
Giovanni Giorgi and the problem of electrical units
At the close of the 19th century three different systems of units of measure existed for electrical measurements: a CGS-based system for electrostatic units, also known as the Gaussian or ESU system, a CGS-based system for electromechanical units (EMU), and an International system based on units defined by the Metre Convention for electrical distribution systems. Attempts to resolve the electrical units in terms of length, mass, and time using dimensional analysis was beset with difficulties – the dimensions depended on whether one used the ESU or EMU systems. This anomaly was resolved in 1901 when Giovanni Giorgi published a paper in which he advocated using a fourth base unit alongside the existing three base units. The fourth unit could be chosen to be electric current, voltage, or electrical resistance.
Electric current with named unit 'ampere' was chosen as the base unit, and the other electrical quantities derived from it according to the laws of physics. When combined with the MKS the new system, known as MKSA, was approved in 1946.
9th CGPM, the precursor to SI
In 1948, the 9th CGPM commissioned a study to assess the measurement needs of the scientific, technical, and educational communities and "to make recommendations for a single practical system of units of measurement, suitable for adoption by all countries adhering to the Metre Convention". This working document was Practical system of units of measurement. Based on this study, the 10th CGPM in 1954 defined an international system derived six base units: the metre, kilogram, second, ampere, degree Kelvin, and candela.
The 9th CGPM also approved the first formal recommendation for the writing of symbols in the metric system when the basis of the rules as they are now known was laid down. These rules were subsequently extended and now cover unit symbols and names, prefix symbols and names, how quantity symbols should be written and used, and how the values of quantities should be expressed.
Birth of the SI
The 10th CGPM in 1954 resolved to create an international system of units and in 1960, the 11th CGPM adopted the International System of Units, abbreviated SI from the French name Le Système international d'unités, which included a specification for units of measurement.
The International Bureau of Weights and Measures (BIPM) has described SI as "the modern form of metric system". In 1971 the mole became the seventh base unit of the SI.
2019 redefinition
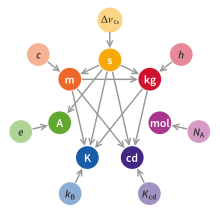
After the metre was redefined in 1960, the International Prototype of the Kilogram (IPK) was the only physical artefact upon which base units (directly the kilogram and indirectly the ampere, mole and candela) depended for their definition, making these units subject to periodic comparisons of national standard kilograms with the IPK. During the 2nd and 3rd Periodic Verification of National Prototypes of the Kilogram, a significant divergence had occurred between the mass of the IPK and all of its official copies stored around the world: the copies had all noticeably increased in mass with respect to the IPK. During extraordinary verifications carried out in 2014 preparatory to redefinition of metric standards, continuing divergence was not confirmed. Nonetheless, the residual and irreducible instability of a physical IPK undermined the reliability of the entire metric system to precision measurement from small (atomic) to large (astrophysical) scales. By avoiding the use of an artefact to define units, all issues with the loss, damage, and change of the artefact are avoided.
A proposal was made that:
- In addition to the speed of light, four constants of nature – the Planck constant, an elementary charge, the Boltzmann constant, and the Avogadro constant – be defined to have exact values
- The International Prototype of the Kilogram be retired
- The current definitions of the kilogram, ampere, kelvin, and mole be revised
- The wording of base unit definitions should change emphasis from explicit unit to explicit constant definitions.
The new definitions were adopted at the 26th CGPM on 16 November 2018, and came into effect on 20 May 2019. The change was adopted by the European Union through Directive (EU) 2019/1258.
Prior to its redefinition in 2019, the SI was defined through the seven base units from which the derived units were constructed as products of powers of the base units. After the redefinition, the SI is defined by fixing the numerical values of seven defining constants. This has the effect that the distinction between the base units and derived units is, in principle, not needed, since all units, base as well as derived, may be constructed directly from the defining constants. Nevertheless, the distinction is retained because "it is useful and historically well established", and also because the ISO/IEC 80000 series of standards, which define the International System of Quantities (ISQ), specifies base and derived quantities that necessarily have the corresponding SI units.
Related units
Non-SI units accepted for use with SI
Main article: Non-SI units mentioned in the SI
Many non-SI units continue to be used in the scientific, technical, and commercial literature. Some units are deeply embedded in history and culture, and their use has not been entirely replaced by their SI alternatives. The CIPM recognised and acknowledged such traditions by compiling a list of non-SI units accepted for use with SI, including the hour, minute, degree of angle, litre, and decibel.
Metric units not recognised by SI
Main article: List of metric unitsAlthough the term metric system is often used as an informal alternative name for the International System of Units, other metric systems exist, some of which were in widespread use in the past or are even still used in particular areas. There are also individual metric units such as the sverdrup and the darcy that exist outside of any system of units. Most of the units of the other metric systems are not recognised by the SI.
Unacceptable uses
Sometimes, SI unit name variations are introduced, mixing information about the corresponding physical quantity or the conditions of its measurement; however, this practice is unacceptable with the SI. "Unacceptability of mixing information with units: When one gives the value of a quantity, any information concerning the quantity or its conditions of measurement must be presented in such a way as not to be associated with the unit." Instances include: "watt-peak" and "watt RMS"; "geopotential metre" and "vertical metre"; "standard cubic metre"; "atomic second", "ephemeris second", and "sidereal second".
See also
- Conversion of units – Comparison of various scales
- List of international common standards
- Metrication
- Outline of the metric system – Overview of and topical guide to the metric system
Organisations
- International Bureau of Weights and Measures – Intergovernmental measurement science and measurement standards setting organisation
- Institute for Reference Materials and Measurements – research organization for a common European measurement systemPages displaying wikidata descriptions as a fallback (EU)
- National Institute of Standards and Technology – Measurement standards laboratory in the United States (US)
Standards and conventions
- Conventional electrical unit – Historical high-precision units of measurement
- Coordinated Universal Time (UTC) – Primary time standard
- Unified Code for Units of Measure – System of codes for unambiguously representing measurement units
Notes
- Ohm's law: 1 Ω = 1 V/A from the relationship E = I × R, where E is electromotive force or voltage (unit: volt), I is current (unit: ampere), and R is resistance (unit: ohm).
- From French: Conférence générale des poids et mesures.
- from French: Comité international des poids et mesures
- from French: Bureau international des poids et mesures
- Argentina, Austria-Hungary, Belgium, Brazil, Denmark, France, German Empire, Italy, Peru, Portugal, Russia, Spain, Sweden and Norway, Switzerland, Ottoman Empire, United States, and Venezuela.
- Attribution
![]() This article incorporates text from this source, which is available under the CC BY 3.0 license.
This article incorporates text from this source, which is available under the CC BY 3.0 license.
References
- ^ International Bureau of Weights and Measures (December 2022), The International System of Units (SI) (PDF), vol. 2 (9th ed.), ISBN 978-92-822-2272-0, archived from the original on 18 October 2021
- Materese, Robin (16 November 2018). "Historic Vote Ties Kilogram and Other Units to Natural Constants". NIST. Retrieved 16 November 2018.
- ISO 80000-1:2009 Quantities and units – Part 1: General.
- ^ David B. Newell; Eite Tiesinga, eds. (2019). The International System of Units (SI) (PDF) (NIST Special publication 330, 2019 ed.). Gaithersburg, MD: NIST. Retrieved 30 November 2019.
- ^ International Bureau of Weights and Measures (2006), The International System of Units (SI) (PDF) (8th ed.), ISBN 92-822-2213-6, archived (PDF) from the original on 4 June 2021, retrieved 16 December 2021
- "Units & Symbols for Electrical & Electronic Engineers". Institution of Engineering and Technology. 1996. pp. 8–11. Archived from the original on 28 June 2013. Retrieved 19 August 2013.
- Lawler, Daniel (18 November 2022). "Earth now weighs six ronnagrams: New metric prefixes voted in". phys.org.
- "List of Resolutions for the 27th meeting of the General Conference on Weights and Measures" (PDF). BIPM. 18 November 2022. Archived from the original (PDF) on 18 November 2022. Retrieved 18 November 2022.
- "New prefixes for the SI adopted by the General Conference on Weights and Measures". BIPM. Retrieved 11 January 2023.
- "Decimal Nature of the Metric System". US Metric Association. 2015. Archived from the original on 15 April 2020. Retrieved 15 April 2020.
- ^ Thompson, Ambler; Taylor, Barry N. (March 2008). Guide for the Use of the International System of Units (SI) (Report). National Institute of Standards and Technology. §10.5.3. Retrieved 21 January 2022.
- ^ "On the extension of the range of SI prefixes". 18 November 2022. Retrieved 5 February 2023.
- "Metric (SI) Prefixes". NIST.
- "Interpretation of the International System of Units (the Metric System of Measurement) for the United States" (PDF). Federal Register. 73 (96): 28432–28433. 9 May 2008. FR Doc number E8-11058. Retrieved 28 October 2009.
- "Avogadro Project". National Physical Laboratory. Retrieved 19 August 2010.
- "NIST Mise en Pratique of the New Kilogram Definition". NIST. 2013. Archived from the original on 14 July 2017. Retrieved 9 May 2020.
- "Practical realizations of the definitions of some important units". BIPM. 2019. Archived from the original on 9 April 2020. Retrieved 11 April 2020.
- "International Committee for Weights and Measures – Proceedings of the 106th meeting" (PDF).
- "Recommendations of the Consultative Committee for Mass and Related Quantities to the International Committee for Weights and Measures" (PDF). 12th Meeting of the CCM. Sèvres: Bureau International des Poids et Mesures. 26 March 2010. Archived from the original (PDF) on 14 May 2013. Retrieved 27 June 2012.
- "Recommendations of the Consultative Committee for Amount of Substance – Metrology in Chemistry to the International Committee for Weights and Measures" (PDF). 16th Meeting of the CCQM. Sèvres: Bureau International des Poids et Mesures. 15–16 April 2010. Archived from the original (PDF) on 14 May 2013. Retrieved 27 June 2012.
- "Recommendations of the Consultative Committee for Thermometry to the International Committee for Weights and Measures" (PDF). 25th Meeting of the CCT. Sèvres: Bureau International des Poids et Mesures. 6–7 May 2010. Archived from the original (PDF) on 14 May 2013. Retrieved 27 June 2012.
- United States. National Bureau of Standards (1959). Research Highlights of the National Bureau of Standards. U.S. Department of Commerce, National Bureau of Standards. p. 13. Retrieved 31 July 2019.
- "1.16" (PDF). International vocabulary of metrology – Basic and general concepts and associated terms (VIM) (3rd ed.). International Bureau of Weights and Measures (BIPM): Joint Committee for Guides in Metrology. 2012. Retrieved 28 March 2015.
- S. V. Gupta, Units of Measurement: Past, Present and Future. International System of Units, p. 16, Springer, 2009. ISBN 3642007384.
- "ISO 80000-1:2022 Quantities and units Part 1: General".
- "Interpretation of the International System of Units (the Metric System of Measurement) for the United States". Federal Register. 73. National Institute of Standards and Technology: 28432. 16 May 2008. Archived from the original on 16 August 2017. Retrieved 6 December 2022.
- "VIM3: International Vocabulary of Metrology". BIPM. Archived from the original on 31 October 2020.
- "Amtliche Maßeinheiten in Europa 1842" [Official units of measure in Europe 1842]. spasslernen (in German). 1 May 2009. Archived from the original on 25 September 2012. Retrieved 26 March 2011. Text version of Malaisé's book: Malaisé, Ferdinand von (1842). Theoretisch-practischer Unterricht im Rechnen [Theoretical and practical instruction in arithmetic] (in German). München: Verlag des Verf. pp. 307–322. Retrieved 7 January 2013.
- ^ Page, Chester H.; Vigoureux, Paul, eds. (20 May 1975). The International Bureau of Weights and Measures 1875–1975: NBS Special Publication 420. Washington, D.C.: National Bureau of Standards. p. 12.
- Alder, Ken (2002). The Measure of all Things – The Seven-Year-Odyssey that Transformed the World. London: Abacus. ISBN 978-0-349-11507-8.
- ^ Giunta, Carmen J. (2023). A Brief History of the Metric System: From Revolutionary France to the Constant-Based SI. SpringerBriefs in Molecular Science. Cham: Springer International Publishing. Bibcode:2023bhms.book.....G. doi:10.1007/978-3-031-28436-6. ISBN 978-3-031-28435-9. S2CID 258172637.
- Quinn, Terry J. (2012). From artefacts to atoms: the BIPM and the search for ultimate measurement standards. New York Oxford: Oxford University Press. ISBN 978-0-19-530786-3.
- Fenna, Donald (2002). Weights, Measures and Units. Oxford University Press. International unit. ISBN 978-0-19-860522-5.
- Maxwell, J. C. (1873). A treatise on electricity and magnetism. Vol. 2. Oxford: Clarendon Press. pp. 242–245. Retrieved 12 May 2011.
- "Historical figures: Giovanni Giorgi". International Electrotechnical Commission. 2011. Archived from the original on 15 May 2011. Retrieved 5 April 2011.
- "BIPM – Resolution 6 of the 9th CGPM". Bipm.org. 1948. Retrieved 22 August 2017.
- "Resolution 7 of the 9th meeting of the CGPM (1948): Writing and printing of unit symbols and of numbers". International Bureau of Weights and Measures. Retrieved 6 November 2012.
- "Redefining the kilogram". UK National Physical Laboratory. Retrieved 30 November 2014.
- "A Turning Point for Humanity: Redefining the World's Measurement System". NIST. 12 May 2018. Retrieved 16 January 2024.
- "Appendix 1. Decisions of the CGPM and the CIPM" (PDF). BIPM. p. 188. Retrieved 27 April 2021.
- Wood, B. (3–4 November 2014). "Report on the Meeting of the CODATA Task Group on Fundamental Constants" (PDF). BIPM. p. 7.
Milton responded to a question about what would happen if ... the CIPM or the CGPM voted not to move forward with the redefinition of the SI. He responded that he felt that by that time the decision to move forward should be seen as a foregone conclusion.
- "Commission Directive (EU) 2019/1258 of 23 July 2019 amending, for the purpose of its adaptation to technical progress, the Annex to Council Directive 80/181/EEC as regards the definitions of SI base units". Eur-Lex. 23 July 2019. Retrieved 28 August 2019.
- Olthoff, Jim (2018). "For All Times, For All Peoples: How Replacing the Kilogram Empowers Industry". NIST. Archived from the original on 16 March 2020. Retrieved 14 April 2020.
... the International System of Units (SI), popularly known as the metric system.
Further reading
- International Union of Pure and Applied Chemistry (1993). Quantities, Units and Symbols in Physical Chemistry, 2nd edition, Oxford: Blackwell Science. ISBN 0-632-03583-8. Electronic version.
- Unit Systems in Electromagnetism Archived 30 October 2020 at the Wayback Machine
- MW Keller et al. (PDF) Metrology Triangle Using a Watt Balance, a Calculable Capacitor, and a Single-Electron Tunnelling Device
- "The Current SI Seen From the Perspective of the Proposed New SI" (PDF). Barry N. Taylor. Journal of Research of the National Institute of Standards and Technology, Vol. 116, No. 6, Pgs. 797–807, Nov–Dec 2011.
- B. N. Taylor, Ambler Thompson, International System of Units (SI), National Institute of Standards and Technology 2008 edition, ISBN 1437915582.
External links
| SI units | |
|---|---|
| Base units | |
| Derived units with special names | |
| Other accepted units | |
| See also | |
| Systems of measurement | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Current |
| ||||||||||||
| Background |
| ||||||||||||
| Historic |
| ||||||||||||
| Ancient | |||||||||||||
| List articles | |||||||||||||
| Other | |||||||||||||



 ,
,  ,
,  , etc.
, etc.










 means that
means that  is used to define
is used to define  .
.